Moment of Inertia of Semicircle: In rotational motion, the moment of inertia serves the same purpose as mass does in translational motion. In other words, the moment of inertia is a measurement of the body’s resistance to a change in rotational motion. For instance, if the body is at rest, the larger the moment of inertia of the body, the more difficult it is to rotate the body. Likewise, the greater the body’s moment of inertia, the more difficult it is to stop its rotational motion.
The moment of inertia of a semicircle has been commonly expressed as:
I = πr4 / 4
To obtain the value of the moment of inertia of semicircle, initially derive the results of the moment of inertia of a full circle and divide it by two for getting the desired result of that moment of inertia for a semicircle. To establish the moment of inertia of the semi-circle, we will add the x and y axes.
Because of complete symmetry and uniform area distribution, we know that the moment of inertia relative to the x-axis is equal to that of the y-axis for a full circle.
Therefore, Ix = Iy = ¼ πr4
Now, the M.O.I relative to the origin, Jo = Ix + Iy = ¼ πr4 + ¼ πr4 = ½ πr4
Then, we need to pull out the area of a circle which gives us:
Jo = ½ (πr2) R2
Likewise, for the semicircle, the x-axis moment of inertia equals the y-axis moment of inertia. Because the semi-circle rotating around an axis is symmetric in this case, we consider these values to be equal. As a result, M.O.I will be half the moment of inertia of a full circle. This now gives us:
Ix = Iy = ⅛ πr4 = ⅛ (Ao) R2 = ⅛ πr2) R2
In order to find the moment of inertia of the semicircle, we will take the sum of both the x and y-axis.
M.O.I relative to the origin, Jo = Ix + Iy = ⅛ πr4 + ⅛ πr4 = ¼ πr4
For deriving the moment of inertia of semicircle we define the coordinates using the polar system.
z = r sin θ
y = r cos θ
We need to determine the differential area by finding the area of the element. It is given as:
ABCD is a sector with area = (r⋅d θ) ⋅ dr = r ⋅ drd θ
Then, the centroid of this elemental area from x-axis = y sin θ
By using this we can find the first moment of inertia about the x-axis,
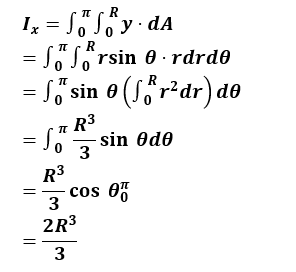
But, Ix = A = π / 2 R2 y
π / 2 R2 y = 2R3 / 3
y = 4R / 3π
For finding the second moment of inertia:
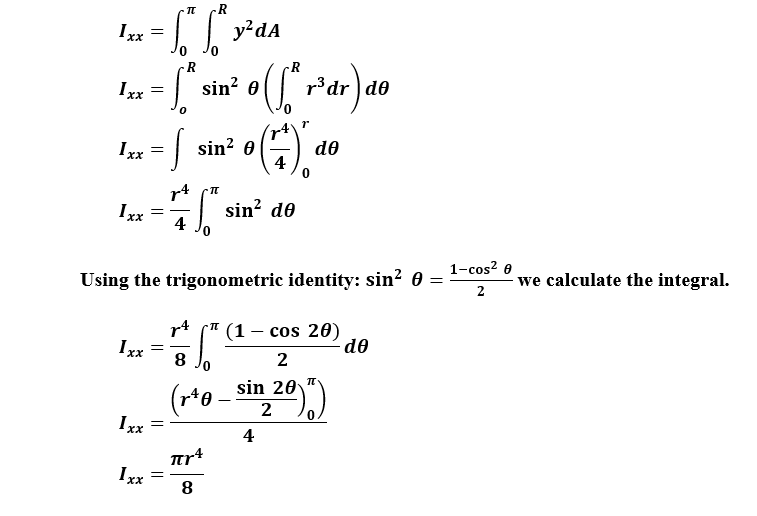
The moment of inertia has been primarily used to calculate the amount of force required to rotate an object. Such a value can be increased or decreased by increasing the radius from the axis of rotation; as the radius increases, the moment of inertia increases, decreasing the rotational speed.
The semicircle's moment of inertia is commonly expressed as follows: I = πr4 / 4
