Exterior Angles Theorem
In the previous segment, we learnt about Exterior angle theorem and its property. In this segment, we will solve an interesting problem based on exterior angles.
Exterior Angles – Example
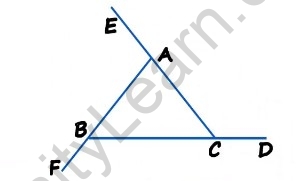
Solved Example:
Question: In the given figure, find the sum of the three exterior angles.
Solution:
The three exterior angles are ∠EAB, ∠ACD, and ∠FBC.
According to the exterior angle property of a triangle, the exterior angle is equal to the sum of the interior opposite angles.
∴ ∠EAB = ∠ACB + ∠ABC … (1)
∠ACD = ∠BAC + ∠ABC… (2)
∠FBC = ∠BAC + ∠ACB … (3)
Adding statements (1), (2), and (3),
∠EAB + ∠ACD + ∠FBC = ∠ACB + ∠ABC + ∠BAC + ∠ABC + ∠BAC + ∠ACB
∴ ∠EAB + ∠ACD + ∠FBC = 2 (∠ACB + ∠ABC + ∠BAC) … (4)
∠ACB, ∠ABC and ∠BAC are the interior angles of Δ ABC and the sum of the interior angles of a
triangle is 180°.
∴ ∠ACB + ∠ABC + ∠BAC = 180°
Substituting the above equation in equation (4),

