
Courses

By Shailendra Singh
|
Updated on 21 Nov 2024, 14:44 IST
Fundamental mode and harmonics: Vibration modes are particularly, but not exclusively, associated with musical instruments. It is a different type of vibration, and most musical instruments have more than one mode of vibration because, without it, their musical range would be severely limited. Contrast the sounds of a violin (which has 4 to 7 strings) with the sounds of a musical triangle, which only emits one note. Once you pluck a stretched string, you will always hear a distinct musical pitch. This pitch can be changed by adjusting the length, tension, or weight of the string, all of which are familiar to musicians. Strings and stretched drumheads have all been capable of producing a wide range of vibrations, allowing musical instruments with a diverse range of sounds to be created. When you use a brick or a frying pan instead, your musical range would be severely limited due to their limited vibration modes.
Whenever an object is forced into resonance vibrations at one of its natural frequencies, the object vibrates in such a way that a standing wave pattern forms within it. The vibrating medium, whether it is a guitar string, a Chladni plate, or the air column enclosed within a trombone, vibrates in such a way that a standing wave pattern results. Every natural frequency produced by an object or instrument has its own distinct vibrational mode or standing wave pattern. Such patterns are only created within the object or instrument at specific vibrational frequencies; these frequencies are known as harmonic frequencies, or simply harmonics. The resulting disturbance of the medium at any frequency other than a harmonic frequency is irregular and non-repeating. Harmonic frequencies are related to each other by simple whole-number ratios for musical instruments and other objects that vibrate in a regular and periodic fashion. It thus contributes to the pleasant sound of such instruments.
Translations (external), rotations (internal), and vibrations are the three general types of motions (internal). A diatomic molecule has a single motion, whereas polyatomic molecules have more complex vibrations called normal modes.
The fundamental model will be the one with the lowest frequency (f1). It is worth noting that the frequency of the nth mode is n times that of the fundamental. A harmonic is among a series of ascending sonic components that sound above the audible fundamental frequency. The sound’s harmonic spectrum is made up of higher frequency harmonics that sound above fundamental. Harmonics can be difficult to detect as single components, but they exist. One of the most fundamental descriptions of the vibration of a stretched string reveals a pattern in the set of resonance frequencies. When the lowest (or fundamental) frequency has been determined by adjusting the string’s weight, tension, and length, all subsequent frequencies are whole-number multiples: when the first frequency is f, the second is 2f, the third is 3f, and the fourth is nf. The frequencies have been known as natural frequencies or overtones, and the simple numerical pattern that connects them is known as a harmonic series: a stretched string has harmonic natural frequencies.
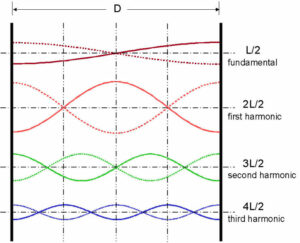
Harmonics often have a lower volume than fundamental modes. Harmonics are multiples of the fundamentals that are positive integers. For instance, if the fundamental frequency is 50 hertz (also known as the first harmonic), the second harmonic will undoubtedly be 100 Hz (50 * 2 = 100 Hz), and the third harmonic will be 150 Hz (50 * 3 = 150 Hz).
Whenever you pluck a string, you generally excite an infinite number of harmonic modes.
The Mathematician Method: “Sine Curve Initial Condition”

Only when you pull each mass element of a stretched string away from equilibrium (flat string), the string will form the shape of a sine curve and then let go, the entire string will vibrate in a single normal mode pattern. In case you begin with a different initial shape, one that is not sinusoidal, the string’s motion will be made up of different modes. Beginning with an exact sine-curve shape is difficult – some kind of fancy contraption is required.
The Musician Method: “Touch and Pluck”
It’s something that guitarists and violinists do on a regular basis. If they really want start making a “loop,” they gently touch the string at one point (where the node should be) and pluck the string at another (antinode). At this stage, a pulled loop’s oscillation (up and down motion) will “drive” the rest of the string to form additional equal-size loops that oscillate at the same frequency as the driving loop.
The Physicist Method: “Resonance”
Once the end of a string is gently shaken (vibrated) will a wave travel to the right (R), strike the fixed end, and reflect back to the left (L). When you shake at just the right “resonance” frequency, one that corresponds to one of the natural frequencies of the string, the two travelling waves (R and L) will combine to produce a large amplitude standing wave: R + L = STANDING WAVE.
Resonance

We will concentrate on this method because RESONANCE is one of the most important concepts in science. Dialling a radio, making music, shattering a crystal glass with your voice, imaging the body with an MRI machine, picking cherries, designing lasers, engineering bridges, skyscrapers, and machine parts, and other similar activities are all examples of resonance phenomena. Consider the act of pushing someone in a swing. If your hand’s frequency (periodic driving force) matches the natural frequency of the swing, the swing will oscillate with a large amplitude. It is a matter of timing rather than strength. A series of “gentle pushes” applied at precisely the “right time” in perfect rhythm with the swing will result in a dramatic increase in the swing’s amplitude. A small stimulus is amplified into a large one.
The smallest frequency at which an oscillation takes place, or the lowest component of a complex vibration. Resonances or harmonics are indeed multiples of the fundamental frequency found in a variety of pulsating variables. The fundamental mode of radial pulsation in stars like RR Lyrae and Cepheids, for example, is the simple periodic expansion and contraction of the star’s outer layers. Quite complex vibrational modes, known as the first overtone mode or harmonic, and so on, can exist.
The fundamental frequency is integrally multiplied by a harmonic. An overtone is said to be any frequency that is higher than the fundamental frequency. The fundamental frequency is the starting point for harmonics. Overtones begin counting after the fundamental frequency and work their way up to the harmonics.
It is the smallest frequency at which an oscillation takes place or the lowest component of a complex vibration.
The fundamental frequency is generally the lowest frequency generated by any given instrument. The fundamental frequency is also known as the instrument's first harmonic.