
Courses

By Shailendra Singh
|
Updated on 20 Nov 2024, 13:18 IST
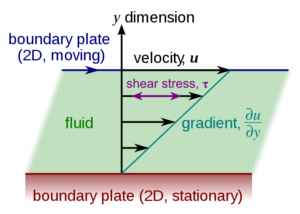
Dimensions of Velocity Gradient: The velocity gradient is a measure of how fluid velocity varies between different points within the fluid. The velocity difference between fluid layers adjacent to each other is called the velocity gradient. It is defined as v/x, where v is the velocity difference and x is the distance between layers.
The momentum transfer rate per unit area between two adjacent fluid layers is proportional to the negative value of the velocity gradient. The velocity gradient can also be described as the velocity rate per unit distance. Mathematically, it is defined as velocity gradient = velocity/distance.
It can also be defined as “the distance travelled by an object in a unit of time.”
The speed of an object is its position's rate of change relative to a frame of reference. A physical quantity through its basic unit with its dimensions is an expression of the dimensional formula of speed. The dimensional formula of speed is defined as an expression of a physical quantity through its basic unit with its own dimensions.
In hydrodynamics, the velocity gradient is defined as the change in velocity between adjacent fluid layers. This change can refer to differences in velocity between layers of flow in a pipe. It is often used to denote the gradient of the flow’s velocity with respect to its coordinates. It can also be defined as the derivative of the strain tensor with respect to time or the symmetric component of the gradient of flow velocity.
Gradients are described in the velocity space as strain rate tensors and vorticity sensors. The velocity gradient carries information about the velocity of the vector in the deformed configuration as an object deforms over time. The temperature gradient is expressed in degrees per unit length.
Viscosity is defined as the shear force per unit area required to produce a unit velocity gradient. Angular velocity is the change in angular displacement per unit of time.

Rigid rotation does not change the relative position of fluid elements, so the antisymmetric velocity gradient term does not affect the rate of strain change. Under turbulent conditions, the velocity profile is no longer parabolic. For turbulent flow, the average fluid velocity in a pipe is 0.82 times the velocity at the center. The third component of the velocity is assumed to be zero everywhere.
Gradients can be used to calculate velocity gradients and include them in stress calculations. For example, during a transient simulation with a time step of Delta t = 0.1, one can calculate the Euler quantity using the Lagrangian quantity.
Water behaves like a Newtonian fluid, meaning there is a linear relationship between shear stresses (internal forces acting on the water) and shear strain rate (velocity gradient). The curve representing this relationship passes through the origin.
All gradients, such as velocity gradients and potential gradients, have magnitude and direction. However, velocity gradients are scalars because they do not follow vector algebra.

1/sec= per second
The rate at which velocity changes as a function of distance from the stationary layer.