
Courses

By Shailendra Singh
|
Updated on 21 Nov 2024, 15:45 IST
In the field of measurements, the least count of a measuring instrument seems to be the smallest and most accurate value in the measured quantity that can be resolved on the scale of the instrument. In a real sense, it’s been connected to the precision of an instrument; an instrument that can measure smaller changes in a value relative to another instrument has a lower “least count” value and is thus more precise. Whatever measurement made by the instrument must be repeatable to the resolution of the least count. The precision of an instrument is inversely proportional to its least count.
A sundial, for example, may have only scale marks representing the hours of daylight; it would have a minimum count of one hour. A stopwatch being used time a race can resolve down to a hundredth of a second, which is its lowest count. Because it has more “counts” (scale intervals) in each hour of elapsed time, the stopwatch is more precise at measuring time intervals than the sundial. The least count of a component is indeed a critical tool for obtaining accurate readings from instruments such as the vernier calliper and screw gauge used in various experiments.
One of several sources of experimental error in measurements is least count uncertainty. The least count of a vernier calliper is about .02 mm, and the least count of a micrometre is .01 mm.
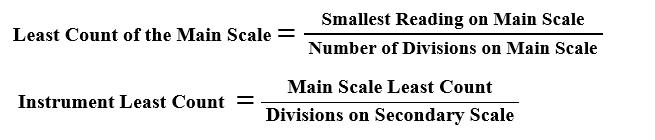
The Least Count of a measuring device has been calculated mathematically by dividing the main scale reading by the total number of divisions on the main scale. Also, check to see if the instrument has a secondary scale. And after that LC is the ratio of the main scale LC to the number of divisions on the secondary scale.
The least or minimum value that the measuring instrument can measure is referred to as its least count. Measurable values are really only valid up to this point. The least count errors are errors associated with the instrument’s resolution.
Graduations on a metre ruler may be at 1 mm division scale spacing or interval. A calliper’s Vernier scale may have a minimum count of 0.1 mm, whereas a micrometre’s minimum count may be 0.01 mm or 10 microns.
With both systematic and random errors, the least count error occurs. Higher precision components can reduce the least count error. The average value would be very close to the true value of the measurement if the observations were repeated and the arithmetic mean of the results was taken.

The least count is the smallest measurement that a device can accurately measure. In other words, it tells you how precise the measuring tool is. Every measuring instrument, like a ruler, a vernier caliper, or a stopwatch, has a least count.
Understanding the least count helps you:
For some instruments like vernier calipers or screw gauges, you calculate the least count using this formula:

The minimum possible measurable distance taken by a Vernier calliper is referred to as a Vernier reading.
The Least Count of a measuring device has been calculated mathematically by dividing the main scale reading by the total number of divisions on the main scale. Also, check to see if the instrument has a secondary scale. And after that LC is the ratio of the main scale LC to the number of divisions on the secondary scale.