
Courses

By Shailendra Singh
|
Updated on 20 Nov 2024, 12:19 IST
What is radius of gyration and how do you define it? Radius of gyration is the radial distance to a location with the same moment of inertia as the body’s actual distribution of mass if the complete mass of the body were concentrated. It is also known as the radius of a body about the axis of rotation. It is defined mathematically as the root mean square distance of an object’s pieces from its centre of mass or a specific axis, depending on the application. It’s the perpendicular distance between a large object and the rotational axis. The trajectory of a moving point can be illustrated using a body. We can also determine the typical distance that this point travels.
The radius of gyration is a measurement of a cross-elastic section’s stability against buckling. In actuality, depending on which axis is being addressed, there are various values for the radius of gyration for a cross-section. The radius of gyration is used to calculate the elastic buckling of columns (also known as the slenderness ratio). The direction in which the has to be regarded as significant and the buckling capacity is often determined by the lowest value. The root-mean-square distance of all electrons from their centre of gravity is the radius of gyration R of a particle. As a result, R may be determined quickly for simple geometrical bodies.
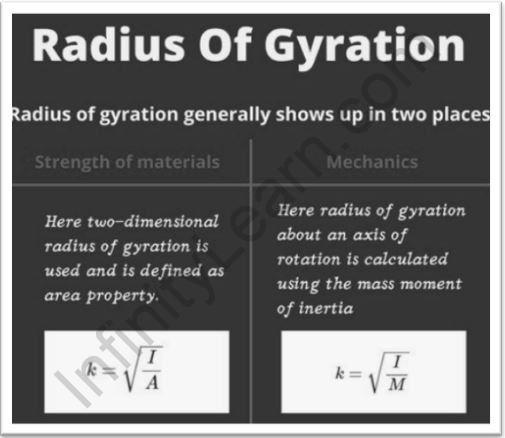
The formula of moment inertia in terms of the radius of gyration is given as follows:
I = mk2 (1)
where I = moment of inertia and m = mass of the body
Accordingly, the radius of gyration is as follows.
K = √I/m (2)
The radius of gyration is measured in millimeters. The moment of inertia of any complex body equation (1) can be easily determined by knowing the radius of the gyration.

Consider a body with n particles, each of which has a mass of m. r1, r2, r3…, rn are the perpendicular distances from the rotating axis. We know that the equation gives the moment of inertia regarding the gyration radius (1). We can calculate the body’s moment of inertia by substituting the values in the equation.
MK2 = M (r12+r22+r32+……+rn2) (3)
M = Total mass of the body
We can deduce from the preceding equation that the radius of gyration can alternatively be characterized as the root-mean-square distance between various body particles and the axis of rotation.
The gyration ratio and the slenderness ratio
The sq. base of the proportion of dormancy to the material region is utilized to work out the span of gyration. The not set in stone from where the cross-sectional region is intended to be accumulated at a point is meant by the worth got from it. This will help you accomplish a similar degree of idleness. It is determined by deciding the slimness of a segment’s cross-sectional region.

The two-dimensional gyro radius is used in structural engineering to describe the distribution of any cross-sectional area in the body mass around the centroidal axis. It is used in structural engineering to calculate the stiffness of a column. If the two-dimensional gyration principle moments are unequal, the column will buckle around the axis with the smaller principle moment. If we’re talking about engineering and we’re talking about continuous bodies of matter, It will be determined as integral.
The term radius of gyration describes the spatial dimensions of a polymer chain. It is possible to receive a polymer chain in a spatial dimension, which refers to a measure of spatial extent, such as height or length. In such instances, the radius of gyration might be used to solve the problems.
In terms of the moment of inertia around the axis and the total mass m, the radius of gyration around a particular axis can be determined.
Candidates must learn all of the topics covered in the JEE syllabus. Thoroughly, with a particular focus on physics. Experts say that questions on force, motion, sound, and so on are usually easy. A good mastery of NCERT texts on such topics is generally required to tackle questions in JEE. Portion A and part B of the JEE physics test are both required. The theoretical portion accounts for 80% of the grade, while the practical portion accounts for 20%. The force chapter accounted for 3.33 percent of total weightage in prior years.
The span of gyration is an estimation that is utilized to inspect how unique primary calculations would act when packed along with a pivot. It's utilized to expect to lock in pressure individuals and pillars.
The length, in inches, millimeters, or feet, is the SI unit for the sweep of gyration. It's the square base of idleness partitioned by the item's region.
The size and state of the body are two factors that influence the calculation of the radius of gyration. It is the positioning and organization of the rotational axis. It also hinges on mass appropriation regarding the body's rotational axis.