Table of Contents
Introduction
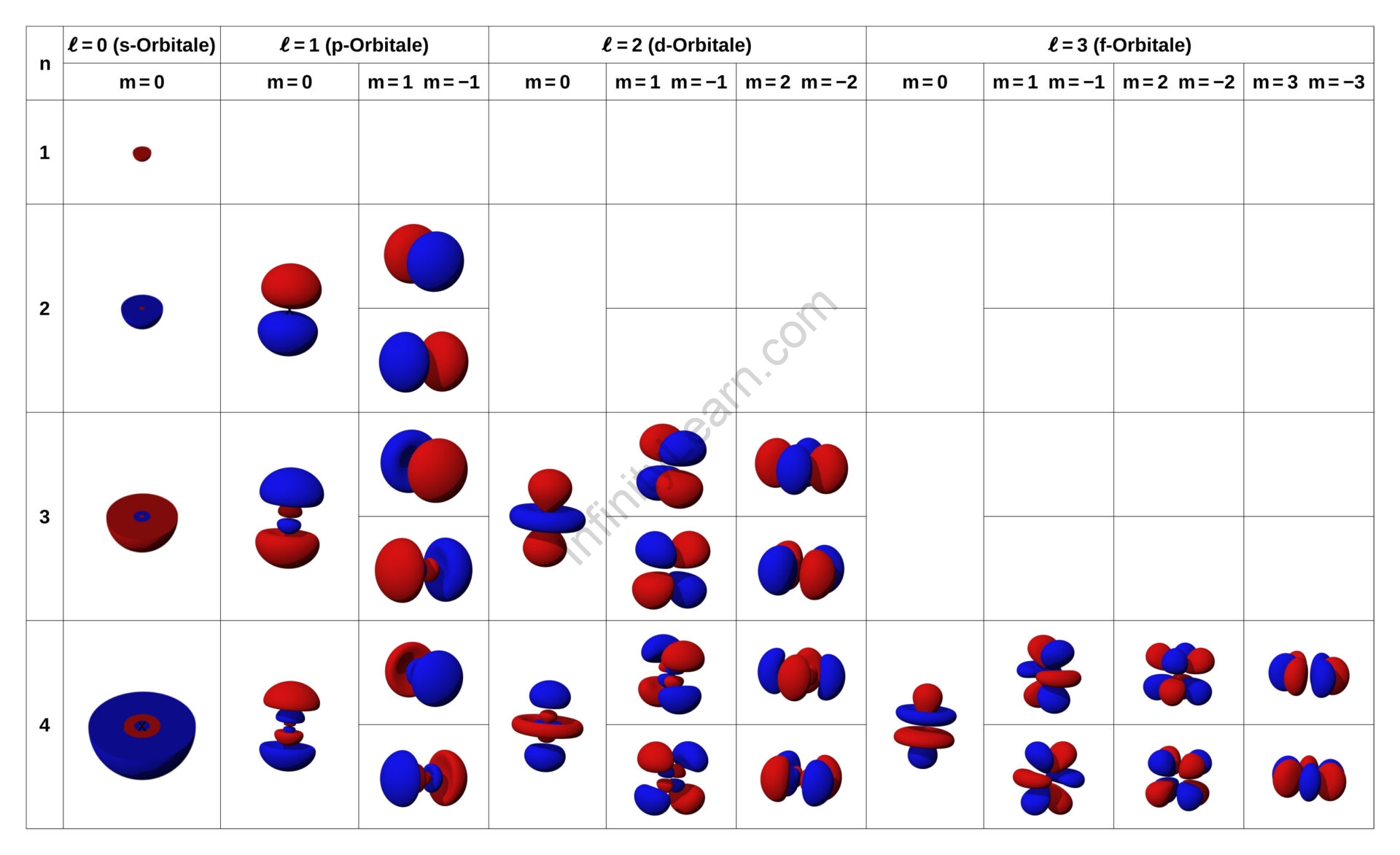
Quantum numbers are used in chemistry and quantum physics to explain the values of conserved quantities in the dynamics of a quantum system. Quantum numbers and their accompanying eigenspaces correspond to eigenvalues of operators that commute with the Hamiltonian—quantities that may be known with precision at the same time as the system’s energy. A specification of all of a quantum system’s quantum numbers combined fully characterizes the system’s underlying state and can, in theory, be measured jointly. Quantization of numerous observable quantities of interest is an important feature of quantum physics. Quantum numbers, in particular, take discrete sets of integers or half-integers as values, albeit they may approach infinity in some situations. This separates quantum mechanics from classical mechanics, in which the system’s properties such as mass, charge, and momentum all vary constantly. Quantum numbers are frequently used to represent the energy levels of electrons in atoms, but they can also refer to angular momentum, spin, and other concepts. Internal quantum numbers dictate the kind of a particle and its interactions with other particles through the fundamental forces, and flavor quantum numbers are one of the most important families.
Quantum numbers can be used to explain the path and mobility of an electron in an atom. When the quantum numbers of all the electrons in an atom are added together, the Schrodinger equation must be satisfied. Quantum numbers are the values of a quantum system’s preserved quantities. The Schrodinger wave equation for hydrogen atoms is solved using electronic quantum numbers (quantum numbers that describe electrons). Quantum numbers are used to define an electron’s trajectory and movement within an atom. Furthermore, the quantum numbers of each electron in an atom are added together; this should obey the Schrodinger equation. Notably, this is an important topic on your course syllabus. You must learn about this topic not only for your syllabus but also for future curriculum in various examinations. As a result, learn about the significance of quantum numbers in depth.
The four quantum numbers listed below can be used to fully characterize all of the properties of an atom’s electrons:
- The primary quantum number is n.
- The letter l represents the quantum number of orbital angular momentum (also known as the azimuthal quantum number).
- ml is an abbreviation for a magnetic quantum number.
- The electron spin quantum number is denoted by the symbol ms.
Overview
Quantum numbers, which are a collection of numbers, describe the position and energy of an electron in an atom. An atom is made up of a large number of orbitals that can be distinguished by their shape, size, and spatial orientation. The orbital properties are used to fully define an electron’s state and are expressed in three numbers:
- The primary quantum number
- Quantum azimuthal number
- Quantum number with magnetic properties
- Quantum number of spin
Quantum numbers are the numbers that designate and distinguish various atomic orbitals and electrons in an atom. Quantum Numbers are a set of four numbers that can be used to obtain information about all of the electrons in an atom, including their energy, location, space, orbital type, and even the direction of that orbital.
No two electrons in an atom may have the same set of quantum numbers, according to the Pauli exclusion principle. A half-integer or integer value is used to represent each quantum number. The primary quantum number, which is an integer, is the number of the electron’s shell. The worth is one or more (never 0 or negative). The angular momentum quantum number (s=0, p=1) represents the value of the electron’s orbital (s=0, p=1). l is greater than or equal to n-1 but less than or equal to zero.
Concept of quantum numbers
All electrons have four quantum numbers that describe their location in an atom’s electron cloud. The electron’s orbital size is described by the principle quantum number (n). The orbital shape is described by the angular momentum quantum number (l). The magnetic quantum number (ml) describes the orbital’s orientation in space, whereas the electron spin number (ms) describes how the electron spins on its own axis.
Principal quantum number
The symbol ‘n’ represents the principal quantum numbers. They denote the atom’s primary electron shell. A larger value of the principal quantum number implies a greater distance between the nucleus and the electrons because it describes the most likely distance between the nucleus and the electrons. Any integer with a positive value equal to or greater than one can be used as the principal quantum number’s value. The value n=1 denotes an atom’s innermost electron shell, which corresponds to the lowest energy state of an electron (or ground state). As a result, the principal quantum number, n, cannot be negative or equal to zero, because an atom cannot have a negative or zero value for a principal shell. When an electron is excited (infused with energy), it jumps from one principle shell to a higher shell, increasing the value of n. Similarly, as electrons lose energy, they return to lower shells, lowering n. Absorption is defined as an increase in the value of n for an electron that emphasizes the photons or energy absorbed by the electron. Similarly, a decrease in the value of n for an electron is referred to as emission, and it is at this point that the electrons emit their energy.
Azimuthal quantum number
The shape of an orbital is described by the azimuthal quantum number (or orbital angular momentum). Its value equals the total number of angular nodes in the orbital and is represented by the letter ‘l.’ A value of the azimuthal quantum number can represent an s, p, d, or f subshell, the shapes of which differ. The value of the azimuthal quantum number is determined (and limited by) the value of the principal quantum number, i.e. the azimuthal quantum number is a number that spans from 0 to 1. (n-1). For instance, if n = 3, the azimuthal quantum number can take one of three values: 0, 1, or 2. When l=1 and l=2, the resulting subshells are ‘p’ and ‘d,’ respectively (respectively). As a result, when n=3, the three possible subshells are 3s, 3p, and 3d. In a different case where n = 5, the possible values of l are 0, 1, 2, 3, and 4. When l = 3, the atom has three angular nodes.
The subshells that can be used for various ‘n’ and ‘l’ combinations are listed above. Because the value of ‘l’ is always less than the value of ‘n,’ the ‘2d’ orbital cannot exist.
Magnetic quantum number
Magnetic quantum numbers express the amount of energy in a subshell and estimate orbital angular momentum along a given axis. Furthermore, although the values associated with ml range from – to l, only integer steps are associated. In addition, the ‘s’ is a subshell with one orbital where l=0. As a result, the ml of an electron within an ‘s’ subshell is always equal to zero. Furthermore, the ‘p’ subshell has three orbitals, i.e. l=1. Three ‘dumbbell-shaped’ clouds are another name for it. As a result, in this ‘p’ subshell, an electron’s ml should be either -1, 0, or 1. Finally, when l=2, the ‘d’ subshell has five orbitals. Furthermore, the value of ml ranges from -2 to +2. Furthermore, the ml quantum number’s value here is related to orbital orientation.
The magnetic quantum number determines both the total number of orbitals and their orientation in a subshell. It is denoted by the symbol ml. This number represents the orbital’s angular momentum projected along a given axis. The azimuthal (or orbital angular momentum) quantum number determines the magnetic quantum number. The value of ml for a given l falls between -l and +l. As a result, it is affected by the value of n indirectly.
Also read:
FAQs
Who came up with the main quantum number?
The concept of energy levels and notation is based on the earlier Bohr model of the atom. Schrodinger's equation transformed the concept of a two-dimensional flat Bohr atom into a three-dimensional wave motion model. Where n = 1, 2, 3 is referred to as the main quantity, and h is the Planck constant.
What are the primary levels of energy?
The shell or orbital in which an electron resides relative to the nucleus of the atom is referred to as the electron's primary energy level in chemistry. This level is denoted by the principal quantum number n. The first element of the periodic table introduces a new key energy level.








