Table of Contents
A bend, produced by crossing a right roundabout cone with a plane is named as ‘conic’. It has recognized properties in Euclidean math. The vertex of the cone partitions it into two nappes alluded to as the upper nappe and the lower nappe.
Various kinds of conic segments are:
- Circle
- Ellipse
- Parabola
- Hyperbola
The back mirrors you find in your vehicle or the colossal round silver ones you experience at a metro station are instances of bends.
Bends have enormous applications all over the place, be it the investigation of planetary movement, the plan of telescopes, satellites, reflectors and so forth Conic comprise of bends which are gotten upon the crossing point of a plane with a twofold rested right roundabout cone.
Conic Section Formulas
Check the recipes for various kinds of areas of a cone in the table given here
| Circle | (x−a)^2+(y−b)^2=r2 | Center is (a,b)
Radius is r |
| Ellipse with the horizontal major axis | (x−a)^2/h^2+(y−b)^2/k^2=1 | Center is (a, b)
Length of the major axis is 2h. Length of the minor axis is 2k. Distance between the middle and either center is c with c^2=h^2−k^2, h>k>0 |
| Ellipse with the vertical major axis | (x−a)^2/k^2+(y−b)^2/h^2=1 | Center is (a, b)
Length of the major axis is 2h. Length of the minor axis is 2k. Distance between the middle and either center is c with c^2=h^2−k^2, h>k>0 |
| Hyperbola with the horizontal transverse axis | (x−a)^2/h^2−(y−b)^2/k^2=1 | Center is (a,b)
Distance between the vertices is 2h Distance between the foci is 2k. c^2=h^2 + k^2 |
| Hyperbola with the vertical transverse axis | (x−a)^2/k^2−(y−b)^2/h^2=1 | Center is (a,b)
Distance between the vertices is 2h Distance between the foci is 2k. c^2= h^2 + k^2 |
| Parabola with the horizontal axis | (y−b)^2=4p(x−a), p≠0 | Vertex is (a,b)
Focus is (a+p,b) Directrix is the line x=a−p Axis is the line y=b |
| Parabola with vertical axis | (x−a)^2=4p(y−b), p≠0 | Vertex is (a,b)
Focus is (a+p,b) Directrix is the line x=b−p Axis is the line x=a |
Concentration, Eccentricity and Directrix of Conic
A conic area can likewise be portrayed as the locus of a point P moving in the plane of a proper point F known as concentration (F) and a decent line d known as directrix (with the emphasis not on d) so that the proportion of the distance of guide P from center F toward its separation from d is a steady e known as unconventionality. Presently,
If capriciousness, e = 0, the conic is a circle
On the off chance that 0<e<1, the conic is an oval
In the event that e=1, the conic is a parabola
What’s more, if e>1, it is a hyperbola
Along these lines, erraticism is a proportion of the deviation of the oval from being round. Assume, the point framed between the outer layer of the cone and its pivot is β and the point shaped between the cutting plane and the hub is α, the unconventionality is;
e = cos α/cos β
Boundaries of Conic
Aside from concentration, flightiness and directrix, there are not many more boundaries characterized under conic areas.
- Head Axis: Line joining the two central focuses or foci of oval or hyperbola. Its midpoint is the focal point of the bend.
- Straight Eccentricity: Distance between the concentration and focus of a segment.
- Latus Rectum: A harmony of area corresponding to directrix, which goes through a concentration.
- Central Parameter: Distance from concentration to the comparing directrix.
- Significant hub: Chord joining the two vertices. It is the longest harmony of an oval.
- Minor hub: Shortest harmony of a circle.
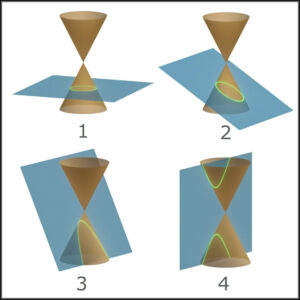
Conic Section Circle
On the off chance that β=90, the conic area framed is a circle as displayed beneath.
Conic Section Ellipse
On the off chance that α<β<90o, the conic area so framed is a circle as displayed in the figure underneath.
Conic Section Parabola
If α=β, the conic area framed is a parabola (addressed by the orange bend) as displayed beneath.
Conic Section Hyperbola
In the event that 0≤β<α, the plane meets both nappes and the conic area so shaped is known as a hyperbola
Conic Section Standard Forms
For circles and hyperbolas, the standard structure has the x-pivot as the key hub and the beginning (0,0) as the middle.
The vertices are (±a, 0) and the foci (±c, 0).
Characterize b by the situations c^2= a^2 − b^2 for a circle and c^2 = a^2 + b^2 for a hyperbola.
For a circle, c = 0 so a2 = b2. For the parabola, the standard structure has the attention on the x-hub at the point (a, 0) and the directrix is the line with condition x = −a. In standard structure, the parabola will constantly go through the beginning.
- Circle: x^2+y^2=a^2
- Circle: x^2/a^2 + y^2/b^2 = 1
- Hyperbola: x^2/a^2 – y^2/b^2 = 1
- Parabola: y^2=4ax when a>0
Conic Sections Equations
| Conic section Name | Equation when the centre is at the Origin, i.e. (0, 0) | Equation when centre is (h, k) |
| Circle | x^2 + y^2 = r^2; r is the radius | (x – h)^2 + (y – k)^2 = r^2; r is the radius |
| Ellipse | (x2/a2) + (y2/b2) = 1 | (x – h)^2/a^2 + (y – k)^2/b^2 = 1 |
| Hyperbola | (x2/a2) – (y2/b2) = 1 | (x – h)^2/a^2 – (y – k)^2/b^2 = 1 |
| Parabola | y^2 = 4ax, where a is the distance from the origin to the focus | |
FAQs
What are the 4 conic areas?
In view of the tendency of point between the plane and the cone, we can arrange the conic areas into 4 sorts. They are: Circles, Ovals, Parabolas, Hyperbolas
What is a conic area, all things considered?
We can notice conic areas in some genuine circumstances. For instance, when we consider the Sun as one concentration, then, at that point, the way of planets structure ovals around it. Illustrative mirrors help in social affair light bars at the focal point of the parabola.