Table of Contents
In analytic geometry, a hyperbola is formed by intersecting a right circular cone with a plane at an angle such that both halves of the cone are crossed. This intersection results in two unbounded curves that are mirror images of each other.
Hyperbola
In other terms, a hyperbola is the locus of all points in a plane where the absolute difference between two fixed points on the plane remains constant.
The fixed points are the foci (single focus). The eccentricity of a hyperbola is the constant, and the directrix is the fixed-line. Eccentricity, denoted by the letter e, is a feature of the hyperbola that shows its lengthening.
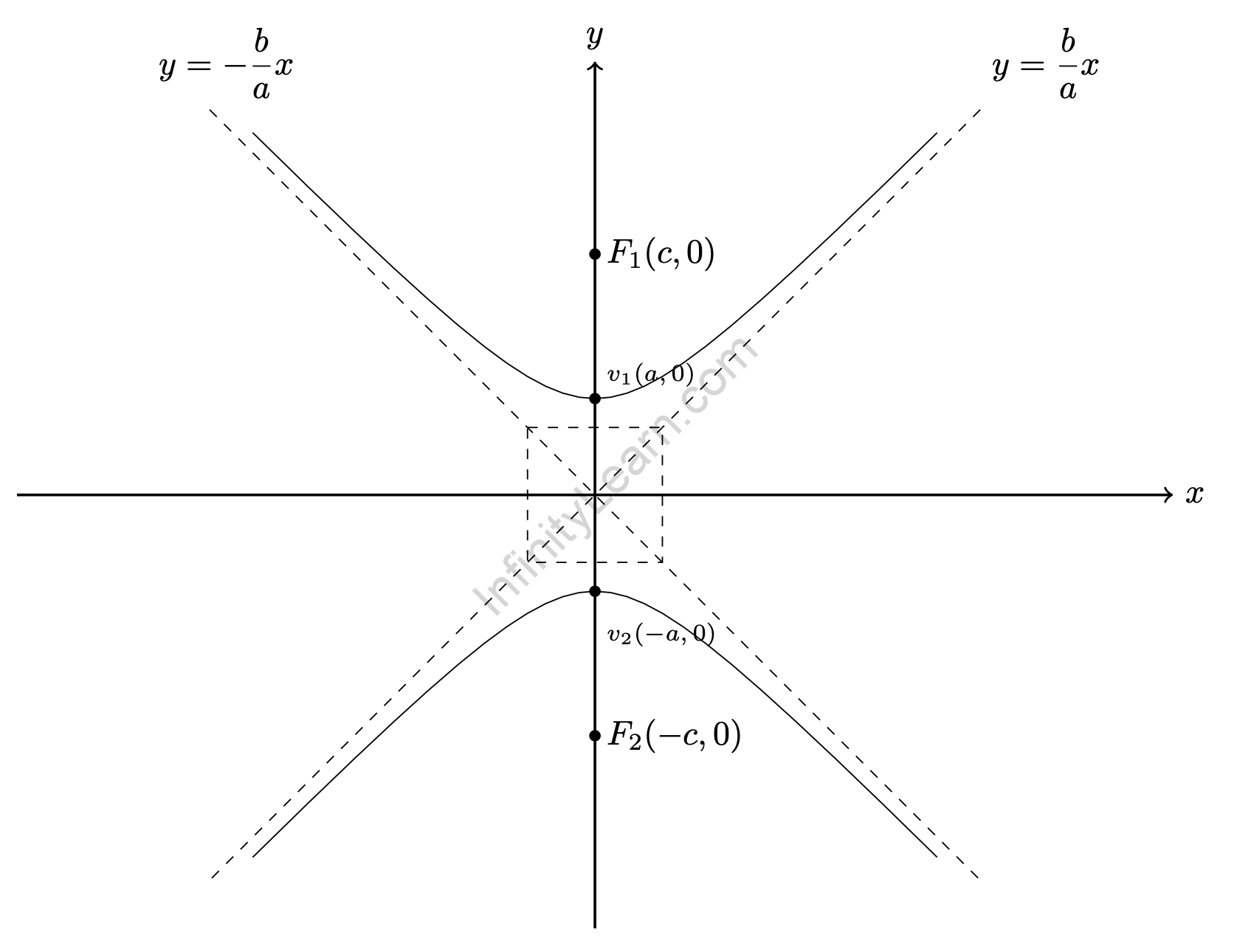
Hyperbola is defined by the following terms:
1. The Transverse Axis is a line that passes through the focus and is perpendicular to the directrix.
2. The vertices are the intersection points of the hyperbola’s primary axes.
3. The hyperbola’s Centre is the midpoint of its vertices.
4. The conjugate axis is a straight line that passes through the Centre of the hyperbola and is perpendicular to the transverse axis.
5. The Latus Rectum is a chord that runs through any of the two foci and is perpendicular to the transverse axis.
Orientation:
A vertical hyperbola is a shape that opens up and down. A horizontal hyperbola, also known as a sideways hyperbola, opens to the left and right. Each type of hyperbola has two symmetry axes.
What are Hyperbola’s Characteristics?
The following are some of the most important properties of a hyperbola:
1. Every hyperbola has two foci or points of emphasis. At each point on the hyperbola, the difference in distances between the two foci is a constant.
2. The directrix is a straight line that connects both of the hyperbola’s foci and runs parallel to the hyperbola’s conjugate axis.
3. The directrix is always perpendicular to the transverse axis.
4. The transverse axis runs across the foci and vertices.
5. The tangent line of a hyperbola is always parallel to the directrix at its vertices.
6. For the hyperbola x²⁄a² – y²⁄b²= 1, the length of the latus rectum is 2b²⁄a.
7. If the transverse and longitudinal lengths are equal, a hyperbola is said to be rectangular or equilateral.
8. The difference in focal distances between any two points on the hyperbola is constant and equal to the transverse axis, i.e. ||PS – PS’|| = 2a. The focal length is measured by the distance SS’.
A Hyperbola’s Components:
- Let’s go through a few keywords related to the different parameters of a hyperbola.
- Hyperbola foci: The hyperbola has two foci, with coordinates F(c, o) and F’ (-c, 0).
- Center of Hyperbola: The Centre of the hyperbola is the middle of the line connecting the two foci.
- Major Axis: The hyperbola’s major axis measures is 2a units in length.
- Minor Axis: The hyperbola’s minor axis measures 2b units in length.
- Vertices: The vertices are the spots on the hyperbola where it intersects the axis. The hyperbola’s vertices are (a, 0), (b, 0), and (c, 0). (-a, 0).
- Latus Rectum: The latus rectum is a line drawn perpendicular to the hyperbola’s transverse axis and going through the hyperbola’s foci. 2b²/a is the length of the hyperbola’s latus rectum.
- Transverse Axis: The transverse axis of the hyperbola is the line that passes through the two foci and the Centre of the hyperbola.
- Conjugate Axis: The conjugate axis of the hyperbola is a line that passes through the Centre of the hyperbola and is perpendicular to the transverse axis.
- Hyperbola Eccentricity: (e > 1) The eccentricity is the ratio of the focus’ distance from the hyperbola’s Centre to the vertex’s distance from the hyperbola’s Centre. Because the focus is ‘c’ units away from the vertex and the vertex is ‘a’ units away, the eccentricity is e = c/a.
Hyperbola Standard Equation:
There are two standard Hyperbola equations.
The transverse and conjugate axes of each hyperbola are used to derive these equations. The typical hyperbola equation is x²⁄ a² – y²⁄ b² = 1, with the transverse axis as the x-axis and the conjugate axis as the y-axis.
Furthermore, another classic hyperbola equation is y²⁄ a² – x²⁄ b² = 1, with the transverse axis as the y-axis and the conjugate axis as the x-axis. The two conventional forms of hyperbola equations are shown in the graphic below.
Also read: Important Parabola Formulas for JEE
FAQs
What is the difference between the focus and Centre of a hyperbola?
Focus - The two fixed points that constitute the hyperbola are referred to as the focus and Foci, respectively. The middle of the line segment that connects the two foci is called the Centre. Or the place where the transverse and conjugate axes connect.
What are the hyperbola's asymptotes?
The asymptotes of the hyperbola are the tangents to the Centre. The line perpendicular to the transverse axis and passing through any of the foci parallel to the conjugate axis is known as the hyperbola's latus rectum. 2b²/a is the answer.








