Table of Contents
Introduction:
That equation which is in the form of sine, cosine, tangent, secant, cosecant, cotangent is termed trigonometric equation. Solving a trigonometric equation is the process of finding the value of the angles, which satisfies the given equation. Let we will discuss its existence in two cases also discuss some examples.
Trigonometry roots special case:
That equation which is in the form of sine, cosine, tangent, secant, cosecant, cotangent is termed as a trigonometric equation. Solving a trigonometric equation is the process of finding the value of the angles, which satisfies the given equation. There exist two cases while solving a trigonometric equation namely:
- When the interval is given: The angle satisfying the equation in the given interval is to be found.
- When no interval is given: The general solution is to be calculated.
Periodic nature indicates that there exist many values, which satisfy the given equation.
The concept of root loss in a trigonometric equation refers to the missing of roots during the application of algebraic operations. It usually occurs while similar terms are cancelled out on both sides. It is explained using the following example.
Let sin θ [A] = sin θ [B]. Here, A and B can be termed as trigonometric functions. Upon cancelling sin θ, the solutions are lost. sin θ = 0 is obtained.
i.e. θ = n π, where n = 0, ±1, ±2, ………
Thus, the cancellation can’t be done directly.
Extraneous roots are the ones that are obtained while the terms in trigonometric equations are squared. The check for extraneous roots must be done in order to obtain the solution accurately.
The steps to solve trigonometric equations are as follows:
- The given equation is taken in the form of a function of an angle.
- The equation, written as a trigonometric function of an angle is constant.
- The possible angles for the angle are listed.
- The variable is solved
- The restriction is applied to the solution.
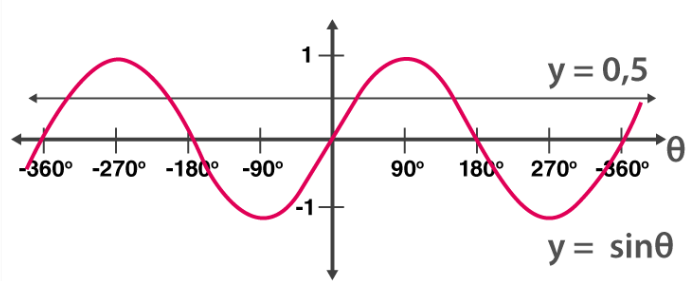
Some examples of special Cases For Trigonometric Roots:
Example 2: The general solution of sin x − 3 sin 2x + sin 3x = cos x − 3 cos 2x + cos 3x is __________.
Solution:
sin x − 3 sin 2x + sin 3x = cos x − 3 cos 2x + cos 3x
⇒ 2 sin2x cosx − 3 sin2x − 2 cos2x cosx + 3 cos2x = 0
⇒ sin2x (2cosx − 3) − cos2x (2cosx − 3) = 0
⇒ (sin2x − cos2x) (2cosx − 3) = 0
⇒ sin2x = cos2x
⇒ 2x = 2nπ ± (π / 2 − 2x)
i.e., x = [nπ / 2] + [π / 8]
Example 3: Find the solution to the following equation such that 0 ≤ x < 2π, 2sin2 (x) − sin (x) + 3 = 4
Solution:
2 sin2(x) − sinx − 1 = 0
Next, we factor.
(2 sin (x) + 1) (sin (x) − 1) = 0
Set each factor equal to zero and solve.
2 sin (x) + 1 = 0 or sin (x) − 1 = 0
sin (x) = −1 / 2 sin (x) = 1
Determine the angles that satisfy each solution within one revolution.
The angles 7π / 6 and 11π / 6 satisfy the first, and π / 2 satisfies the second.
Example 4: Solve the following equation for 0 ≤ x < 2π, sin 2x = cos 2x
Solution:
Let u = 2x.
The equation now becomes:
sin u = cos u
The sine and cosine functions are equal at the following angles.
u = π / 4; 5π / 4; 9π / 4; 13π / 4
These angles are divided by 2 so that it is within the range.
2x = π / 4; 2x = 5π / 4; 2x = 9π / 4; 2x = 13π / 4
Dividing each answer by 2 gives
x = π / 8; 5π / 8; 9π / 8; 13π / 8
Example 5: Solve the equation for 0 ≤ x < 2π, 3 tan2 x = 1
Solution:
3 tan2 x = 1;
Divide both sides by 3
tan2 x = 1 / 3
Take the square root on both sides.
tan x = ± 1 / √3
This can be written as two separate equations
tan x = 1 / √3 and tan x = −1 / √3
Take the inverse tangent
x = π / 6; 7π / 6 and x = 5π/ 6; 11π / 6
Example 6: If cos θ + cos 7θ + cos 3θ +cos 5θ = 0, then what is the value of θ?
Solution:
Combining θ and 7θ, 3θ and 5θ, we get
2 cos 4θ (cos 3θ + cos θ) = 0
4 cos 4θ cos 2θ cosθ = 0
4 [(1 / 23) sinθ] (sin23 θ) = 0; sin 8θ = 0.
Hence, θ = nπ / 8
Also read: Inverse Trigonometric Functions in Maths
FAQs
How many trigonometry functions?
In mathematics 6 are the important trigonometry function. those are sine, cosine, tangent, cosecant, secant, cotangent.
How trigonometry function is helpful?
Trigonometry functions are very useful for studying the triangle. It's used in oceanography in calculating the height of tides in oceans.
Q. What is trigonometry special case?
Ans: If any trigonometry equation does not have any exact roots. the angle depends on two cases such as
- interval is given then angle satisfying the equation in the given interval
- interval is not given then the general solution can be calculated for any angle.






