Table of Contents
Introduction
Trigonometry is a branch of mathematics that studies triangles and the relationships between their sides and angles. Trigonometry can be used to solve problems in physics, engineering, and other fields.
The word “trigonometry” is derived from the Greek words “tri” meaning “three” and “gonia” meaning “angle.” Thus, trigonometry literally means “the study of three angles.”
The first recorded use of trigonometry was by the Greek mathematician Hipparchus in the 2nd century BC. Hipparchus developed a system for measuring angles and determining the lengths of sides of triangles.
The most important contribution made by Hipparchus was the discovery of a relationship between the angles of a triangle and the lengths of its sides. This relationship is known as the “sine law” and is one of the most fundamental concepts in trigonometry.
The sine law states that the sine of an angle is equal to the length of the opposite side divided by the length of the hypotenuse. This relationship can be used to solve problems involving triangles.
Trigonometry was further developed by the Islamic mathematician Al-Khwarizmi in the 9th century. Al-Khwarizmi developed methods for solving problems involving right triangles.
The modern form of trigonometry was developed by the English mathematician Isaac Newton in the 17th century. Newton developed a system for representing angles in terms of radians instead of degrees.
Trigonometry is used in a variety of fields, including physics, engineering, and navigation. It can be used to solve problems involving triangles, such as calculating the height of a building or the distance between two points.
Trigonometry is also used in the study of waves and vibrations, and in the design of bridges and other structures. It is an essential tool for anyone working in the field of engineering.
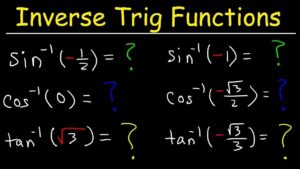
Inverse trigonometric functions
Inverse trigonometric functions are functions that allow us to solve problems involving angles in radians. There are three inverse trigonometric functions: arcsin, arccos, and arctan.
The arcsin function takes an angle in radians and returns the sine of that angle. The arccos function takes an angle in radians and returns the cosine of that angle. The arctan function takes an angle in radians and returns the tangent of that angle.
Each of these inverse trigonometric functions can be used to solve problems in which we are given an angle in radians and we need to find the other trigonometric function. For example, if we are given an angle in radians and we need to find the cosine of that angle, we can use the arccos function to do so.
The inverse trigonometric functions can also be used to find angles in radians when we are given the other trigonometric function. For example, if we are given the cosine of an angle in radians, we can use the arccos function to find the angle.
Graphs of Inverse Trigonometric Functions
For each trigonometry ratio, there are six inverse trig functions in particular. Six significant trigonometric functions have inverses:
- Arcsine
- Arccosine
- Arctangent
- Arccotangent
- Arcsecant
- Arccosecant
Derivatives of Inverse Trigonometric Functions
Inverse trigonometric functions’ derivatives are first-order derivatives. Let’s look at the derivatives of all six inverse functions in this section.
| Inverse Trig Function | dy/dx |
| y = sin-1(x) | 1/√(1-x2) |
| y = cos-1(x) | -1/√(1-x2) |
| y = tan-1(x) | 1/(1+x2) |
| y = cot-1(x) | -1/(1+x2) |
| y = sec-1(x) | 1/[|x|√(x2-1)] |
| y = csc-1(x) | -1/[|x|√(x2-1)] |
Inverse Trigonometric Functions Table
Let’s rebuild all of the inverse trigonometric functions in this section, including their notation, definition, domain, and range.
| Function Name | Notation | Definition | Domain of x | Range |
| Arcsine or inverse sine | y = sin-1(x) | x=sin y | −1 ≤ x ≤ 1 |
|
| Arccosine or inverse cosine | y=cos-1(x) | x=cos y | −1 ≤ x ≤ 1 |
|
| Arctangent or
Inverse tangent |
y=tan-1(x) | x=tan y | For all real numbers |
|
| Arccotangent or
Inverse Cot |
y=cot-1(x) | x=cot y | For all real numbers |
|
| Arcsecant or
Inverse Secant |
y = sec-1(x) | x=sec y | x ≤ −1 or 1 ≤ x |
|
| Arccosecant | y=csc-1(x) | x=csc y | x ≤ −1 or 1 ≤ x |
|
FAQ’S
Q. What are the names of the six inverse trig functions?
Ans 1. Sine, cosine, tangent, secant, cosecant, and cotangent are the six fundamental trigonometric functions. Inverse sine, inverse cosine, inverse tangent, inverse secant, inverse cosecant, and inverse cotangent are the inverse trigonometric functions of these.
Q. Which of the three fundamental inverse trigonometric functions are you familiar with?
Ans 2. When two side lengths are known, the inverse trigonometric functions sin1(x), cos1(x), and tan1(x) are used to obtain the unknown measure of an angle in a right triangle.
Q. What is the procedure for determining the inverse of a trig function?
Ans. Solve for the statement “x is equal to the angle whose sine is 1/2” to obtain the inverse of an equation like sin x = 1/2. This sentence is written as x = sin–1(1/2) in trig. A –1 is placed in the superscript position in this notation.