Table of Contents
One of the key concepts in the derivative application is monotonicity. The monotonicity of a function provides information about the function’s behaviour. A function is said to be monotonically increasing if its graph only grows with increasing equation values. Similarly, if the values of a function are only decreasing, it is monotonically decreasing. The sign of a function’s derivative determines its nature. A monotonic function is one in which the graph of a function is in either the upward or downward direction. If the function’s graph is pointing upward, it has increasing values and is said to be monotonically increasing. Similarly, if the graph of a function is pointing down, it has decreasing values and is said to be monotonically decreasing.
Extremum is pointed in a function’s domain where the graph changes direction from upwards to downwards or downwards to upwards. The derivative of a function, if it exists at such points, is always zero.
Monotonicity of a Function
If a function increases or decreases across its entire domain, it is said to be monotonic.
Increasing function:
If x1 < x2and F(x1) < F(x2) the function is known as an increasing function or a strictly increasing function.
Decreasing function:
For F(x) = e(-x)
If x1 > x2 and F(x1) > F(x2), then the function is known as a decreasing function or a strictly decreasing function.
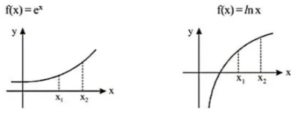
Increasing and Decreasing Function
(a). For a monotonically increasing function y = f(x), dy/dx ≥ 0 for all such values of interval (a,b), and equality may hold for discrete values.
(b). For a monotonically decreasing function y = f(x), dy/dx ≤ 0 for all such values of interval (a,b), and equality may hold for discrete values.
Maxima and Minima
The point on the curve where the function value is greater than the limiting function value is defined as the local maxima.
The point on the curve where the function value is less than the limiting function value is defined as the local minima.
Global Maxima: the highest value of the function among its various critical points.
Global Minima: is the function’s minimum value among its various critical points.
Extremum of functions
The extremum of a function is the function’s smallest and greatest value. There are three distinct cases for all such values, which are as follows:
Case 1: If a function y = f(x) is the strictly increasing function in an interval [a,b], then f(a) is the smallest value and f(b) is the greatest value.
Case 2: If the strictly decreasing function y = f(x) in an interval [a,b] is f(a), then f(a) is the greatest value and f(b) is the lowest value.
Case 3: If a function y = f(x) is non-monotonic in the interval [a,b] and continuous, the greatest and least value of the function are at the points where dy/dx = 0 or does not exist, or at extreme values, i.e. at x = a and x = b.
FAQs
Q. What does the term “function monotonicity” mean?
Ans: A monotonic function is one that is either entirely nonincreasing or entirely nondecreasing. A function is monotonic if the sign of its first derivative (which does not have to be continuous) does not change.
Q. How do you demonstrate that a function is monotonic or decreasing?
Ans: The following are the results of the monotonic function test: Assume that a function is continuous on [a, b] and differentiable on (a, b). If the derivative for all x in (a, b) is greater than zero, the function is increasing on [a, b]. If the derivative for all x in (a, b) is less than zero, the function is decreasing on [a, b].








