Table of Contents
The inverses of exponential functions were indeed logarithmic functions. x = ay would be the inverse of the exponential function y = ax. The logarithmic function y = logax has been defined as being equivalent to the exponential equation x = ay when the following conditions are met:
x = ay, a > 0, and a≠1. The logarithmic function with base a is how it should be called.
Graphs of the form y = logax when a > 1 and when a = 0 are being shown below. It’s worth noting that the domain only contains positive real numbers, and that the function always increases as x increases.
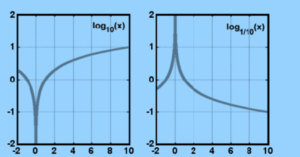
A logarithmic function does have a domain of real numbers greater than zero and a range of real numbers. With respect to the line y = x, the graph of y = logax is symmetrical to the graph of y = ax. This holds true for any function and its inverse.
Here are some useful logarithmic properties that arise from identities involving exponents and the definition of the logarithm. Keep in mind that a > 0 and x > 0.
loga1 = 0.
logaa = 1.
loga(ax) = x.
alogax = x.
loga(bc) = logab + logac.
loga(b/c) = logab – logac.
loga(xd) = d logax
A natural logarithmic function would be one with base e. f (x) = logex = ln x, where x > 0. ln x seems to be actually a new notation for logarithms with base e. Many calculators have “log” and “ln” buttons. The “log” button presumes the base is ten, while the “ln” button lets the base equal e. The base-10 logarithmic function can be described as the common logarithmic function. Because our numbering system is based on ten, it is widely used. Natural logarithms appear more frequently in calculus.
Applications of Logarithms
Prior to the invention of the modern calculator, John Napier invented logarithms as a method of performing lengthy arithmetic calculations.
Logarithms have recently become popular for simplifying complex calculations involving high-level exponents. In science, for instance, pH and pKa are used to simplify high exponential concentrations and dissociation constants. The goal is to condense a wide range of values into a more manageable scope. A dissociation constant can be less than 1010 or greater than 10-50. Having taken the logarithm of each value gives the values a more comprehensible context (10 to -50).
FAQs
What is the difference between exponential and logarithmic functions?
The exponential function can be represented by ƒ(x) = ex, while the logarithmic function is represented by g(x) = ln x, with the former being the inverse of the latter. The exponential function's domain is indeed a set of real numbers, whereas the logarithmic function's domain is a set of positive real numbers.
What is exponential and logarithmic functions used for?
Interest earned on an investment, population growth, and carbon dating seems to be three of the most common applications of exponential and logarithmic functions.
How do you solve logarithmic and exponential functions?
For solving an exponential equation, first isolate the exponential expression, then logarithm on both sides of the equation, and solve for the variable. For solving a logarithmic equation, isolate the logarithmic expression first, then exponentiate both sides of the equation and solve for the variable.




