Table of Contents
The primary objective of a collision experiment is to determine how particles interact during a collision by measuring particle masses and velocities before and after the collision. We do not seem to have direct access to what the particles do during the collision in the case of microscopic objects because the lengths and times involved are very small for observation, so there is no more direct way to obtain this information. Throughout this experiment, you would then investigate several simple collisions between macroscopic objects in order to gain a better understanding of how these experiments work and the effects of different conservation laws.
In several cases, conservation laws limit but do not completely determine the outcome of a collision. For instance, if the only force that can change the motion of m1 and m2 is the force between them, then both linear and angular momentum will be conserved in the system consisting of m1.
Example of Collision of Point masses
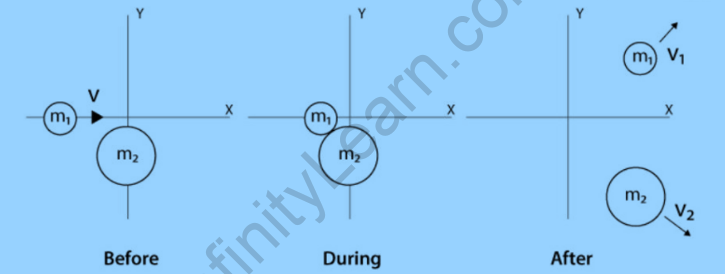
A collision sequence displaying m1 and m2 ‘s initial and final velocities. As in the circumstance depicted in the image above, where only m1 is moving prior to the collision, we can immediately conclude that at least one of the masses should be in motion after the collision to carry off the initial momentum.
- Conservation of linear momentum: Regardless of what happens during the collision, all components of linear momentum must be conserved.
- Conservation of Angular Momentum: Because a rigid body has two parts, angular momentum is a little more complicated. When motion is restricted to two dimensions, the translational angular momentum must be perpendicular to the plane of motion.
- Kinetic Energy: Even though unlikely for macroscopic objects, the kinetic energy may remain constant during the collision. The kinetic energy of a rigid object, like angular momentum, can be thought of as the sum of a piece due to the translation of the centre of mass and a part as a result of rotation about the centre of mass. Because we don’t know much about what happens when two objects collide, we’ll have to test whether our collisions are elastic or inelastic. After a two-dimensional collision with known starting conditions, there are four unknown linear velocity components and two angular speeds.
As a result, the final velocities must be determined by the details of the forces acting during the collision. In theory, measuring the final velocities for different initial conditions can teach us about the interaction force between the particles.
Conservation of linear momentum
The conservation of linear momentum is a fundamental principle in physics that states that the total momentum of a closed system of objects remains constant if no external forces act on it. This principle is expressed mathematically as:
p1 + p2 + … + pn = p1′ + p2′ + … + pn’
where p is the momentum of an object, and the subscripts 1, 2, …, n denote the objects in the system before the collision, and the subscripts 1′, 2′, …, n’ denote the objects in the system after the collision.
This equation asserts that the sum of the momenta of all objects in the system before the collision is equal to the sum of the momenta of all objects in the system after the collision. This means that the total momentum of the system is conserved, even if the individual momenta of the objects change during the collision.
The conservation of linear momentum is a powerful tool for analyzing collisions and other interactions between objects. It allows us to predict the motion of objects after a collision, based on their initial velocities and masses, without knowing the details of the forces involved in the collision.
Conservation of Angular Momentum
The Conservation of Angular Momentum is a fundamental principle in physics that states that the total angular momentum of a closed system remains constant, unless acted upon by an external torque. This concept is essential in understanding rotational motion and is closely related to the Law of Conservation of Momentum.
Angular momentum is a measure of an object’s rotational motion and is calculated using the formula:
L = Iω
where L is the angular momentum, I is the moment of inertia, and ω is the angular velocity.
The total angular momentum of a system is the sum of the angular momentum of each individual object in the system. The Conservation of Angular Momentum states that if no external torque acts on the system, the total angular momentum of the system remains constant.
This principle can be demonstrated with a simple example. Consider a figure skater spinning on the ice. As the skater pulls their arms in, their moment of inertia decreases, causing their angular velocity to increase. This is due to the Conservation of Angular Momentum – the total angular momentum of the system (the skater and the ice) remains constant, so as the moment of inertia decreases, the angular velocity must increase to maintain the same total angular momentum.
The Conservation of Angular Momentum is a fundamental principle in physics that states that the total angular momentum of a closed system remains constant, unless acted upon by an external torque. This principle can be demonstrated with the example of a figure skater spinning on the ice, where the skater’s moment of inertia decreases as they pull their arms in, causing their angular velocity to increase to maintain the same total angular momentum.
Kinetic Energy
Kinetic energy is the energy an object possesses due to its motion. It is defined by the equation:
E=mv Where:
- KE is the kinetic energy
- m is the mass of the object
- v is the velocity of the object
This equation shows that the kinetic energy of an object is directly proportional to the square of its velocity and its mass. In other words, an object with a greater mass or higher velocity will have more kinetic energy.For example, a car moving at 50 miles per hour will have more kinetic energy than a bicycle moving at the same speed, due to the car’s greater mass. Similarly, a car moving at 100 miles per hour will have four times the kinetic energy of the same car moving at 50 miles per hour, due to the velocity being squared in the kinetic energy equation.Kinetic energy is an important concept in physics and is used to understand the energy associated with the motion of objects.
Collision of Point Mass FAQs
How do you find the mass after a collision?
Because the colliding objects move in the same direction after the collision, the total momentum is simply the total mass of the objects multiplied by their velocity.
What will happen if masses are different during a collision?
As per Newton's second law of motion, an object's acceleration is determined by both force and mass. As a result of the contact force generated during the collision, if the colliding objects have unequal masses, they will have unequal accelerations.
What happens to the masses in an elastic collision?
A collision occurs when a relatively small mass approaches a larger mass. Whenever a larger mass initially moves toward a smaller mass, both will maintain momentum in the same direction.







