Table of Contents
Introduction:
Strain is a change in dimension over the original dimension. Now we know dimension has the units of L thus this means it has no dimensional formula and has no units.
Strain= Change in dimension/Original dimension
Thus
Dimensional Formula of Strain= (M0L1T0)/(M0L1T0)
Strains can be divided into normal and shear strains on the basis of the force that causes conversion. Typical gravity is caused by the forces intertwined with the planes or with the opposite positions of objects, such as at low pressure in all directions or with a rod being pulled or pressed at length.
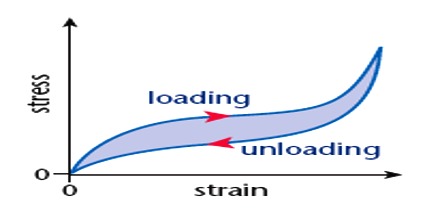
Engineering strain, also known as Cauchy strain:
It is expressed as the ratio of the total strain to the initial size of the material body upon which the forces are applied. The amount of strain in the direction of the applied force divided by the previous length of the material is called engineering strain.
Engineering normal strain or engineering extensive strain or nominal strain of a linear material element or axially loaded fibre of a material line element or axially loaded fibre is expressed as a change in length DL per unit of original length L of the line element or fibres. The state of deformation at a material point of a solid body is defined as the set of all variations in the length of material lines or fibres, the normal deformation passing through this point, as well as the set of all variations in the angle between pairs of lines, initially perpendicular to each other, the shear deformation emanating from this point.
The amount of elongation or compression of elements or fibres along the material line is the normal strain, and the amount of strain associated with the sliding of the planar layers against each other is the shear strain within the deformable body. The strain applied perpendicular to the cross-section is the normal strain, and the strain applied parallel to the cross-section is the shear strain. Shear strain occurs when the strain of an object is in response to shear stress (i.e., in the volume change equation, the ratio between shear stress and shear strain is called the shear modulus of the material.
Stress and strain are related by a governing law, and we can determine their relationship experimentally by measuring the amount of stress required to stretch a material.
From Hookes’ law and our definitions of stress and strain, we can easily derive a simple relationship for the strain of material. Normal deformation is a type of deformation caused by forces perpendicular to the plane or cross-sectional area of the material, such as in a member that is stretched or compressed lengthwise, or in a volume that is under pressure from all sides.
True strain measurements account for changes in the cross-sectional area using instantaneous area values. This means that the local and average deformations are the same, and the deformation can be determined taking into account the overall length. Based on the calculation results, a scalar value is constructed that characterizes hardening as a function of plastic deformations.
The elongation factor or elongation factor is a measure of the extensive or normal deformation of a differential linear element, which can be determined in an un deformed configuration or in a deformed configuration. A rod in uniaxial tension is stretched in uniaxial tension to a new length, and the normal deformation is the ratio of this small deformation to the initial length of the rods. Let’s try to understand with an example where the strain of a bar stretched in tension is the amount of stretch or change in length divided by its original length.
In deformation of volumes under pressure, the normal strain is expressed mathematically which is equal to the change in volume divided by the original volume.
In parts under tension or pressure – such as load-bearing balls and rollers, straight-laced shafts, or hardware for tightening and fastening – mechanical pressure and pressure features play an important role in determining whether a component can withstand application load conditions.
Explaining Stress-Strain Graph
The stress-strain diagram has different points which are:
-
Proportional limit
-
Elastic limit
-
Yield point
-
Ultimate stress point
-
Fracture or breaking point
Frequently used strain parameters includes:
- Strain
- Expressing the ability to shorten/thicken relatively to the original length in proportion
- Strain rate, which reflects that this proportional change occurs within a given time interval.
In this case, the strain can be measured indirectly from the speed or directly from the displacement using the same time interval.
The specific speed and displacement of each segment naturally translate into a different inter-segment speed, from which the strain rate and therefore strain values can be calculated, which are often used in strain imaging. As a tissue that moves and shifts in time, the left ventricle probably performs this function by changing shape (deformation), moving in different segments and at different speeds.
Deformations occurring in three orthogonal directions can give us a measure of the expansion of a material in response to a multiaxial load. New methods for measuring myocardial strain, such as point-tracking echocardiography, overcome the limitation of strain angle dependence associated with TDI. It can be shown that if the stress-strain curve of the material is convex or linear, then the prism deforms uniformly and a uniform state of strains and stresses develops inside the element.
Although the inside-out/top-to-bottom angular gradient that occurs at LV reflects the actual torsional and angular shear stress in angular form, its accurate calculation requires measuring 3D motion by observing time in linear and angular form.
Envision gives us six stresses and six strains (three normal and three shears), which we relate to each other using the generalized Hookes law for homogeneous, isotropic, and elastic materials.
FAQs:
Ques 1. What is the dimensional formula of strain?
Ans 1 :[M0 L0 T0] = Dimensionless Quantity.
Ques 2. Why do strains have no dimensions?
Ans 2: Because it is a ratio.
Ques 3. What is the mathematical definition of strain?
Ans 3: A measure of the amount an object deforms as a result of a force.



