Important Topic: Voids

Voids are actually holes between component particles. The unoccupied area between the component particles of a compact packed structure is referred to as a void in solid states. Close packing in solids may be accomplished in three ways: one-dimensional (1D), two-dimensional (2D), and three-dimensional (3D).

We perceive empty gaps between the atoms in 2-dimensional structures when the atoms are organised in square close packing and hexagonal close packing. These empty spaces are known as voids, and in the case of hexagonal packing, these voids have a triangle form and are known as triangular voids.

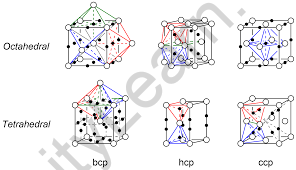
Tetrahedral and Octahedral Voids

These triangular voids may be found in two distinct orientations in a hexagonal packing. The apex of one row’s triangle points upward, while the apex of the other row’s triangle points downward.

In the three-dimensional structure, approximately 26% of total space is unoccupied and not filled by spheres in CCP and HCP near packing in solids. These empty areas are known as interstitial voids, interstices, or gaps. The voids in solids described above are proportional to the number of spheres present.

There are two kinds of interstitial spaces in a three-dimensional structure:

Tetrahedral Voids:

In a cubic close-packed structure, the spheres of the second layer are above the triangular voids of the first layer. Each sphere hits one of the three spheres in the first layer. By uniting the centres of these four spheres, it makes a tetrahedron, and the space formed by joining the centres of these spheres produces a tetrahedral vacuum. The amount of tetrahedral voids in a closed packed structure is twice the number of spheres. Let n be the number of spheres. The number of tetrahedral voids will therefore be 2n.

Tetrahedral void characteristics

• The empty space, or nothingness, is surrounded by four atomic spheres. As a result, the tetrahedral void’s coordination number is 4.
• The atom in the tetrahedral void interacts with four atoms located at the four corners of a tetrahedron.
• When a triangular void consisting of coplanar atoms comes into touch with the fourth atom above or below it, this void is generated.
• The vacuum has a substantially lower volume than the spherical particle.
• The radius of the tetrahedral vacuum is 0.225 R if R is the radius of the component spherical particle.
• If N is the number of close-packed spheres, then 2N is the number of tetrahedral voids.

Covering Tetrahedral Voids:

The spheres of the third layer may cover the tetrahedral spaces of the second layer. The spheres of the third layer are perfectly aligned with those of the first layer in this scenario. As a result, the spherical pattern is repeated in alternating levels. This pattern is frequently written as ABAB……. pattern. This is known as a hexagonal close-packed (HCP) structure.

Many metals, including magnesium and zinc, have this type of atom arrangement.

Octahedral Voids:

Octahedral voids can be found next to tetrahedral voids. Tetrahedral voids are close to octahedral voids. When the triangular voids of the first layer meet with the triangular voids of the layer above or below it, a void made by encapsulating six spheres is generated. Octahedral Voids are the empty spaces generated by merging the triangular voids of the first and second layers. The space formed by merging the triangular voids of the first and second levels is referred to as Octahedral Voids. If there are n spheres in a close-packed configuration, there will be n octahedral voids.

Octahedral Voids Characteristics:

• Six atomic spheres encircle the empty area of nothingness. As a result, the tetrahedral vacancy has a coordination number of 6.
• The atom in the octahedral void interacts with six atoms located at the six corners of an octahedron.
• This void is created by two sets of six equilateral triangles oriented in opposing directions.
• The vacuum has a modest volume.
• The radius of the octahedral vacuum is 0.414 R if R is the radius of the component spherical particle.
• If N is the number of close-packed spheres, then N is the number of octahedral voids.

Covering Octahedral Voids:

The third layer can be positioned above the second layer such that its spheres fill the octahedral voids. The spheres of the third layer are not aligned with those of the first or second layers when arranged in this manner. This is known as a ‘C’ type configuration. Only after the fourth layer is added are the spheres of the fourth layer aligned with those of the first layer, as depicted. This layering pattern is frequently written as ABCABC……….. The cubic close-packed (CCP) or face-centered cubic (FCC) structure is the name given to this structure. In this structure, metals such as copper and silver crystallize.

The number of Voids

The amount of these two kinds of voids is proportional to the number of closed-packed spheres.

If N is the number of closely packed spheres, then

• N is the octahedral void
• The tetrahedral void has a value of 2N.

The primary distinction between Tetrahedral and Octahedral Voids:

Tetrahedral voids are unfilled empty spaces found in materials with a tetrahedral crystal structure. Octahedral voids are empty spaces in substances with an octahedral structural structure. It is present in compounds with a tetrahedral structure in their crystal system. A tetrahedral void in a crystal is a simple triangular void surrounded by four spheres aligned tetrahedrally around it. An octahedral void, on the other hand, is a double triangular void surrounded by six spheres with one triangle vertex upwards and the second triangle vertex below.

FAQ’s

What exactly are octahedral sites?

An octahedral location is a place in the centre of an octahedron made up of six atoms. The octahedral area is larger than the tetrahedral area. Because atoms of the same size are packed as closely as possible together, each atom has one octahedral site.

In BCC, how many octahedral sites are there?

It is well known that both spheres are hard and collide. The six spheres depict a regular octahedra, and there is a given space in its interior for an interstitial atom, which is surrounded by six spheres. Octahedral sites may be found in crystals like FCC and BCC.

What exactly are interstitial voids?

Vacuums in solid states are defined as empty space between component particles in a closed packed system. These empty areas are referred to as apertures, interstices, or interstitial voids.