Table of Contents
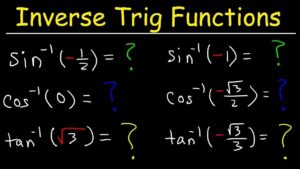
Inverse trigonometric functions are the inverse functions of the basic trigonometric functions sine, cosine, tangent, cotangent, secant, and cosecant functions. Arcus functions, anti trigonometric functions, and cyclometric functions are all terms used to describe them. In trigonometry, these inverse functions are used to get the angle with any of the trigonometric ratios. In engineering, physics, geometry, and navigation, inverse trigonometry functions are extremely useful.
Inverse trigonometric functions
Inverse trigonometric functions are sometimes known as “Arc Functions” since they create the length of arc required to acquire a given value of trigonometric functions. Inverse trigonometric functions, such as sine, cosine, tangent, cosecant, secant, and cotangent, conduct the opposite action of trigonometric functions such as sine, cosine, tangent, cosecant, secant, and cotangent. We already know that trigonometric functions are particularly useful in right-angle triangles. When the measurements of two sides of the triangle are known, these six key functions are employed to obtain the angle measure in the right triangle.
Graphs of Inverse Trigonometric Functions
For each trigonometry ratio, there are six inverse trig functions in particular. Six significant trigonometric functions have inverses:
- Arcsine
- Arccosine
- Arctangent
- Arccotangent
- Arcsecant
- Arccosecant
Derivatives of Inverse Trigonometric Functions
Inverse trigonometric functions’ derivatives are first-order derivatives. Let’s look at the derivatives of all six inverse functions in this section.
| Inverse Trig Function | dy/dx |
| y = sin-1(x) | 1/√(1-x2) |
| y = cos-1(x) | -1/√(1-x2) |
| y = tan-1(x) | 1/(1+x2) |
| y = cot-1(x) | -1/(1+x2) |
| y = sec-1(x) | 1/[|x|√(x2-1)] |
| y = csc-1(x) | -1/[|x|√(x2-1)] |
Inverse Trigonometric Functions Table
Let’s rebuild all of the inverse trigonometric functions in this section, including their notation, definition, domain, and range.
| Function Name | Notation | Definition | Domain of x | Range |
| Arcsine or inverse sine | y = sin-1(x) | x=sin y | −1 ≤ x ≤ 1 |
|
| Arccosine or inverse cosine | y=cos-1(x) | x=cos y | −1 ≤ x ≤ 1 |
|
| Arctangent or
Inverse tangent |
y=tan-1(x) | x=tan y | For all real numbers |
|
| Arccotangent or Inverse Cot |
y=cot-1(x) | x=cot y | For all real numbers |
|
| Arcsecant or
Inverse Secant |
y = sec-1(x) | x=sec y | x ≤ −1 or 1 ≤ x |
|
| Arccosecant | y=csc-1(x) | x=csc y | x ≤ −1 or 1 ≤ x |
|
FAQs
Q. What are the names of the six inverse trig functions?
Ans: Sine, cosine, tangent, secant, cosecant, and cotangent are the six fundamental trigonometric functions. Inverse sine, inverse cosine, inverse tangent, inverse secant, inverse cosecant, and inverse cotangent are the inverse trigonometric functions of these.
Q. Which of the three fundamental inverse trigonometric functions are you familiar with?
Ans: When two side lengths are known, the inverse trigonometric functions sin1(x), cos1(x), and tan1(x) are used to obtain the unknown measure of an angle in a right triangle.
Q. What is the procedure for determining the inverse of a trig function?
Ans: Solve for the statement “x is equal to the angle whose sine is 1/2” to obtain the inverse of an equation like sin x = 1/2. This sentence is written as x = sin–1(1/2) in trig. A –1 is placed in the superscript position in this notation.