JEE Main Maths Coordinate Geometry Previous Year Questions With Solutions

Introduction

The coordinate geometry is a specific part of arithmetic that gives the connection between variable based math and calculation by utilizing charts. Coordinate geometry assists us with tracking down the point’s situation on the plane by utilizing the arranged sets. Two fanciful lines mostly establish coordinate math: one is an upward line, and the other is a flat/horizontal line. Let us look at the JEE Main Maths Coordinate Geometry Previous Year Questions With Solutions.

Enroll Now to JEE Dropper Course

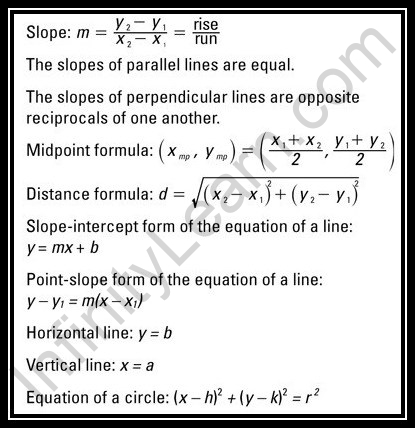
Coordinate Geometry Previous Year Questions are as follows:

Question 1: The equations of two equal sides of an isosceles triangle are 7x − y + 3 = 0 and x + y − 3 = 0 and the third side passes through the point (1, 10). The equation of the third side is ___________.

Solution:

Any line through (1, 10) is given by y + 10 = m (x − 1)

Since it makes equivalent point say α with the given lines 7x − y + 3 = 0 and x + y − 3 = 0, in this way tan α = [m − 7]/[1 + 7m]

= [m − (−1)]/[1 + m (−1)]

⇒ m = [1/3] or 3

Henceforth, the two potential conditions of the third side are 3x + y + 7 = 0 and x − 3y − 31 = 0.

Question 2: A-line through A (−5, − 4) meets the lines x + 3y + 2 = 0, 2x + y + 4 = 0 and x − y − 5 = 0 at B, C and D, respectively. If (15 / AB)² + (10 / AC)² = (6 / AD)², then the equation of the line is _________.

Solution:

[x + 5]/[cosθ] = [y + 4]/[sinθ] = r1/AB = r2/AC = r3/AD
(r1 cosθ − 5, r1 sinθ − 4) lies on x + 3y + 2 = 0

r1 = 15/[cosθ + 3 sinθ]

Likewise, 10/AC = 2 cosθ + sinθ and 6/AD = cosθ − sinθ

Placing in the given connection, we get (2 cosθ + 3 sinθ)2 = 0

tan θ = −2/3

⇒ y + 4 = [−2/3] (x + 5)

2x + 3y + 22 = 0

Question 3: The graph of the function cosx cos (x + 2) − cos² (x + 1) is

A) A straight line passing through (0, −sin² 1) with slope 2

B) A straight line passing through (0, 0)

C) A parabola with vertex 75^o

D) A straight line passing through the point (π / 2, −sin² 1) and parallel to the x-axis

Solution:

y = cos (x + 1 −1) cos (x + 1 + 1) − cos2 (x + 1)

= cos2 (x + 1) −sin2 1 − cos2 (x + 1) = −sin2 1, which addresses a straight line corresponding to x-pivot with y = −sin2 1 for all x thus additionally for x = π/2.

Enroll Now to JEE Class 12 Course

Question 4: In what direction can a line be drawn through the point (1, 2) so that its points of intersection with the line x + y = 4 is at a distance √6 / 3 from the given point?

Solution:

Let the expected line through the point (1, 2) be leaned at a point θ to the pivot of x.

Then, at that point, its condition is [x − 1]/[cosθ] = [y − 2]/[sinθ] = r … ..(I)

where r is the distance of any point (x, y) on the line from the point (1, 2).

The directions of any point on the line (I) are (1 + r cosθ, 2 + r sinθ).

On the off chance that this point is a ways off √6/3 structure (1, 2), then, at that point, r = √6/3.

Along these lines, the fact is (1 + [√6/3] cosθ, 2 + [√6/3] sinθ).

In any case, this point lies on the line x + y = 4.

√6/3 (cosθ + sinθ) = 1 or

sinθ + cosθ = 3/√6

[1/√2] sinθ + [1/√2] cosθ = √3/2, {Dividing the two sides by √2}
sin (θ + 45°) = sin60° or sin 120°

θ = 15° or 75°

Question 5: A variable line passes through a fixed point P. The algebraic sum of the perpendicular drawn from (2, 0), (0, 2) and (1, 1) on the line is zero, then what are the coordinates of the P?

Solution:

Let P (x1, y1), then, at that point, the condition of the line going through P and whose slope is m, is y − y1 = m (x − x1).

Presently as indicated by the condition

[{−2m + (mx1 − y1)}/{√1 + m2}]+ [{2 + (mx1 − y1)}/{√1 + m2} + {1 − m + (mx1 − y1)}/{√1 + m2}=0
3 − 3m + 3mx1 − 3y1 = 0

⇒ y1 − 1 = m (x1 − 1)

Since it is a variable line, so hold for each value of m.

Along these lines, y1 = 1, x1 = 1

⇒ P(1,1)

Question 6: The area enclosed within the curve |x|+|y|= 1 is ____________.

Solution:

The given lines are ± x ± y = 1 for example x + y = 1, x − y = 1, x + y = −1 and x − y = −1.

These lines structure a quadrilateral whose vertices are A (−1, 0), B (0, −1), C (1, 0) and D (0, 1) Obviously ABCD is a square.

Length of each side of this square is √12 + 12 = √2

Henceforth, the area of square is √2 * √2 = 2 sq.units

Stunt: Required region = 2c2/|ab| = [2 * 12]/[|1 * 1|] = 2.

Question 7: The locus of a point P, which divides the line joining (1, 0) and (2 cosθ, 2 sinθ) internally in the ratio 2 : 3 for all θ, is a ________.

Solution:

Let the directions of the point P, what isolates the line joining (1, 0) and (2 cosθ, 2 sinθ) in the proportion 2 : 3 be (h, k). Then, at that point,

h = [4 cosθ + 3]/[5] and k = [4 sinθ]/[5]

cosθ = [5h − 3]/[4] and sinθ = [5k]/[4]

([5h − 3]/[4])2 + ([5k]/[4])2 = 1

(5h − 3)2 + (5k2) = 16

Thus, locus of (h, k) is (5x − 3)2 + (5y)2 = 16, which is a circle.

Question 8: The area of a parallelogram formed by the lines ax ± by ± c = 0, is __________.

Solution:

It is a rhombus whose area is given by = [1 / 2] AC * BD = [ 1 / 2] * [2c / a] * [2c / b] = 2c² / ab.

Enroll Now to JEE Class 11 Course

Question 9: If the sum of the distances of a point from two perpendicular lines in a plane is 1, then its locus is ________.

Solution:

Required locus of the point (x, y) is the curve|x| + |y| = 1.

On the off chance that the point lies in the principal quadrant, x > 0, y > 0 thus |x| + |y| = 1

⇒ x + y = 1, which is straight line AB.

On the off chance that the point (x, y) lies in the second quadrant x < 0, y > 0 thus |x| + |y| = 1

⇒ −x + y = 1.

Essentially, for the third and fourth quadrant, the conditions are −x −y = 1 and x − y = 1.

Subsequently, the expected locus is the bend comprising of the sides of the square.

Question 10: The line 2x + 3y = 12 meets the x-axis at A and y-axis at B. The line through (5, 5) perpendicular to AB meets the x-axis, y-axis and the AB at C, D and E, respectively. If O is the origin of coordinates, then the area of OCEB is _______.

Solution:

Here O is the point (0, 0).

The line 2x + 3y = 12 meets the y-pivot at B thus B is the point (0, 4).

The condition of any line opposite to the line 2x + 3y = 12 and goes through (5, 5) is 3x − 2y = 5 … … (I)

The line (I) meets the x-pivot at C thus facilitates of C are (5/3, 0).

Essentially, the directions of E are (3, 2) by settling the lines AB and (I).

Subsequently, O (0, 0), C (5/3, 0), E (3, 2) and B (0, 4).

Presently the area of figure OCEB = area of ΔOCE + area of ΔOEB = [23/3] sq.units.

FAQs

Is Coordinate Geometry important from a JEE point of view?

Coordinate calculation is a significant section of math according to the JEE perspective. The section isn't just in the schedule of arithmetic yet additionally one of the most simple to score parts in JEE. Consistently around 20 to 25 per cent of the complete imprints in science comes from this part. From the coordinate calculation, around 50% of the inquiries come from the straight line and the circles.

How can Coordinate geometry be helpful in our daily lives?

Besides the test perspective, coordinate math is exceptionally useful and can be applied in our day to day routines to take care of different issues. The idea of direction math is extremely helpful in the aeroplane business, particularly when they are managing the aeroplane fuselage. The coordinate calculation is utilized in scanners as well as control pictures.

What do we Understand by Polar Coordinates?

Polar facilitates fundamentally characterizes the place of a point as (r, Ө), in which r represents the distance of a point from the beginning and Ө → represents the point from the positive x-hub forthright.