Table of Contents
Everywhere in the universe, there is rotational motion. Rotational motion is demonstrated by the movement of electrons around an atom and the movement of the moon around the earth. When an object exhibits rotational motion, it cannot be treated as a particle because different parts of the object move at different velocities and accelerations. As a result, the object must be regarded as a particle system. The dynamics of rotational motion are identical to those of linear or translational motion. Many of the equations for rotating object mechanics are similar to linear motion equations. Only rigid bodies are considered in rotational motion. A rigid body is a mass-bearing object with a rigid shape.
Kinematics is the study of motion. The relationships between rotation angle, angular velocity, angular acceleration, and time are described by rotational motion kinematics. Torque is the ability of a force to alter the rotational speed of an object. The rotational equivalent of force is torque. The distance between the point of rotation (pivot point) and the location where force is applied is defined as the lever arm. Torque is maximized by applying a force perpendicular to the lever arm and as far away from the pivot point or fulcrum as possible. If the torque is zero, the angular acceleration is also zero.
Overview:
When all parts of a rigid body move parallel to a fixed plane, the object is said to be in-plane motion. There are two types of plane motion, which are as follows:
- Pure rotational motion: In this motion, the rigid body rotates about a fixed axis that is perpendicular to a fixed plane. To put it another way, the axis is fixed and does not move or change direction in relation to an inertial frame of reference.
- The general plane motion is as follows: The motion, in this case, can be thought of as a combination of pure translational motion parallel to a fixed plane and pure rotational motion about an axis perpendicular to that plane. The kinematics and dynamics of pure rotational motion are covered in this chapter.
Consider a rigid body that is performing only rotational motion (i.e., a rotational motion which has no translational component). It is possible to define an axis of rotation (which is assumed to pass through the body for simplicity)—this axis corresponds to the straight-line that is the locus of all points inside the body that remains stationary as the body rotates. A general point inside the body performs a circular motion that is centered on the rotation axis and oriented perpendicular to this axis.
The following assumes implicitly that the axis of rotation remains constant.
Rotational motion physics:
We discussed motion in a circle at a constant speed and, thus, constant angular velocity in the section on uniform circular motion. However, angular velocity is not always constant—rotational motion can speed up, slow down, or reverse directions. When a spinning skater pulls in her arms, a child pushes a merry-go-round to make it rotate, or a CD slows to a halt when turned off, the angular velocity is not constant. Because the angular velocity changes in all of these cases, angular acceleration occurs. The greater the angular acceleration, the faster the change occurs. The rate of change of angular velocity is defined as angular acceleration. Angular acceleration is expressed as an equation.
a=Δωt / Δt
where,
Δω =the change in angular velocity and t is the change in time.
The units of angular acceleration are (rad/s)/s, or rad/s 2 .
If ω increases, then α is positive. If decreases, then are negative.
Keep in that, by convention, counterclockwise is the positive direction and clockwise is the negative direction.
Rotational Motion Examples:
Rotation of Earth:
The motion of the earth and other planets about their respective axes is an example of rotatory motion, as the name implies. The spinning of the celestial bodies about their axis is caused by inertia.
Wheels of moving vehicles:
A vehicle’s wheels rotate in relation to the axle. This rotation of the wheels aids in moving the vehicle forward or backward. The motion of the wheels demonstrates rotatory motion, whereas the motion of the car is the result of rotational motion being converted into linear motion.
Spinning top:
A spinning top is a toy with a pointed tip that is externally wrapped in thread. One of the best examples of rotatory motion is the movement of a spinning top. When the spinning top is placed on a surface with only its pointed end in contact with the ground and the thread is pulled with force, the top spins about its own axis until all of the top’s energy is consumed.
In our daily lives, we see examples of rotational motion.
- One example of rotational motion is the rotation of the earth about its own axis, which causes the cycle of day and night.
- Rotational motion is the movement of a wheel, gears, motors, and so on.
- The motion of the helicopter’s blades is also rotatory motion.
- A door that swivels on its hinges as it is opened or closed.
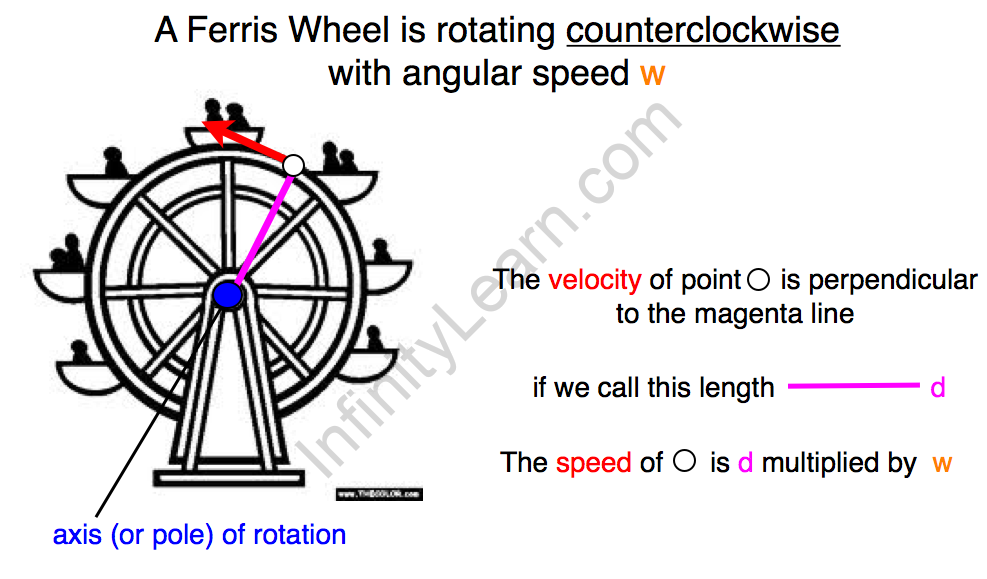
- The motion of a Ferris Wheel in an amusement park.
Define Rotational Motion:
As the name implies, rotational motion is defined as the motion of a point in a circular path, with the centre point fixed on the fixed axis of rotation. Rotational kinematics is the study of rotational motion without regard for its causes, whereas rotational dynamics is the study of rotational motion with regard for its causes as well as its properties.
Because rotational motion is more complicated than linear motion, only rigid body motion will be considered here. In contrast to the sun, which is a ball of gas, a rigid body is an object with a mass that holds a rigid shape, such as a phonograph turntable. Many of the equations for the mechanics of rotating objects are similar to linear motion equations.
Rotational motion is defined as the movement of an object in a fixed orbit around a circular path. It can also be defined as a body’s motion in which all of its particles move in a circular motion with a common angular velocity around a fixed point, such as the rotation of the Earth around its axis. An object’s rotation about a fixed point can occur in two directions: clockwise and anticlockwise. Rotational energy is the energy produced by this rotational motion. Torque, moment of inertia, angular momentum, and other important terms are associated with rotational motion.
Torque is the magnitude of force applied to a body away from the point of application of force. It is also known as the turning effect. It is easier, for example, to push a door open at its ends rather than at its hinges.
Moment of Inertia: This is a quantity that determines how much torque is required to rotate an object around its axis. It is also known as rotational inertia.
Angular momentum is the product of angular velocity and moment of inertia, and it determines the amount of rotation of the body.
Also read: NEET Exam Pattern 2022
Frequently Asked Questions
What is Rotational Motion?
Rotational motion is defined as the movement of an object in a fixed orbit around a circular path.
What are the examples of rotational motion about a fixed axis?
Rotation about a fixed point can be seen in the rotation of a ceiling fan, the rotation of the minute and hour hands in a clock, and the opening and closing of a door.
What are the examples of rotational motion about an axis of rotation?
Pushing a ball from an inclined plane is the best example of rotation about an axis of rotation. The ball travels to the bottom of the inclined plane via translational motion, whereas rotational motion occurs as the ball rotates around its axis.









