Table of Contents
Introduction:
What are Scalar and Vector Products? Physics’ core notions have a mathematical foundation. Physical quantities are all quantifiable quantities. A scalar and a vector are two mathematical numbers that can be used to explain the motion of objects. A scalar quantity can be described entirely in terms of the magnitude of numbers. Scalar quantities include length, mass, distance, energy, volume, and so on.
To fully define a vector quantity, it must have both a magnitude and a direction. Displacement, velocity, weight, dipole moment, and other vector quantities are examples.
There are two ways to multiply vector quantities.
- Dot or scalar product
- Cross-product or vector
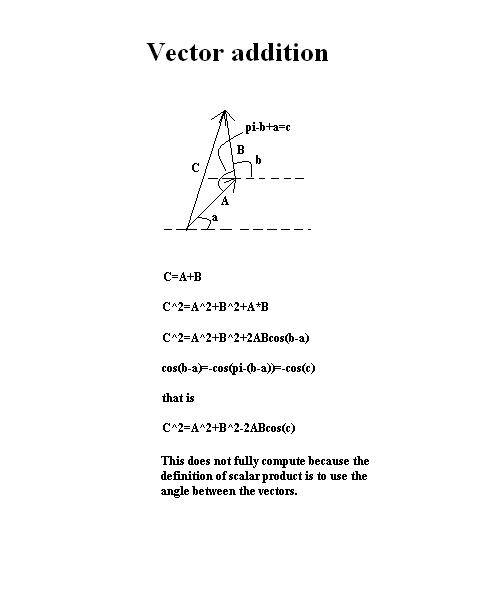
Whenever two vectors are scalarized, you have a number or a scalar. When it comes to identifying energy and work relationships, scalar products come in handy. The labour performed by a Force (which is a vector) in displacing (a vector) an object is given by the product rule of Force and Displacement vectors, which is an example of a scalar product. The scalar product is indicated by a dot (.), and its formula is as follows:
X. Y = XY Cos θ,
where is the angle formed by the two vectors?
Due to the obvious dot notation employed in it, the scalar product is also known as the dot product.
A Brief outline
- In physics and astronomy, the scalar and vector products, are the two techniques of multiplying vectors that are used the most. Take the component of one vector in the path of the other and multiply it by the magnitude of the other vector to get the scalar product of the two vectors.
- The scalar product is essential in geometry for determining the direction of arbitrary vectors in spacetime. Rather than representing vectors as unit vectors as in the scalar product method, it is sometimes more convenient to represent them as row or column matrices.
- The sum of the products of the respective spatial components of these two matrices would be the result of the matrix product of these two matrices. The scalar product of the two vectors yields this number. The scalar product of two vectors, when written in this fashion, depicts the method employed in matrix multiplication, in which the total of the products of the components of a row and column yields a single value.
Important concepts related to scalar and vector products
- It is possible to multiply a vector by some other vector, but do not split it. In physics and engineering, there are two types of vector components that are commonly employed. One sort of multiplication is the scalar multiplication of two vectors. The scalar product of two vectors produces a number, as the name implies (a scalar).
- Scalar products are used to define the link between energy and work. For example, the work that a force (a vector) accomplishes on an item while creating its displacement is defined as the scalar product of the force vector and the displacement vector (a vector).
- The vector multiplication of vectors is a kind of multiplication that is quite different. As the name implies, the vector product of two vectors produces a vector. Vector products are used to define other derived vector values.
- In defining rotations, a vector variable called torque is defined as the vector product of a force applied (a vector) and its displacement from the pivot to the force (a vector). It is critical to distinguish between the two types of vector multiplications since a scalar product is a scalar quantity and then a vector product is a vector quantity.
Crack NEET with Result-Oriented Learning Program from Infinity Learn
FAQs
Question: As a matrix, how do you express the scalar product of two vectors?
Answer: Rather than representing vectors as unit vectors, it is sometimes more helpful and easy to represent them as row or column matrices. If we represent a vector’s x, y, and z coordinates as a column matrix, we can get row matrices by transposing them. As a result, we can write: XT = [Xx Xy Xz]
The matrix product of the two vectors above yields a single integer, the scalar product of the two vectors.
Infinity Learn App
Now you can find answers to all your subject queries & prepare for your Exams on our Educational App – Infinity Learn.






