Dimensional Formula Of Reynolds Number

Dimensional Formula Of Reynolds Number What is Reynolds number? How do you determine Reynolds number? What are its uses? This article will answer all your questions along with the definition and, the dimensional formula for the Reynolds number. Continue reading for complete information on Reynolds Number.

The Reynolds number addresses the proportion of inertial powers to thick powers and is an advantageous boundary for anticipating assuming that a stream condition will be laminar or tempestuous. It is characterized as a trademark length duplicated by a trademark speed and separated by the kinematic consistency.

Key points on Reynolds Number

• Osborn Reynolds found that the stream system relies principally upon the proportion of the latency powers to thick powers in the liquid.
• At the point when the gooey powers are prevailing (slow stream, low Re), they are adequate to keep every one of the liquid particles in line, then, at that point, the stream is laminar.
• At the point when the inertial powers rule over the thick powers (when the liquid streams quicker and Re is bigger), the stream is tempestuous.
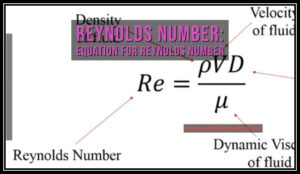
• It is characterized as: Reynolds number in which V is the mean stream speed, D is a trademark straight aspect, ρ liquid thickness, μ dynamic consistency, and ν kinematic thickness.
• The Reynolds number can be utilized to analyze what is going on (e.g.,, wind stream around an airfoil and water stream in a line) with a limited scale model.

Determining Reynolds number

1. Decide the sort of stream (inner, outer, consistent state, and so on)
2. Pick or compute the trademark straight aspect
3. From tables, decide the thickness and the thickness of the liquid.
4. Pardoned stream speed, work out the Reynolds number.

It is a dimensionless number involved the actual attributes of the stream. A rising Reynolds number demonstrates expanding choppiness of stream.

Liquid, Flow and Reynolds Number

The pertinence of the Reynolds number contrasts relying upon the determinations of the liquid stream like the variety of thickness (compressibility), variety of consistency (Non-Newtonian), being interior or outside stream, and so on The basic Reynolds number is the outflow of the worth to determine progress among systems which expands in regards to the sort of stream and math too.

While the basic Reynolds number for fierce stream in a line is 2000, the basic Reynolds number for tempestuous stream over a level plate, when the stream speed is the free-stream speed, is in a reach from 10⁵ to 10⁶

The Reynolds number additionally predicts the thick conduct of the stream in the event that liquids are Newtonian. In this way, it is profoundly critical to see the actual case to stay away from erroneous expectations. Change systems and inward and outside streams are the fundamental fields to thoroughly examine the Reynolds number. Newtonian liquids are liquids that have a consistent thickness. Assuming the temperature remains something very similar, it doesn’t make any difference how much pressure is applied on a Newtonian liquid; it will constantly have a similar consistency. Models incorporate water, liquor and mineral oil.

Laminar to Turbulent Transition

The liquid stream can be determined under two unique systems: Laminar and Turbulent. The progress among the systems is a significant issue that is driven by both liquid and stream properties. As referenced previously, the basic Reynolds number can be named inside and outside. However while the Reynolds number with respect to the laminar-tempestuous progress can be characterized sensibly for inner stream, it is difficult to indicate a definition for outer stream.

Use of the Reynolds Number

The mathematical arrangement of liquid stream depends on numerical models that have been created by both test review and related actual regulations.

• One of the critical stages all through the mathematical assessment is to decide a proper numerical model that mimics the actual space.
• To acquire a sensibly decent forecast for the conduct of liquids under different conditions, the Reynolds number has been acknowledged as a significant essential for liquid stream examination.
• For example, development of glycerin in a round pipe can be anticipated by the Reynolds number

Dimensional Formula of Reynolds Number (R_e)

What is the dimensional formula of Reynolds number? Well, here is the answer. The dimensional formula of Reynolds number after derivation is found to be M⁰ L⁰ T⁰

• At the point when fluid courses through the line, it hits the line. The designers ensure that the fluid course through a line all around the city ought to be essentially as consistent as could really be expected.
• Thus, for this, a number called Reynolds number predicts in the event that the progression of the fluid will be consistent or tempestuous.
• Sir George Stoke presented this idea interestingly. Later on, it was promoted by Osborne Reynolds, then, at that point, the name of this number was given as Reynolds number.
• Reynolds number is an unadulterated number that decides the progression of fluid through a line.

As per Reynold, the basic speed vₙ of a fluid coursing through a container of the distance across D is given by

vₙ = Nᵣη/ρD

Or on the other hand Nᵣ = ρDvₙ/η

Where η is the coefficient of thickness of the fluid coursing through the cylinder

ρ = thickness of the fluid

Nᵣ = It is a consistent called as a Reynold number

Here, vₙ is the basic speed.

Highlight be Noted

• The normal speed of the liquid isn’t something similar at every one of the spots in the line.
• It implies in the middle of the line; the speed is most extreme, while at the surfaces, the speed is lesser, you can say near nothing, not by and large zero on account of the rubbing presented by the dividers of the line.

FAQs

How is Reynolds number determined?

The Reynolds number (Re ) of a streaming liquid is determined by duplicating the liquid speed by the inside pipe measurement (to acquire the latency power of the liquid) and afterward isolating the outcome by the kinematic thickness (gooey power per unit length).

What does Reynolds number demonstrate?

The Reynolds number is utilized to decide if a liquid is in laminar or violent stream. In light of the API 13D proposals, it is accepted that a Reynolds number not exactly or equivalent to 2100 demonstrates laminar stream, and a Reynolds number more noteworthy than 2100 shows fierce stream.