Table of Contents
The expanded value of an algebraic expression of the form (x + y)n is found using the binomial theorem. Finding the value of (x + y)2, (x + y)3, (a + b + c)2 is simple and may be done simply by multiplying the exponent value by the number of times. The binomial theorem, on the other hand, can be used to find the expanded version of (x + y)17 or other expressions with greater exponential values. This binomial theorem expansion’s exponent value can be a fraction of a negative number. We restrict our explanations to only non-negative values in this case. Let’s look at the terms and attributes of the coefficients in this binomial expansion in more detail.
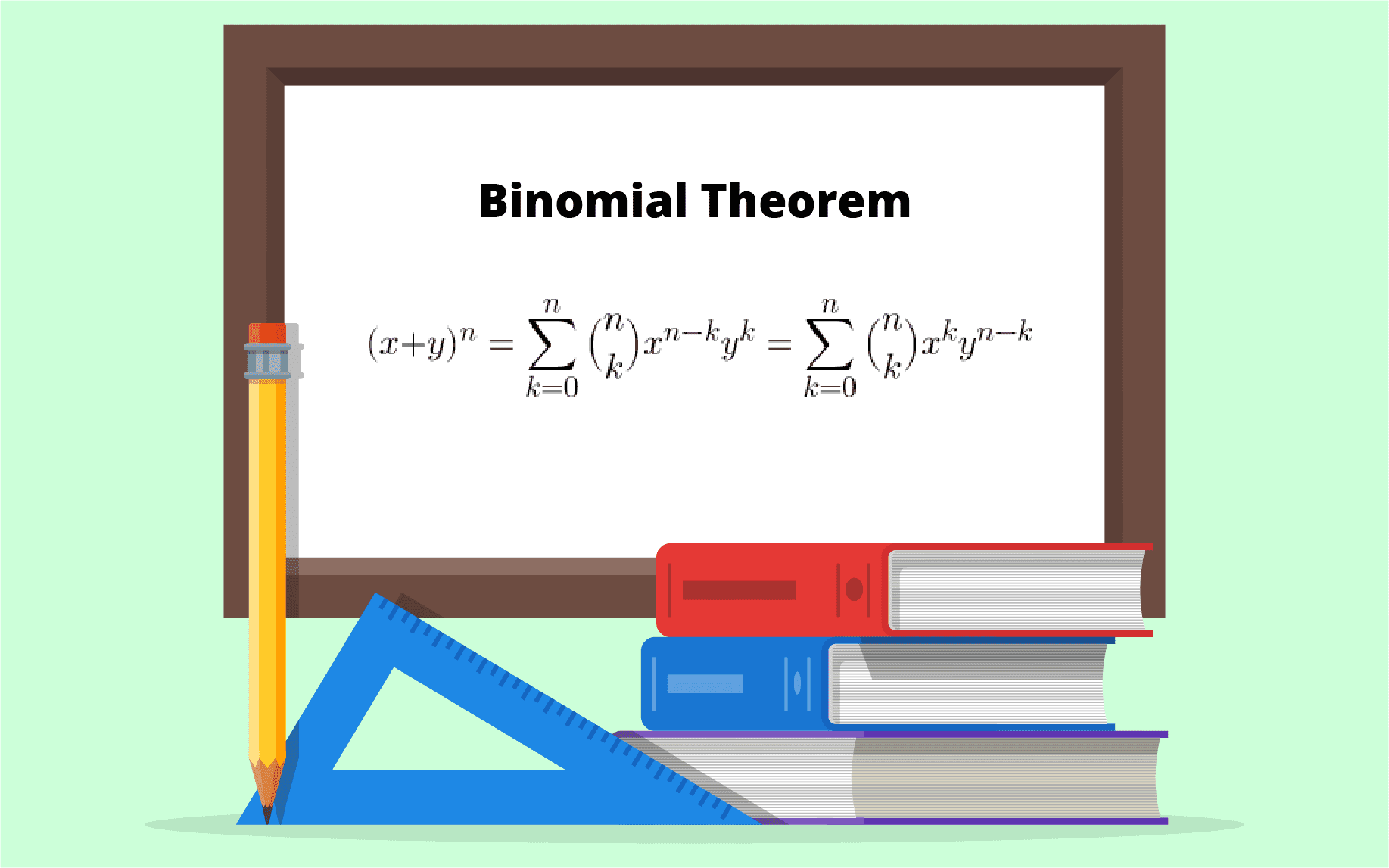
Definition of Binomial Theorem:
The binomial theorem was first mentioned in the 4th century BC by Euclids, a great Greek mathematician. The binomial theorem explains how to expand the algebraic statement (x + y)n to a sum of terms using individual exponents of variables x and y. A numeric value called coefficient is assigned to each term in a binomial expansion.
Binomial Expansion:
Binomial expansion of (x + y)n by using the binomial theorem is as follows,
(x +y)n = nC0xn+nC1xn-1y+nC2xn−2y2+…..+nCrxn−ryr+……nCnyn.
Properties of Binomial Theorem:
- The number of coefficients in the binomial expansion of (x + y)n is equal to (n + 1).
- There are (n+1) terms in the expansion of (x+y)n.
- The first and the last terms are xn and yn
- From the beginning of the expansion, the powers of x, decrease from n up to 0, and the powers of a, increase from 0 up to n.
- The general term in the expansion of (x + y) n is the (r +1)thterm that can be represented as Tr+1, Tr+1 = nCr X n-r yr
- The binomial coefficients in the expansion are arranged in an array, which is called Pascal’s triangle. This pattern developed is summed up by the binomial theorem formula.
- In the binomial expansion of (x + y)n, the rthterm from the end is (n – r + 2)th term from the beginning.
- If n is even, then in (x + y)nthe middle term = (n/2)+1 and if n is odd, then in (x + y)n, the middle terms are (n+1)/2 and (n+3)/2.
Coefficients of the Binomial Theorem:
In the expansion of (x + y)n, the binomial coefficients are the numbers associated with the variables x, y. The binomial coefficients are written as follows:
nC0,nC1,nC2 ….The binomial coefficients are calculated using either the pascal triangle or the combinations formula.
Pascal’s Triangle:
The values of the binomial coefficients follow a distinct pattern that can be seen as Pascal’s triangle. Pascal’s triangle is a triangular arrangement of binomial coefficients. It is named after Blaise Pascal, a French mathematician. All of the boundary components of the numbers in Pascal’s triangle are 1, and the remaining numbers within the triangle are arranged so that each number is the sum of two numbers close above it.
Combinations:
Using the binomial theorem, the formula for combinations is used to find the value of the binomial coefficients in the expansions. In this scenario, the combinations are distinct ways of selecting r variables from the available n variables.
The formula to find the combinations of r objects taken from n different objects is ncr=n!r!(n−r)!
Here the coefficients have the following properties.
- nC0=nCn=0
- nC1=nCn−1=n
- nCr= nCr−1
The following properties of binomial expansion can be derived by simply substituting simple numeric values of x = 1 and y = 1 in the binomial expansion of (x + y)n. The properties of binomial coefficients are as follows:
- C1+C2+C3+C4+…….Cn=2n
- C0+C2+C4+….=C1+C3+C5+…….= 2(n-1)
- C0−C1+C2−C3+C4−C5+……….(−1)nCn=0
- C1+2C2+3C3+4C4……….+nCn=n2n−1
- C1−2C2+3C3−4C4………+(−1)n−1nCn=0
Important Terms of Binomial Expansion:
The terms related to binomial expansion using the binomial theorem are listed below to help you find them. The following are the specifics of each of the terms.
General Word: This term represents all of the terms in the (x + y)n binomial expansion. In the binomial expansion of (x + y)n, the general term is
The r-value, in this case, is one less than the number of binomial expansion terms. In addition, is the coefficient, and the exponents of the variables x and y add up to n.
Middle Term: In the expansion of (x + y)n, the total number of terms is equal to n + 1. The binomial expansion’s middle term is determined by the value of n. The number of middle terms and the value of n is determined by whether n is even or odd. There is just one middle term for an even value of n, and it is the (n/2 + 1)th term. There are two middle terms for an odd number of n, and the two middle terms are n/2 and n/2 + 1.
Important Reminders:
The following crucial points can help you comprehend the binomial theorem better. n + 1 is the number of terms in the binomial expansion of (x + y)n. The sum of the powers of x and y in each term equals n in the expansion of (x + y)n. The binomial coefficients on both sides of the expansion have the same value. n(n + 1) is the number of terms in the binomial expansion of (x + y + z)n.
Also Read: JEE MAIN Maths Preparation Tips
FAQs
Is the binomial theorem simple in JEE?
The syllabus for class eight also includes some fundamental sums for an introduction to the Binomial Theorem. Once students are familiar with the derivation, the concept of the Binomial Theorem becomes clear.
In JEE mains, how significant is the binomial theorem?
Around 1-2 problems from the Binomial Theorem are asked, for a total of around 4 marks. As a result, the Binomial Theorem has a weightage of 1-2 percent in JEE Main. To learn more about the paper pattern, visit some educational site's Jee Main 2020 Mathematics Pattern.







