Table of Contents
Dimensions of Torque: Torque is indeed the rotational equivalent of linear force in physics and mechanics. Based on the field of study, it is also known as the moment, moment of force, rotational force, or turning effect. This denotes a force’s ability to cause a change in the rotational motion of the body. Archimedes’ studies on the use of levers inspired the concept. Like a linear force, a torque can be a twist to an object around a specific axis. Torque has been calculated as the product of force magnitude and the perpendicular distance of a force’s line of action from the axis of rotation.
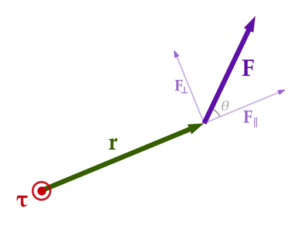
Torque has always been defined as a force applied perpendicularly to a lever multiplied by its distance from the lever’s fulcrum (the length of the lever arm). A three-newton force applied two metres from the fulcrum, for example, produces the same Torque as a one-newton force applied six metres from the fulcrum. The right-hand grip rule could be used to determine the torque direction: if the fingers of the right hand are curled from the lever arm’s direction into the force’s direction, then the thumb points in the direction of the Torque.
The Torque is a pseudovector in three dimensions; for point particles, it is given by the cross product of the position vector (distance vector) and the force vector. The magnitude of something like a rigid body’s Torque is determined by force applied, the lever arm vector connecting the Torque’s point is calculated to the point of force application, and the angle between the force and lever arm vectors.
Derivation of Dimensions of Torque
- We have seen, Torque (T) = Moment of Inertia × Angular Acceleration . . . . (1)
- It is known that Moment of Inertia (M.O.I.) = Radius of Gyration2 × Mass
- Therefore, the dimensional formula of Moment of Inertia = M1 L2 T0 . . . (2)
- Likewise, Angular Acceleration = Angular velocity × Time-1
- Therefore, the dimensional formula of Angular Acceleration = M0 L0 T-2 . . . (3)
- When substituting equations (2) and (3) in equation (1), we get,
- Torque = Moment of Inertia × Angular Acceleration
- That is, I = [M1 L2 T0] × [M0 L0 T-2] = [M L2 T-2].
- Thus, the Torque is dimensionally represented as [M L2 T-2].
Also Read: Dimensions Of Rotational Kinetic Energy | Dimensions Of Dipole Moment
FAQs
What is dimension formula?
The dimensional formula has been defined as the expression of a physical quantity in terms of its fundamental unit with appropriate dimensions.
What is the dimension and unit of torque?
Torque does have the dimension of force times distance, denoted by the symbol M L2 T-2. Even though the fundamental dimensions are the same as for energy or work, official SI literature recommends using newton metre (Nm) rather than joule.





