Moment of Inertia of Flywheel – JEE

Moment of Inertia of Flywheel: Flywheels seem to be circular disc-shaped objects that are used to store energy in machines. The flywheel has been made up of a large circular disc/massive wheel with a strong axle protruding on either side. The axle would be supported by two fixed supports and is mounted on ball bearings. Just on the axle, there is a small peg. The weight-hanger is carried by one end of a cord that is loosely looped around the peg.

The formula that is used to calculate the moment of inertia of a flywheel is:

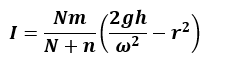
In which, I = moment of inertia of the flywheel. Here, the symbols denote;

m = rings’ mass.

N = flywheel rotation.

n = number of windings of the string.

h = height of the weight assembly.

g = acceleration due to gravity.

r = radius of the axle.

Similarly, we can also use the following expression;

mgh = ½ mv² + ½ Iω² + n₁E

Determining the Moment of Inertia of Flywheel

A few important factors must be considered when determining the moment of inertia of a flywheel. Initially, we must assemble a flywheel, as well as equipment such as a weight hanger, slotted weights, a metre scale, and a stopwatch.

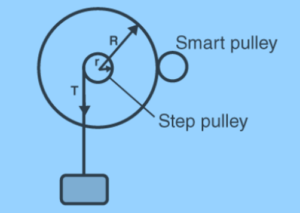
Afterwards, we make some assumptions. We’ll use mass (m) for both the weight hanger and the hanging ring. The maximum height will be (h). Just consider a situation in which the mass will descend to a new height. There’ll be some potential energy loss, which we can express as follows in the equation:

P_loss = mgh

In the meantime, when the flywheel and axle rotate, there is a gain in kinetic energy. We say it this way:

K_flywheel = (½) Iω²

I = moment of inertia

ω = angular velocity

Correspondingly, the kinetic energy of descending weight assembly is written as;

K_weight = (½) Iv²

Here, v = veocity

We must also consider the effort expended in overcoming the friction. It can be determined by;

W_friction = nW_f

In this particular instance,

n = number of windings of the string

W_f = work done in overcoming frictional torque

When we state the law of conservation of energy then we obtain;

P_loss = K_flywheel + K_weight + W_friction

Now, We’ll substitute the values and the equation will now become;

mgh = (½)Iω² + (½) mv² + nW_f

Shifting on to the next phase, we examine the kinetic energy of the flywheel assembly as it rotates (N) times against the frictional torque. We obtain;

NW_f = (½ ) Iω² and W_f = (1 / 2N) Iω²

Moreover, we establish a relationship between the weight assembly’s velocity (v) and the axle’s radius (r). The equation is as follows:

v = ωr

Now, we need to substitute the values for W_f and v.

mgh = (½) Iω² + (½ )mr²ω² + (n / N) x ½ Iω²

When we solve the equation for finding the moment of inertia of flywheel, we obtain;

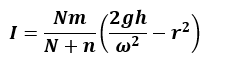
FAQs

What is meant by MOI of the flywheel?

The moment of inertia of a body has been defined as the object's resistance to rotational changes. The flywheel flexibly rotates about a horizontal axis. A calliper could be used to measure the radius of the flywheel's axile.

What is the main focus of the flywheel experiment?

The moment of inertia of a flywheel has been investigated by varying the flywheel's point of mass. The experiment has been carried out by recording the time taken by a fixed load until the point at which the load escapes from the flywheel, as well as the number of rotations performed afterwards, which is independent of the load.