Table of Contents
Introduction Radial nodes
Within an atom, there are two sorts of nodes: angular and radial. This section is dedicated to angular nodes, which are or will be explored in another section. Radial nodes, as the name implies, are determined radially. Places lacking electrons, or radial nodes, can be found using the radial probability density function.
Angular nodes are either x, y, or z planes that are devoid of electrons, whereas radial nodes are parts of these axes that are devoid of electrons.
Definition of Radial nodes
Radial nodes are often spherical locations with a 0% probability of detecting an electron. This sphere has a set radius. Radial nodes can thus be determined radially. When the principal quantum number grows, radial nodes form.
The primary quantum number is related to electron shells. The radial probability density function must be employed while calculating radial nodes.
How to find radial nodes: Radial nodes formula
Radial nodes can be calculated using the following formula:
Number of radial nodes count = n-l-1 = n-(l+1)
Where n denotes the principal quantum number and l denotes the azimuthal quantum number.
Radial nodes in 2p
The value of the principal quantum number (n) in the 2p orbital is 2 and the value of the azimuthal quantum number (l) is 1.
Radial nodes in 2p = n-l-1 = 2-1-1 = 0
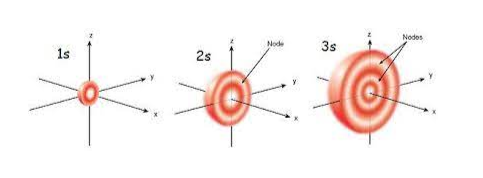
Radial nodes in 3s
In the 3s orbital, the primary quantum number (n) equals 3 and the azimuthal quantum number (l) equals 0.
Radial nodes in 3s = n – l -1 = 3 – 0 – 1 = 2
Radial nodes in 4d orbital
In 4d orbital, the value of principal quantum number (n) is 4 and the value of Azimuthal quantum number (l) is 2
Number of Radial nodes in 4d orbital = n- l -1 = 4-2-1 = 1
Radial nodes and Angular nodes: Comparison
Count of Electrons
Radial Nodes: These nodes often have spherical surfaces with a zero likelihood of finding an electron.
Angular Nodes: Angular nodes are normally flat planes (or cones) with a 0% probability of containing an electron.
Based on Shape
Radial nodes are discovered to be spherical.
Angular Nodes: Angular nodes are planar or cone-shaped.
Through Characteristic Properties
Radial Nodes: Radial nodes have fixed radii.
Angular Nodes: These nodes have set angles.
Depending on the number of nodes
Number of radial nodes: The primary quantum number can be used to calculate the number of radial nodes in an atom.
Angular Nodes: The number of angular nodes in an atom is often computed using angular momentum quantum number.
Also Check Relevant Topics like:
Angular nodes formula
The angular node values are not determined by the value of the primary quantum number. It is solely determined by the value of the azimuthal quantum number.
l = number of angular nodes
Where l denotes the azimuthal quantum number
Total number of nodes = no of radial nodes plus angular nodes = (n-l-1) = (n-1)
As a result, the total number of nodes equals (n-1).
Frequently Asked Questions on Radial nodes
What is radial node?
Radial nodes are spherical surface regions with a 0% probability of finding an electron.
How to calculate radial node?
The following simple equation can be used to calculate the number of radial nodes. No of Radial Nodes = n - 1 - l The 'n' represents the total number of nodes present. The total number of nodes is n-1. Using the total number of nodes, we can calculate the number of radial nodes. The number of radial nodes is n-l-1
How many no of radial nodes are present in 4d orbital?
The number of radial nodes relates to the principal quantum number, n. Overall, the nd orbitals have (n - 3) radial nodes. As a result, the 4d-orbitals each have (4 - 3) = 1 radial node.
How many radial nodes are present in 3p orbital?
one radial node The np orbital has (n - 2) radial nodes in general. As a result, the 3p-orbital has (3 - 2) = 1 radial nodes.
What are radial nodes also called?
A radial node is also known as a nodal area. A radial node is a spherical surface with a zero probability of finding an electron. The number of radial nodes grows in proportion to the principle quantum number (n). An angular node is also known as a nodal plane.









