Table of Contents
A cube table is a tabular representation of the cubes of numbers, where each number is raised to the power of 3. It serves as a quick reference tool for finding cube values and understanding number patterns.
The cube table typically lists numbers in ascending order, starting from 1 and extending up to a specified range. For each number, its cube is calculated by multiplying the number by itself twice. The cube value is then displayed in the table, allowing for easy access to the cubes of different numbers.
Also Check: Velocity
Importance of Cube Table
- Easy Calculation: A cube table allows for rapid calculation of cube values without the need for repeated multiplication.
- Numerical Patterns: The table helps identify patterns and relationships among cube values, such as perfect cubes and the increasing differences between consecutive cubes.
- Computational Efficiency: By referring to the cube table, one can perform calculations involving cubes more efficiently and accurately.
- Mathematical Applications: Cube tables are fundamental in algebra, geometry, and physics for solving problems related to volume, three-dimensional shapes, and equations involving cubes.
- Learning Aid: Cube tables aid in the memorization of cube values and support the development of mathematical fluency.
Cube Table 1 to 30
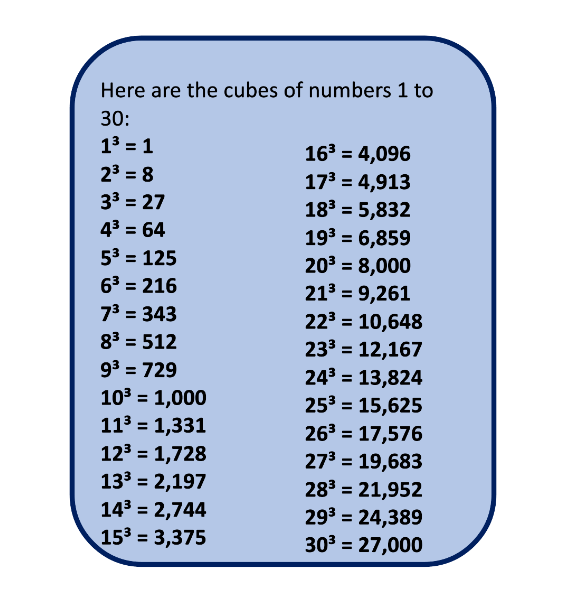
- The cube table of 1 to 30 displays the cube values of each number from 1 to 30.
- It allows for quick identification of the cubes of specific numbers, facilitating calculations and problem-solving.
- The table reveals the patterns present in the cube values, such as the increasing sequence of cubes and the concept of perfect cubes.
- It serves as a reference guide for students, professionals, and enthusiasts working with cubes in various disciplines.
- The cube table provides a concise and organized overview of the cube values, aiding in learning, visualization, and analysis of cubes within the specified range.
Cubes 1 to 30 – Even Numbers
| 23 = 8 | 43 = 64 |
| 63 = 216 | 83 = 512 |
| 103 = 1000 | 123 = 1728 |
| 143 = 2744 | 163 = 4096 |
| 183 = 5832 | 203 = 8000 |
| 223 = 10648 | 243 = 13824 |
| 263 = 17576 | 283 = 21952 |
| 303 = 27000 |
Cube 1 to 30 – Odd Numbers
| 13 = 1 | 33 = 27 |
| 53 = 125 | 73 = 343 |
| 93 = 729 | 113 = 1331 |
| 133 = 2197 | 153 = 3375 |
| 173 = 4913 | 193 = 6859 |
| 213 = 9261 | 233 = 12167 |
| 253 = 15625 | 273 = 19683 |
| 293 = 24389 |
Conclusion
In conclusion, a cube table is a useful tool that displays the cubes of numbers in a structured format. It serves as a reference for quickly finding cube values, aids in understanding number patterns, and supports mathematical calculations. Whether in educational settings or practical applications, cube tables are valuable resources for enhancing mathematical skills and problem-solving abilities.
Solved Examples on Cubes 1 to 30
Example 1: Find the sum of the cubes of the numbers 1 to 10.
Solution:
Cube of 1 = 1³ = 1
Cube of 2 = 2³ = 8
Cube of 3 = 3³ = 27
Cube of 4 = 4³ = 64
Cube of 5 = 5³ = 125
Cube of 6 = 6³ = 216
Cube of 7 = 7³ = 343
Cube of 8 = 8³ = 512
Cube of 9 = 9³ = 729
Cube of 10 = 10³ = 1000
Sum of the cubes = 1 + 8 + 27 + 64 + 125 + 216 + 343 + 512 + 729 + 1000 = 3025
Therefore, the sum of the cubes of the numbers 1 to 10 is 3025.
Example 2: Find the difference between the cube of 20 and the cube of 19.
Solution:
Cube of 20 = 20³ = 8000 Cube of 19 = 19³ = 6859
Difference = 8000 – 6859 = 1141
Therefore, the difference between the cube of 20 and the cube of 19 is 1141.
Example 3: Determine the cube root of 27,000.
Solution:
Cube root of 27,000 = ∛27,000 = 30
Therefore, the cube root of 27,000 is 30.
Related Links:
| Cube Root Formula | Cube Root List 1 to 100 |
| Cubes and Cube Roots | Cube Root of 2 |
| Perfect Cube Of Numbers | Cubes From 1 to 50 |
FAQs on Cubes 1 to 30
What is the cube number 1 to 30?
The cube values of numbers 1 to 30 are as follows:
1³ = 1
2³ = 8
3³ = 27
4³ = 64
5³ = 125
6³ = 216
7³ = 343
8³ = 512
9³ = 729
10³ = 1000
11³ = 1331
12³ = 1728
13³ = 2197
14³ = 2744
15³ = 3375
16³ = 4096
17³ = 4913
18³ = 5832
19³ = 6859
20³ = 8000
21³ = 9261
22³ = 10648
23³ = 12167
24³ = 13824
25³ = 15625
26³ = 17576
27³ = 19683
28³ = 21952
29³ = 24389
30³ = 27000
How to memorize cubes 1 to 30?
To memorize the cubes from 1 to 30, you can use mnemonic techniques, such as creating associations or visualizations for each cube's value. For example, you can associate 8³ (512) with 5-1-2 by visualizing a clock with the hands pointing at 5, 1, and 2. Practice repetition, flashcards, and regular review to reinforce memorization.
What are cube values?
The cube values represent the result of multiplying a number by itself twice or raising it to the power of 3. For example, 4³ means 4 multiplied by itself twice, resulting in 64.
What is the formula for the cube trick?
The formula for the cube trick is (a + b)³ = a³ + 3a²b + 3ab² + b³, which is known as the binomial cube formula. It allows you to expand the cube of a binomial expression.
What is cube problem solving?
Cube problem-solving refers to solving mathematical problems involving cubes, such as finding the sum of cubes, differences between cube values, cube roots, and applying cube-related concepts in various mathematical contexts.
What is the cube rule in math?
The cube rule in math refers to the principle that the cube of a sum is not equal to the sum of cubes. In other words, (a + b)³ ≠ a³ + b³. The cube rule is an important concept to understand when working with cubed expressions.
Is 64 a perfect cube?
Yes, 64 is a perfect cube. It is equal to 4³, which means 4 multiplied by itself twice, resulting in 64.
What does 4 cubed means?
4 cubed, written as 4³, means 4 raised to the power of 3, which is equal to 4 × 4 × 4 = 64.