Table of Contents
Introduction:
Gibbs free energy, or simply Gibbs energy, is an important thermodynamic state function that describes the energy available for work in a thermodynamic system. It is the difference between the enthalpy and the product of the temperature and the entropy of the system. It is a measure of the potential for a chemical reaction to occur, with a negative value indicating a spontaneous reaction.
Gibbs energy change is a measure of the change in Gibbs free energy from the start to the end of a chemical reaction. It is a function of the enthalpy and entropy changes that accompany the reaction. It can also be used to assess the spontaneity of a reaction. A positive Gibbs energy change indicates that the reaction is non-spontaneous and requires an input of energy to proceed. A negative Gibbs energy change indicates that the reaction is spontaneous and releases energy.
Gibbs energy change can be used to calculate the equilibrium constants of a reaction and to determine the amount of energy released or absorbed by a reaction. It can also be used to evaluate reaction mechanisms and to determine the thermodynamic feasibility of a reaction.
Gibbs energy change is an important concept in thermodynamics and is used to understand and predict the behavior of thermodynamic systems. It is also an important tool for scientists in the study of chemical systems and in the development of new materials.
Overview
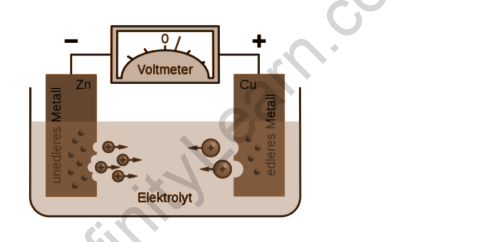
Electrochemical cells are devices that use a chemical reaction to generate electricity. It is essentially a device that converts chemical energy into electrical energy. An electrochemical cell requires a chemical reaction that involves the exchange of electrons to function. These kinds of reactions are known as redox reactions.
The voltage of a cell defines it. Without considering the cell size, a specific cell type generates the same voltage. Given ideal operating conditions, the only thing that depends on cell voltage is the chemical composition of the cell.
Usually, the cell voltage will deviate from this ideal value due to various factors such as temperature differences, concentration changes, and so on. Generally, the Nernst equation can be used to calculate the EMF value of a given cell if the cell’s standard cell potential is known.
As per the second law of thermodynamics, there is a general natural tendency for systems reacting at standard conditions for temperature and pressure (or any other fixed temperature and pressure) to obtain a minimum of the Gibbs free energy. The phrase “free” was traditionally included in “Gibbs free energy” to mean “available in the form of useful work.” When we add the qualification that it is the energy available for non-pressure-volume work, the characterization becomes more precise. (For systems at a constant temperature, an analogous but slightly different meaning of “free” applies in conjunction with the Helmholtz free energy.)
Emf of a cell
In general, the electromotive force or emf of a cell is the maximum potential difference between two cell electrodes. This is also the net voltage between the reaction’s oxidation and reduction halves. We can say that a cell’s EMF is primarily used to determine whether or not an electrochemical cell is galvanic.
When no current is drawn from a cell, the voltage or electric potential difference across its terminals, now, the emf is the net sum of the electric potential differences produced by charge separation (electrons or ions) at each phase boundary (or interface) in the cell. Each potential difference’s magnitude is determined by the chemical nature of the two contacting phases. As a result, some electrons will have moved from the metal with a higher free energy of electrons to the metal with a lower free energy of electrons at the interface between two different metals. The subsequent charge separation will generate a potential difference, similar to how charge separation generates a voltage across a capacitor; this exactly opposes further electron flow at equilibrium. Potential differences can also be produced when electrons partition across a metal solution or metal solid interface or when ions partition across a solution| membrane solution interface.
Gibbs free energy
Gibbs free energy, also identified as the Gibbs function, Gibb’s energy, or free enthalpy, is a quantity used to calculate the maximum amount of work done in a thermodynamic system with constant temperature and pressure. The symbol ‘G’ represents Gibbs’s free energy, and it is usually measured in Joules or Kilojoules. The maximum amount of work extracted from a closed system is defined as Gibbs’s free energy.
One such property was discovered in 1876 by American scientist Josiah Willard Gibbs while conducting experiments to predict the behavior of systems when combined or whether a process could occur simultaneously and spontaneously. Gibbs’s free energy was previously referred to as “available energy.” It can be represented as the amount of useful energy present in a thermodynamic system that can be used to do some work.
According to Gibbs, the initial state of the body is such that “the body can be made to pass from it to states of dissipated energy by reversible processes.” He completely engaged his views on chemical-free energy in his magnum opus On the Equilibrium of Heterogeneous Substances, a graphical analysis of multi-phase chemical systems, published in 1876.
Standard Gibb’s free energy formula
Gibb’s free energy is equivalent to the system’s enthalpy minus the product of temperature and entropy. The equation is as follows:
G = H – TS
Here,
- G = Gibbs free energy
- H = enthalpy
- T = temperature
- S = entropy
OR
or more completely as;
G = U + PV – TS
Here,
- U = the internal energy (SI unit: joule)
- P = pressure (SI unit: pascal)
- V = volume (SI unit: m3)
- T = temperature (SI unit: kelvin)
- S = entropy (SI unit: joule/kelvin)
The standard Gibbs free energy of formation of a compound is the change in Gibbs free energy that is followed by the formation of 1 mole of that substance from its component element available at their standard states or the most stable form of the element at 25 °C and 100 kPa. It has the symbol ΔfG˚.
Because there is no chance involved, all elements in their standard states (diatomic oxygen gas, graphite, etc.) have standard Gibbs free energy change of formation equal to zero.
ΔfG = ΔfG˚ + RT ln Qf,
Here Qf is the reaction quotient.
At equilibrium, ΔfG = 0 and Qf = K, so the equation will be,
ΔfG˚ = −RT ln K,
Here, K is said to be the equilibrium constant.
Relation between Gibbs energy change and EMF of a cell
As in the case of galvanic cells, the Gibbs energy change is proportional to the cell’s electrical work.
ΔG = -nFE(cell)
Here,
- n = no. of moles of electrons involved
- F = the Faraday constant
- E = emf of the cell
- F=1 Faraday =96500 coulombs
When reactants and products are in their standard states,
ΔG°= –nFE°cell
Full Forms:
- ATS Full Form
- BFSI Full Form
- EVM Full Form
- ELISA Full Form
- SPM Full Form
- RDX Full Form
- SENSEX Full Form
- FHR Full Form
- DNS Full Form
Crack NEET with Result-Oriented Learning Program from Infinity Learn.
FAQs
Question 1: What is cell potential and emf?
Answer 1: The cell potential is the potential difference between the two electrodes of a galvanic cell, and it is measured in volts,, whereas the emf is the difference among the electrode potentials of the cathode and anode.
Question 2: What is the use of emf?
Answer 2: Magnetic resonance imaging (MRI), radiofrequency ablation (RFA) used in cardiology and tumor therapy, and localized dielectric heating (short wave diathermy) used in physiotherapy are the three main EMF applications in medicine.
Question 3: What is Gibb’s energy in electrochemistry?
Answer 3: The maximum amount of work that can be extracted from a closed system is characterized as Gibbs free energy.
Infinity Learn App
Now you can find answers to all your subject queries & prepare for your Exams on our Free Learning App – Infinity Learn.
