Table of Contents
Introduction to Linear Function
Linear functions are fundamental ideas in mathematics and have applications in physics, economics, and engineering. When plotted on a Cartesian plane, these functions illustrate a simple connection between two variables, resulting in a straight-line graph. Understanding linear functions is essential for understanding more complicated mathematical ideas and applications..
What is Linear function
A linear function is a mathematical function that has the formula f(x) = mx + b, where ‘m’ and ‘b’ are constants and ‘x’ is the independent variable. The variable ‘m’ denotes the line’s slope, while ‘b’ represents the y-intercept, or the point at which the line crosses the y-axis. The slope controls the function’s rate of change, whereas the y-intercept provides the function’s value at x = 0..
What is non-linear function
Non-linear functions, unlike linear functions, do not have a constant rate of change. A non-linear function’s graph does not form a straight line in the Cartesian plane. It might instead have curves, parabolas, exponential growth, or other complicated forms. Non-linear functions have more complicated shapes, and their rates of change vary throughout the curve.
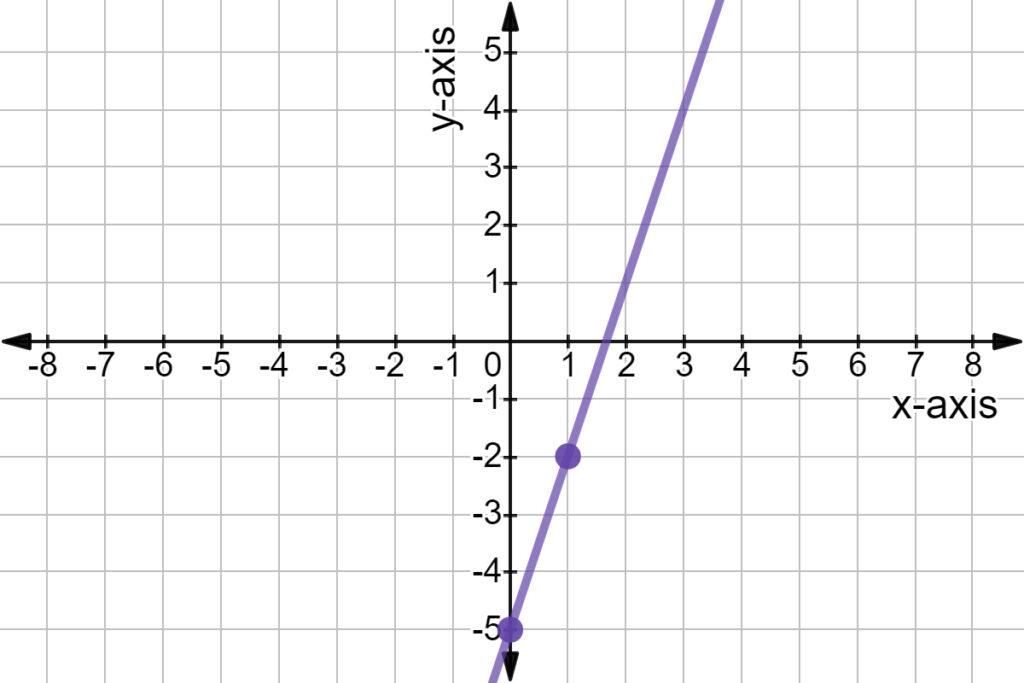
Graph of linear function
A linear function’s graph is always a straight line. The slope’m’ specifies the steepness or direction of the line, whereas the y-intercept ‘b’ specifies where the line intersects the y-axis. Positive slopes cause the line to climb from left to right, whereas negative slopes lead it to descend from left to right. If the slope is zero, the line becomes horizontal; otherwise, the line becomes vertical.
Linear function formula
A linear function’s general formula is f(x) = mx + b, where ‘f(x)’ is the dependent variable, ‘x’ is the independent variable,’m’ is the slope, and ‘b’ is the y-intercept. This formula allows us to get the function value for any given ‘x’ value and aids in visualising the linear function.
Characteristics of linear function
Linear functions have numerous important properties:
-
- Constant Rate of Change: Because the slope ‘m’ remains constant throughout the function, the rate of change is constant.
- Straight-Line Graph: A straight line is formed when a graph is drawn on a Cartesian plane.
- A linear function has a single unique solution for each value of ‘x.’
- Changes in the independent variable ‘x’ result in a proportionate change in the dependent variable ‘f(x).’
Linear function examples
Example 1: Consider the linear function f(x) = 2x + 3. Find the value of ‘f(x)’ when x = 5.
Solution: Linear Function f(x) = 2x + 3. To generate the output, this function takes an input value, multiplies it by 2, and then adds 3.
- f(5) = 2(5) + 3
- f(5) = 10 + 3
- f(5) = 13L
Therefore, the value of f(5) is 131
Example 2: The cost of producing x units of a product is given by the linear function C(x) = 50x + 200. Find the cost when x = 10.
Solution:
- C(10) = 50(10) + 200
- C(10) = 500 + 200
- C(10) = 700
Conclusion
Linear functions, which express a straight-line relationship between two variables, are essential notions in mathematics. Understanding their properties, graphs, and formulae lets us to analyse and make educated judgements about many real-world challenges. Furthermore, distinguishing between linear and non-linear functions is critical for understanding more complex mathematical ideas and applications. With a strong understanding of linear functions, one may investigate increasingly sophisticated mathematical models and their real-world applications.
Frequently asked questions on Linear Function
What does a negative slope in a linear function indicate?
A negative slope shows that the slope of the line reduces as the value of 'x' rises. On the graph, the line slants downhill from left to right.
What is linear function with example?
linear function is a mathematical function that describes the connection between two variables in a straight line. It has the formula f(x) = mx + b, where 'm' is the slope reflecting the rate of change and 'b' is the y-intercept, or point at which the line contacts the y-axis. Consider a car rental business that costs a $30 fixed fee plus $0.25 every mile travelled. The total cost 'C' is calculated as C(x) = 0.25x + 30, where 'x' is the number of miles travelled. This linear function calculates the total cost of automobile rental based on the distance travelled.
What defines a linear function?
It has the formula f(x) = mx + b, where ‘m' indicates the slope, which determines the rate of change, and 'b' is the y-intercept, which is the point where the line contacts the y-axis.
What is a formula for linear function?
A linear function has the formula f(x) = mx + b, where 'f(x)' represents the dependent variable, 'x' represents the independent variable,'m' represents the slope (rate of change), and 'b' represents the y-intercept (value at x = 0). A straight-line relationship between two variables on a Cartesian plane is defined by this formula.
How are functions being linear?
Linear functions are those that have a straight-line connection between two variables on a Cartesian plane. The linearity is determined by the formula f(x) = mx + b, where'm' represents the constant slope and 'b' is the constant y-intercept. Linear functions are distinguished by the simplicity of a straight-line connection.
What are 2 examples of linear?
Here are two instances of linear functions: Cost function: C(x) = 0.5x + 100, where 'x' is the quantity produced and the cost per item is $0.5 with a constant cost of $100. Temperature conversion: F(x) = 1.8x + 32, where 'x' is the Celsius temperature, and 'F(x)' is the Fahrenheit equivalent using the linear conversion formula.
What is linear function in LPP?
A linear function in Linear Programming Problems (LPP) refers to the objective function or the constraints with variables of degree one. The goal is to optimise this function while keeping linear restrictions in mind, which are expressed as linear inequalities or equations. Linear functions in LPP serve an important role in solving real-world issues such as resource allocation and production planning.
What is linear and non-linear examples
Linear Example: The cost of renting a car for $30 plus $0.25 per mile is a linear function, C(x) = 0.25x + 30, where 'x' is the number of miles travelled. Non-linear Example: Bacterial population increase, in which the number of bacteria doubles every hour, is a non-linear function due to its exponential development.
What are the types of linear?
There are two kinds of linear functions: Linear functions are represented by the equation f(x) = mx + b, which has a constant rate of change'm' and a y-intercept 'b.' A straight line is depicted on the graph. Affine Functions: Affine functions are similar to linear functions but may have an extra constant component. They are denoted by the equation f(x) = mx + b + c, where 'c' is a constant. The graph, too, is a straight line.
Is a table linear function?
No, a table is not a linear function in and of itself. A table may include data points from a linear function, but it does not represent the function as a whole. When displayed on a Cartesian plane, a linear function is a mathematical connection between variables that is often expressed by an equation or formula (f(x) = mx + b).
hat is non-linear in math?
In mathematics, a non-linear relationship or function is one that does not form a straight line when plotted on a Cartesian plane. Non-linear functions do not vary at a constant pace and can take on a variety of forms, such as curves, parabolas, or exponential development. These functions are more complicated than linear functions, and they frequently include higher-order equations.









