Reflection of light

Introduction

Almost every day we see ourselves in the mirror. Why is it that we can ourselves in a mirror of glass but not in a wall? Let us try to answer it!

When a ray of light falls on a smooth polished surface and if a part or whole of it bounces back, we say light has reflected from the surface.

The ray that falls on the surface is called incident ray and the re-bounced ray is called reflected ray.

Light obeys some laws as it undergoes reflection from a surface. These are called laws of reflection.

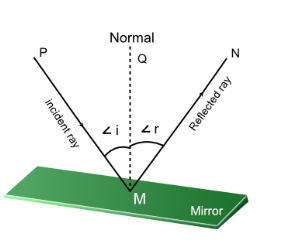
1st law of reflection: The incident ray, the normal and the reflected ray, at the point of incidence, lie in the same plane.

In the figure shown, PM is the incident ray, MQ is the outward normal (perpendicular) to the reflecting surface. These two lines form a plane called the plane of incidence. As per 1st law of reflection, the direction of reflected ray, MN , is such that PM, MQ, MN all lie in the plane of incidence.

 

 

 

 

 

2nd law of reflection: Angle of incidence is equal to the angle of reflection. Note that both angles are measured from the normal, and not from the surface. ∠i =∠r.

Problem 1

If an incident ray of light makes an angle of 60^o with the surface, then what is the angle of incidence?

Solution

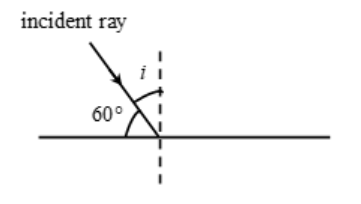
Angle of incidence, i = 90^o– 60^o = 30^o

Problem 2

A ray of light falls on a smooth surface and undergoes reflection. If angle of incidence is 60^o, then what is the angle between the direction of incident ray and that of the reflected ray?

Solution

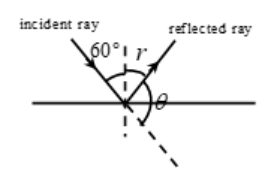
By laws of reflection, r =i = 60^o

Angle between the direction of incident ray and that of the reflected ray

θ + 180^o – (i + r) = 180^o – 2i x 60^o = 60^o

Problem 3

In the adjacent diagram, a ray of light is incident at the origin . If a mirror is kept along , in which quadrant will the reflected ray lie?

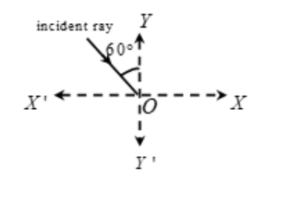
Solution

The light will reflect into the third quadrant, as shown.

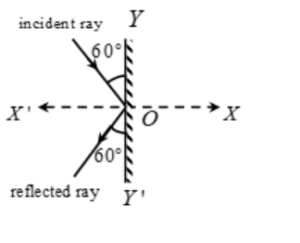
Problem 4

In the given diagram, if the mirror is rotated by an angle of clockwise about a perpendicular axis passing through point , what will be the new angle of reflection?

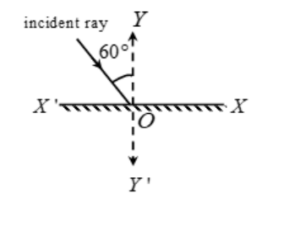
Solution

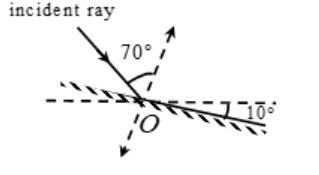
If the mirror is rotated by an angle of 10^o clockwise about a perpendicular axis passing through point O, the normal to mirror also rotates clockwise by 10^o.

Angle between incident ray and new normal = 60^o + 10 = 70^o

Hence, new angle of incidence .

By laws of reflection, new angle of reflection = new angle of incidence

Physics Articles Physics Formulas Vernier Calipers Surface Tension

Types of reflections

• Regular reflection is also known as specular reflection
• Diffused reflection

Regular reflection

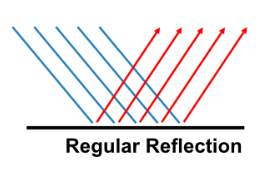
Here reflecting surface is made a lot smoother by coating it with metals like mercury or with powders. This makes very less variations in angles of incidence for different incident rays present in the incident beam. Almost all rays get reflected along the same direction giving greater intensity of light. This is what makes reflection from mirrors show sharp images of us.

Diffused reflection

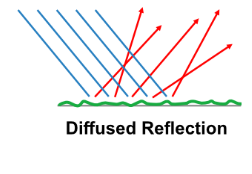
Reflections from rough surfaces fall under this category. As is clear from figure, many different incident rays are reflected along different directions. Hence intensity of reflected light in a specific direction is much less. This is the reason why normally we do not see ourselves in the wall or doors.

 

 

 

 

Frequently Asked Questions

What are the laws of reflection?

First law of reflection: Incident ray, normal and the reflected ray all lie in the same plane. Second law of reflection: Angle of incidence is equal to angle of reflection.

Why do we not see ourselves in walls but see ourselves in mirrors?

In mirrors, reflecting surface is made a lot smoother by coating it with metals like mercury or with powders. This makes very less variations in angles of incidence for different incident rays present in the incident beam. Almost all rays get reflected along the same direction giving greater intensity of light. This is what makes reflection from mirrors show sharp images of us. In walls, the variations in angles of incidence is quite high. So rays are practically scattered in many directions, giving very less intensity in a specific direction.

Sharp images are produced by which type of reflection?

Regular reflection results in sharp images.