Lines and Angles Class 7 Notes: Chapter 5

Welcome to the world of geometry, where lines and angles come together to form the foundation of shapes and structures. In Class 7, you will learn about different types of lines and angles, their properties, and how they are used to create various shapes.

To help you master this chapter, we have prepared a set of Lines and Angles Class 7 Extra Questions Maths Chapter 5 that will challenge your understanding and reinforce your learning. These extra questions cover a range of topics, from identifying different types of lines and angles to solving problems involving complementary and supplementary angles.

With these extra questions, you will be well-prepared to tackle the NCERT Solutions for Class 7 Maths Chapter 5 and excel in your CBSE syllabus. Download the Lines and Angles Class 7 Extra Questions Maths Chapter 5 PDF to get started and stay ahead in your Class 7 Maths syllabus.

Lines and Angles Class 7 Extra Questions Maths Chapter 5 PDF

Get ready to master the world of geometry with our ‘Lines and Angles Class 7 Extra Questions Maths Chapter 5 PDF‘. This comprehensive guide covers various types of lines and angles, their properties, and problem-solving techniques. Stay ahead in your Class 7 Maths syllabus with our extra questions and NCERT Solutions class 7 for Chapter 5.

Lines and Angles Class 7 Extra Questions with Answers PDF Download

Lines and Angles Class 7 Extra Questions with Answers and Solutions

Question 1. Find the angles which is \(\frac { 1 }{ 5 }\) of its complement.

Solution:

Let the required angle be x°

its complement = (90 – x)°

As per condition, we get

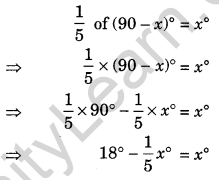
Question 2. Find the angles which is \(\frac { 2 }{ 3 }\) of its supplement.

Solution:

Let the required angle be x°.

its supplement = (180 – x)°

As per the condition, we get

\(\frac { 2 }{ 3 }\) of (180 – x)° = x°

Question 3. Find the value of x in the given figure.

Solution: ∠POR + ∠QOR = 180° (Angles of linear pair)

⇒ (2x + 60°) + (3x – 40)° = 180°

⇒ 2x + 60 + 3x – 40 = 180°

⇒ 5x + 20 = 180°

⇒ 5x = 180 – 20 = 160

⇒ x = 32

Thus, the value of x = 32.

Question 4. In the given figure, find the value of y.

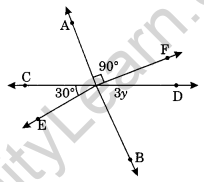
Solution:

Let the angle opposite to 90° be z.

z = 90° (Vertically opposite angle)

3y + z + 30° = 180° (Sum of adjacent angles on a straight line)

⇒ 3y + 90° + 30° = 180°

⇒ 3y + 120° = 180°

⇒ 3y = 180° – 120° = 60°

⇒ y = 20°

Thus the value of y = 20°.

Question 5. Find the supplements of each of the following:

(i) 30°

(ii) 79°

(iii) 179°

(iv) x°

(v) \(\frac { 2 }{ 5 }\) of right angle

Solution:

(i) Supplement of 30° = 180° – 30° = 150°

(ii) Supplement of 79° = 180° – 79° = 101°

(iii) Supplement of 179° = 180° – 179° = 1°

(iv) Supplement of x° = (180 – x)°

(v) Supplement of \(\frac { 2 }{ 5 }\) of right angle

= 180° – \(\frac { 2 }{ 5 }\) × 90° = 180° – 36° = 144°

Question 6. If the angles (4x + 4)° and (6x – 4)° are the supplementary angles, find the value of x.

Solution:

(4x + 4)° + (6x – 4)° = 180° (∵ Sum of the supplementary angle is 180°)

⇒ 4x + 4 + 6x – 4 = 180°

⇒ 10x = 180°

⇒ x = 18°

Thus, x = 18°

Question 7. Find the value of x.

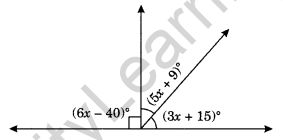
Solution:

(6x – 40)° + (5x + 9)° + (3x + 15) ° = 180° (∵ Sum of adjacent angles on straight line)

⇒ 6x – 40 + 5x + 9 + 3x + 15 = 180°

⇒ 14x – 16 = 180°

⇒ 14x = 180 + 16 = 196

⇒ x = 14

Thus, x = 14

Question 8. Find the value of y.

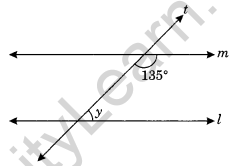
Solution:

l || m, and t is a transversal.

y + 135° = 180° (Sum of interior angles on the same side of transversal is 180°)

⇒ y = 180° – 135° = 45°

Thus, y = 45°

Enroll Now to Foundation Course for Class 7

Lines and Angles Class 7 Extra Questions Short Answer Type

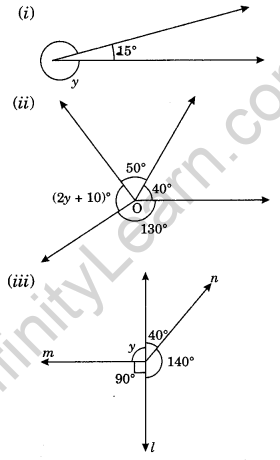
Question 9. Find the value ofy in the following figures:

Solution:

(i) y + 15° = 360° (Sum of complete angles round at a point)

⇒ y = 360° – 15° = 345°

Thus, y = 345°

(ii) (2y + 10)° + 50° + 40° + 130° = 360° (Sum of angles round at a point)

⇒ 2y + 10 + 220 = 360

⇒ 2y + 230 = 360

⇒ 2y = 360 – 230

⇒ 2y = 130

⇒ y = 65

Thus, y = 65°

(iii) y + 90° = 180° (Angles of linear pair)

⇒ y = 180° – 90° = 90°

[40° + 140° = 180°, which shows that l is a straight line]

Question 10. In the following figures, find the lettered angles.

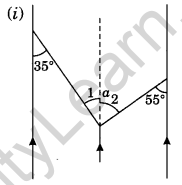
Solution:

(i) Let a be represented by ∠1 and ∠2

∠a = ∠1 + ∠2

∠1 = 35° (Alternate interior angles)

∠2 = 55° (Alternate interior angles)

∠1 + ∠2 = 35° + 55°

∠a = 90°

Thus, ∠a = 90°

Question 11. In the given figure, prove that AB || CD.

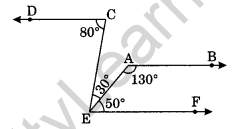
Solution:

∠CEF = 30° + 50° = 80°

∠DCE = 80° (Given)

∠CEF = ∠DCE

But these are alternate interior angle.

CD || EF ……(i)

Now ∠EAB = 130° (Given)

∠AEF = 50° (Given)

∠EAB + ∠AEF = 130° + 50° = 180°

But these are co-interior angles.

AB || EF …(ii)

From eq. (i) and (ii), we get

AB || CD || EF

Hence, AB || CD

Co-interior angles/Allied angles: Sum of interior angles on the same side of transversal is 180°.

Question 12. In the given figure l || m. Find the values of a, b and c.

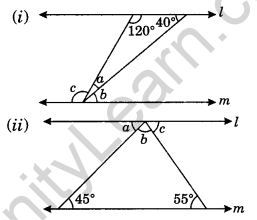
Solution:

(i) We have l || m

∠b = 40° (Alternate interior angles)

∠c = 120° (Alternate interior angles)

∠a + ∠b + ∠c = 180° (Sum of adjacent angles on straight angle)

⇒ ∠a + 40° + 120° = 180°

⇒ ∠a + 160° = 180°

⇒ ∠a = 180° – 160° = 20°

Thus, ∠a = 20°, ∠b = 40° and ∠c = 120°.

(ii) We have l || m

∠a = 45° (Alternate interior angles)

∠c = 55° (Alternate interior angles)

∠a + ∠b + ∠c = 180° (Sum of adjacent angles on straight line)

⇒ 45 + ∠b + 55 = 180°

⇒ ∠b + 100 = 180°

⇒ ∠b = 180° – 100°

⇒ ∠b = 80°

Question 13. In the adjoining figure if x : y : z = 2 : 3 : 4, then find the value of z.

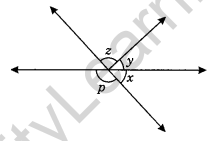
Solution:

Let x = 2s°

y = 3s°

and z = 4s°

∠x + ∠y + ∠z = 180° (Sum of adjacent angles on straight line)

2s° + 3s° + 4s° = 180°

⇒ 9s° = 180°

⇒ s° = 20°

Thus x = 2 × 20° = 40°, y = 3 × 20° = 60° and z = 4 × 20° = 80°

Question 14. In the following figure, find the value of ∠BOC, if points A, O and B are collinear. (NCERT Exemplar)

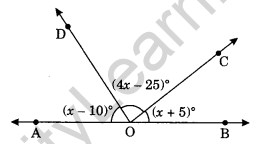
Solution:

We have A, O and B are collinear.

∠AOD + ∠DOC + ∠COB = 180° (Sum of adjacent angles on straight line)

(x – 10)° + (4x – 25)° + (x + 5)° = 180°

⇒ x – 10 + 4x – 25 + x + 5 = 180°

⇒ 6x – 10 – 25 + 5 = 180°

⇒ 6x – 30 = 180°

⇒ 6x = 180 + 30 = 210

⇒ x = 35

So, ∠BOC = (x + 5)° = (35 + 5)° = 40°

Question 15. In given figure, PQ, RS and UT are parallel lines.

(i) If c = 57° and a = \(\frac { c }{ 3 }\), find the value of d.

(ii) If c = 75° and a = \(\frac { 2 }{ 5 }\)c , find b.

 

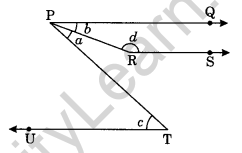
Solution:

(i) We have ∠c = 57° and ∠a = \(\frac { \angle c }{ 3 }\)

∠a = \(\frac { 57 }{ 3 }\) = 19°

PQ || UT (given)

∠a + ∠b = ∠c (Alternate interior angles)

19° + ∠b = 57°

∠b = 57° – 19° = 38°

PQ || RS (given)

∠b + ∠d = 180° (Co-interior angles)

38° + ∠d = 180°

∠d = 180° – 38° = 142°

Thus, ∠d = 142°

(ii) We have ∠c = 75° and ∠a = \(\frac { 2 }{ 5 }\) ∠c

∠a = \(\frac { 2 }{ 5 }\) × 75° = 30°

PQ || UT (given)

∠a + ∠b = ∠c

30° + ∠b = 75°

∠b = 75° – 30° = 45°

Thus, ∠b = 45°

Question 16. What is the sum of the measures of the angles of a triangle?

Solution: The sum of the measures of the angles of a triangle is 180 degrees.

Question 17. What is the sum of the measures of the angles of a quadrilateral?

Solution: The sum of the measures of the angles of a quadrilateral is 360 degrees.

Question 18. What is the measure of the angle formed by two intersecting lines?

Solution: The measure of the angle formed by two intersecting lines is 90 degrees, which is a right angle.

Question 19. What is the measure of the angle formed by two lines that are parallel and cut by a transversal?

Solution: The measure of the angle formed by two lines that are parallel and cut by a transversal is 60 degrees, which is an exterior angle.

Question 20. What is the measure of the angle formed by two lines that are perpendicular and cut by a transversal?

Solution: The measure of the angle formed by two lines that are perpendicular and cut by a transversal is 90 degrees, which is an exterior angle.

Question 21. What is the measure of the angle formed by two lines that are parallel and cut by a transversal, and one of the lines is perpendicular to the transversal?

Solution: The measure of the angle formed by two lines that are parallel and cut by a transversal, and one of the lines is perpendicular to the transversal, is 90 degrees, which is an exterior angle.

Question 22. What is the measure of the angle formed by two lines that are perpendicular and cut by a transversal, and one of the lines is parallel to the transversal?

Solution: The measure of the angle formed by two lines that are perpendicular and cut by a transversal, and one of the lines is parallel to the transversal, is 90 degrees, which is an exterior angle.

Question 23. What is the measure of the angle formed by two lines that are parallel and cut by a transversal, and both lines are perpendicular to the transversal?

Solution: The measure of the angle formed by two lines that are parallel and cut by a transversal, and both lines are perpendicular to the transversal, is 90 degrees, which is an exterior angle.

Question 24. What is the measure of the angle formed by two lines that are perpendicular and cut by a transversal, and both lines are parallel to the transversal?

Solution: The measure of the angle formed by two lines that are perpendicular and cut by a transversal, and both lines are parallel to the transversal, is 90 degrees, which is an exterior angle.

Question 25. What is the measure of the angle formed by two lines that are parallel and cut by a transversal, and one of the lines is perpendicular to the transversal, and the other line is parallel to the transversal?

Solution: The measure of the angle formed by two lines that are parallel and cut by a transversal, and one of the lines is perpendicular to the transversal, and the other line is parallel to the transversal, is 90 degrees, which is an exterior angle.

Question 26. What is the measure of the angle formed by two lines that are perpendicular and cut by a transversal, and one of the lines is perpendicular to the transversal, and the other line is parallel to the transversal?

Solution: The measure of the angle formed by two lines that are perpendicular and cut by a transversal, and one of the lines is perpendicular to the transversal, and the other line is parallel to the transversal, is 90 degrees, which is an exterior angle.

Lines and Angles Class 7 FAQs

What are lines in geometry?

Lines are straight, one-dimensional figures that extend infinitely in both directions. They have no endpoints.

What is the difference between a line and a line segment?

A line extends infinitely in both directions, while a line segment has two endpoints and a fixed length.

What are angles?

An angle is formed when two rays (sides of the angle) meet at a common endpoint (vertex). It measures the rotation between the two rays.

How do we measure angles?

Angles are measured in degrees (°) using a protractor. A full circle is 360°, a right angle is 90°, and a straight angle is 180°.

What are complementary angles?

Complementary angles are two angles that add up to 90°. For example, if one angle is 30°, the other must be 60°.

What are supplementary angles?

Supplementary angles are two angles that sum up to 180°. For example, if one angle is 110°, the other must be 70°.

What are vertical angles?

Vertical angles are the opposite angles formed when two lines intersect. They are always equal in measure.

What is a transversal?

A transversal is a line that crosses two or more other lines. It creates several angles, including corresponding, alternate interior, and alternate exterior angles.

What are corresponding angles?

Corresponding angles are formed when a transversal intersects two parallel lines. They are in the same position on each line and are equal in measure.

Why are angles important in real life?

Angles are crucial in various fields such as construction, art, engineering, and navigation, helping to create accurate designs and measurements.