Table of Contents
Introduction to Surface Area of a Pyramid Formula
The surface area of a pyramid is obtained by adding the area of all its faces. A pyramid is a three-dimensional shape whose base is a polygon and whose side faces (that are triangles) meet at a point which is called the apex (or) vertex. The perpendicular distance from the apex to the center of the base is called the altitude or height of the pyramid. The length of the perpendicular drawn from the apex to the base of a triangle (side face) is called the ‘slant height’. Let us learn more about the surface area of a pyramid along with its formula, and a few solved examples.
What is the Surface Area of Pyramid?
The surface area of a pyramid is a measure of the total area that is occupied by all its faces. Observe the pyramid given below to see all its faces and the other parts like the apex, the altitude, the slant height, and the base.
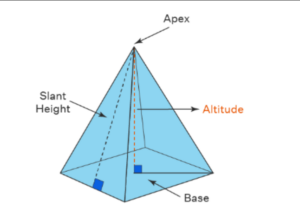
The surface area of a pyramid is the sum of areas of its faces and hence it is measured in square units such as m2, cm2, in2, ft2, etc. A pyramid has two types of surface areas, one is the Lateral Surface Area (LSA) and the other is the Total Surface Area (TSA).
- The Lateral Surface Area (LSA) of a pyramid = The sum of areas of the side faces (triangles) of the pyramid.
- The Total Surface Area (TSA) of a pyramid = LSA of pyramid + Base area
In general, the surface area of a pyramid without any specifications refers to the total surface area of the pyramid.
Surface Area of Pyramid Formula
The surface area of a pyramid can be calculated by finding the areas of each of its faces and adding them. If the pyramid is regular (i.e., a pyramid whose base is a regular polygon and whose altitude passes through the center of the base), there are some specific formulas to find the lateral surface area and total surface area.
Consider a regular pyramid whose base perimeter is ‘P’, the base area is ‘B’, and slant height (the height of each triangle) is ‘l’. Then,
- The Lateral Surface Area of pyramid (LSA) = (1/2) Pl
- The Total Surface Area of pyramid (TSA) = LSA + base area = (1/2) Pl + B
Proof of Surface Area of Pyramid Formula
The surface area of a pyramid involves the perimeter and slant height. Let us understand the formulas of LSA and TSA of a pyramid by taking a specific pyramid as an example. Let us consider a square pyramid whose base length is ‘a’ and whose slant height is ‘l’.
Then,
- The base area (area of square) of the pyramid is, B = a2
- The base perimeter (perimeter of square) of the pyramid is, P = 4a
- The area of each of the side faces (area of triangle) = (1/2) × base × height = (1/2) × (a) × l
Therefore, the sum of all side faces (sum of all 4 triangular faces) = 4 [(1/2) × (a) × l] = (1/2) × (4a) × l = (1/2) Pl. (Here, we replaced 4a with P which represents its perimeter.)
Hence, the Lateral Surface Area of the pyramid (LSA) = (1/2) Pl
We know that the Total Surface Area of a pyramid (TSA) is obtained by adding the base and lateral surface areas. Thus,
The total surface area of pyramid (TSA) = LSA + base area = (1/2) Pl + B
Using these two formulas, we can derive the surface area formulas of different types of pyramids.
Surface Area of Pyramid with Altitude
The surface area of a pyramid can be calculated if its altitude is given. Observe the figure given below which shows that the triangle formed by half the side length of the base (a/2), the slant height (l), and the altitude (h) is a right-angled triangle. Hence, we can apply the Pythagoras theorem and find out the slant height if the altitude and base length is given. Thus, l2 = h2 + (a/2)2
So, we can calculate the slant height using the formula, l2 = h2 + (a/2)2.
Now that we have the slant height, the base length, and the height, we can find the surface area of the pyramid using the formula,
Total surface area of pyramid (TSA) = LSA + base area = (1/2) Pl + B
Solved Examples on Surface Area of a Pyramid Formula
Example 1: Calculate the lateral surface area of a square pyramid if the side length of the base is 14 inches and the slant height of the pyramid is 20 inches.
Solution:
The side length of the base, a = 14 inches
Then, the perimeter of the base (square) is, P = 4a = 4(14) = 56 inches.
Slant height, l = 20 inches
The lateral surface area of a square pyramid is,
Lateral surface area (LSA) = (1/2) Pl
= (1/2) × (56) × 20
= 560 in2
Therefore, the lateral surface area of the given pyramid is 560 in2.
Example 2: State true or false.
a.) The surface area of any pyramid can be calculated by finding the areas of each of its faces and adding them.
b.) The total surface area (TSA) of a pyramid = Lateral Surface Area (LSA) of the pyramid
Solution:
a.) True, the surface area of any pyramid can be calculated by finding the areas of each of its faces and adding them.
b.) False, the total surface area (TSA) of a pyramid = Lateral Surface Area (LSA) of the pyramid + Base area
Example 3: Calculate the total surface area of a square pyramid if the side of the base is given as 14 inches and the height (altitude) of the pyramid is given as 24 inches.
Solution:
Given, the side of the base is, a = 14 inches, and the height of the pyramid is, h = 24 inches.
Let its slant height be ‘l’.
By Pythagoras theorem, l2 = (a/2)2 + h2
l2 = (14/2)2 + 242 = 625
Thus, l = 25 inches.
The perimeter of the base is, P = 4a = 4(14) = 56 inches.
The base area is, B = 142 = 196 square inches.
The total surface area of the square pyramid is,
TSA = (1/2) Pl + B in which B = 196, P = 56, l = 25
TSA = [(1/2) × 56 × 25] + 196 = 896 square inches
Therefore, the TSA of the given pyramid = 896 square inches.
Frequently Asked Questions on Surface Area of a Pyramid Formula
1: What is the Definition of Surface Area of Pyramid?
Answer: The surface area of a pyramid is defined as the sum of the areas of all its faces. There are two types of surface areas – the Total Surface Area (TSA), which is the sum of the areas of all the faces, and the other is the Lateral Surface Area (LSA), which is the sum of the areas of the side faces.
2: What is the Total Surface Area of Pyramid?
Answer: The total surface area of a pyramid is obtained by adding the area of all its faces (both the base and the side faces). The total surface area of a pyramid whose base perimeter is ‘P’, the base area is ‘B’, and slant height is ‘l’ is calculated using the formula
TSA = (1/2) Pl + B.
3: What is the Lateral Surface Area of Pyramid?
Answer: The lateral surface area of a pyramid is the sum of the areas of all its side faces (which are triangles). The lateral surface area of a pyramid is calculated using the formula LSA = (1/2) Pl, where ‘P’ is the perimeter of the base and ‘l’ is the slant height.
4: What is the Formula for Surface Area of Pyramid?
Answer: There are two types of surface areas of a pyramid, one is the total surface area and the other is the lateral surface area. The formula that is used to find these two areas is given below.
Total surface area = (1/2) Pl + B
Lateral surface area = (1/2) Pl
where ‘B’ is the base area, ‘l’ is the slant height, and ‘P’ is the base perimeter.
5: How to Find Surface Area of Pyramid with Slant Height?
Answer: The formula which is used to find the surface area of a pyramid can be calculated using the slant height. Its total surface area can be calculated using the formula,
(1/2) Pl + B.
Consider a pyramid whose slant height is ‘l’, the base perimeter is ‘P’, and the base area is ‘B’. The base area can be found by applying the formulas of the area of a polygon.
6: How to Find the Surface Area of Pyramid with Height (or Altitude)?
Answer: The surface area of a pyramid can be calculated if the altitude is given. Let us consider a pyramid whose base is a regular polygon of side length ‘a’, the slant height of the pyramid is ‘l’ and its altitude is ‘h’. Now, if only ‘a’ and ‘h’ are given and we need to find the surface area, then we need to find the slant height first. Let us understand this using the following steps.
- Step 1: Find ‘l’ using the Pythagoras theorem, l2 = (a/2)2 + h2
- Step 2: Find the base perimeter ‘P’.
- Step 3: Find the base area ‘B’.
- Step 4: Find the total surface area of the pyramid using the formula (1/2) Pl + B.
7: What is the base area of a square pyramid?
Answer: The base of a square pyramid is a square. Hence, the base area of the square pyramid of base edge a is a2.
8: How do you calculate the volume of a pyramid?
Answer: The volume of a pyramid is measured in cubic units and the formula used to calculate the volume of a pyramid is: Volume of a Pyramid = 1/3 × Base Area × Height









