CBSE Sample Papers for Class 10 SA2 Maths Solved 2016 Set 12
Section A
![]()
2.Find the length of the shadow of a tree 18 m long when the Sun’s angle of elevation is 45°.
3.If two tangents inclined at an angle of 120° are drawn to a circle of radius 5 cm, then find the length of each tangent.
4.A pair of die is thrown. Find the probability of getting sum of numbers which is perfect square and divisible by 5.
Section B
5.Solve for x: a/x-a+b/x-b——— -2; x not equal to a.b
6.Which term of the AP: 125, 121, 117, … is 1st negative term?
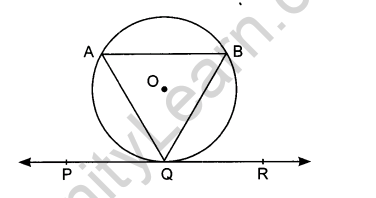
7.In the given figure, PQR is the tangent to a circle at Q whose centre is O. AB is a chord parallel to PR and BOR = 60°. Find triangle AQB.
8.In the given figure, AC is diameter of the circle with centre O. PAQ is the tangent to the circle at A. If AB || CD and ZBAQ = 65°, find triangle DCA.

9.Find a relation between x and y if area of the triangle formed by the points (x, y), (1, 2) and (7,0) is 5 sq units.
10.If the point P(2, 1) lies on the line segment joining the points A(4,2) and B(8,4) then find AP/AB
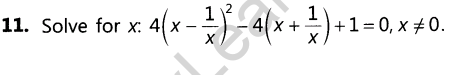
Section C
12.The sum of fourth and the ninth terms of an AP is 46 and their product is 465. Find the sum of first 10 terms of this AP.
13.The angle of elevation of the top of a building from the foot of the tower is 30° and the angle of elevation of the top of the tower from the foot of the building is 60°. If the tower is 50 m high, find the height of the building.
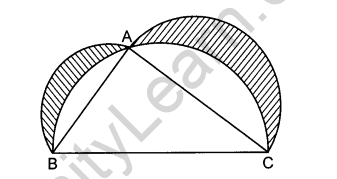
14.In figure ABC is a right angled triangle right angled at A. Semicircles are drawn on AB, AC and BC as diameters. If AB = 6 cm, AC = 8 cm, find the area of the shaded region.
15.A wooden toy rocket is in the shape of a cone mounted on a cylinder, as shown in figure. The height of the entire rocket is 52 cm while the height of the conical part is 12 cm. The base of the conical portion has a diameter of 10 cm, while the base diameter of the cylindrical portion is 6 cm. If the conical portion is to be painted, red and the cylindrical portion green, find the area of the rocket painted with each of these colours. (Take, pi = 3.14)

16.A building is in the form of a cylinder surmounted by a hemispherical dome. The base diameter of the dome is equal to 2/3 of the total height of the building. Find the surface area of the building, if it contains 2816/21 of air.
17.Solid sphere of diameter 12 cm are dropped into a cylindrical vessel containing some water and are fully submerged. If the diameter of the vessel is 36 cm and the water rises by 80 cm, find the number of solid spheres dropped in the water.
18.The height of a cone is 60 cm. A small cone is cut off at the top by a plane parallel to the base. If its volume is 1/27 of the volume of the given cone, at what height above the base is the section made?
19.ABCD is a rectangle formed by the points A(-l, -1), B(-l, 4), C(5, 4) and D(5, -1). P, Q, R and S are the mid-points of AB, BC, CD and AD respectively. Is the quadrilateral PQRS is a square or rhombus? Justify your answer.
20.What is the probability that an year will have 53 Tuesdays?
Section D
21.A swimming pool is filled with three pipes with uniform flow of water. The first two pipes operating simultaneously fill the pool in the same time during which the pool is filled by the third pipe alone. The second pipe fills the pool 5 hrs faster than the third pipe and 4 hrs slower than the third pipe. Find the time required by each pipe to fill the pool separately.
22.If the roots of the quadratic equation: p(q – r)x2 + q(r – p)x + r(p – q) = 0 are equal, show that:1/p+ 1/r = 2/q
23.A contractor employed 150 workers to finish a piece of work in a certain fixed number of days. On the first day, all 150 workers worked. He dropped four workers on the second day, four more workers were dropped on the third day and so on. In this way work got finished in 8 more days. Find the number of days in which work was to be completed originally.

25.The tangent at any point of a circle is perpendicular to the radius through point of contact. Prove it.
26. A contractor was awarded to construct a vertical pillar at a horizontal distance of 200 m from a fixed point. It was decided that angle of elevation of the top of the complete pillar from that point to be 60°. Contractor
finished the job by making a pillar such that the angle of elevation of its top was 45°.
(i) Find the height of the pillar to be increased as per the terms of contract.
(ii) Contractor demands full payment for this work
(a) Is he justified?
(b) Which ‘value’ is he lacking?
27.Draw a right triangle ABC in which AB = 5 cm, BC = 7 cm, B = 90°. Through B, draw BD perpendicular AC. Then draw a circle passing through B, C and D. Construct a pair of tangents to this circle from A.
28.Water is pumped from a sump (an underground tank) to an overhead tank in the shape of cylinder. Sump is in the shape of a cuboid of dimensions 4mx3mx2m. The overhead tank has radius 60 cm and height 80 cm. Find the height of water left in the sump after the overhead tank has been completely filled with water from the sump (assume sump was completely filled before water was pumped to overhead tank). Also compare the capacity of tank with that of sump. (Take pi = 3.14)
29.The area of an equilateral AABC is 17320.5 cm2. With each vertex of the triangle as centre, a circle is drawn with radius equal to half the length of side of the triangle. Find the area of the triangle which is uncommon with circles. (Take pi = 3.14, root 3= 1.73205)
30.Find the coordinates of the point equidistant from three given points A(5, 1), B(-3, -7) and (7, -1).
31.At a fete, cards bearing numbers 1 to 1000, one number on one card are put in a box. Each player select one card at random and that card is not replaced. If the selected card has a perfect square greater than 500, the player wins a prize. What is the probability that: (0 the first player wins a prize? (//) second player wins a prize, if the first has won?





