Surface Areas and Volumes Class 10 Notes Maths Chapter 13

 

CBSE Class 10 Maths Notes Chapter 13 Surface Areas and Volumes

SURFACE AREA AND VOLUME OF COMBINATIONS

Cone on a Cylinder.

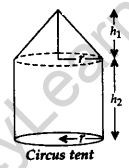
r = radius of cone & cylinder;
h₁ = height of cone
h₂ = height of cylinder
Total Surface area = Curved surface area of cone + Curved surface area of cylinder + area of circular base
= πrl + 2πrh₂ +πr²;
Slant height, l = \(\sqrt { { r }^{ 2 }+{ { { h }_{ 1 }^{ 2 } } } }\)
Total Volume = Volume of cone + Volume of cylinder
= \(\frac { 1 }{ 3 } { \pi r }^{ 2 }{ h }_{ 1 }+{ \pi r }^{ 2 }{ h }_{ 2 }\)

Cone on a Hemisphere:

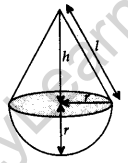
h = height of cone;
l = slant height of cone = \(\sqrt { { r }^{ 2 }+{ h }^{ 2 } }\)
r = radius of cone and hemisphere
Total Surface area = Curved surface area of cone + Curved surface area of hemisphere = πrl + 2πr²
Volume = Volume of cone + Volume of hemisphere = \(\frac { 1 }{ 3 } { \pi r }^{ 2 }h+\frac { 2 }{ 3 } { \pi r }^{ 3 }\)

Conical Cavity in a Cylinder

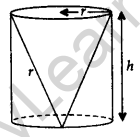
r = radius of cone and cylinder;
h = height of cylinder and conical cavity;
l = Slant height
Total Surface area = Curved surface area of cylinder + Area of bottom face of cylinder + Curved surface area of cone = 2πrh + πr² + πrl
Volume = Volume of cylinder – Volume of cone = \({ \pi r }^{ 2 }h-\frac { 1 }{ 3 } { \pi r }^{ 2 }h=\frac { 2 }{ 3 } { \pi r }^{ 2 }h\)

Cones on Either Side of Cylinder.

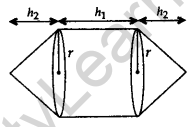
r = radius of cylinder and cone;
h₁ = height of cylinder
h₂ = height of cones
Slant height of cone, l = \(\sqrt { { h }_{ 2 }^{ 2 }+{ r }^{ 2 } }\)
Surface area = Curved surface area of 2 cones + Curved surface area of cylinder = 2πrl + 2πrh₁
Volume = 2(Volume of cone) + Volume of cylinder = \(\frac { 2 }{ 3 } { \pi r }^{ 2 }{ h }_{ 2 }+{ \pi r }^{ 2 }{ h }_{ 1 }\)

Cylinder with Hemispherical Ends.

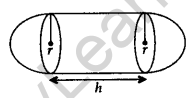
r = radius of cylinder and hemispherical ends;
h = height of cylinder
Total surface area= Curved surface area of cylinder + Curved surface area of 2 hemispheres = 2πrh + 4πr²
Volume = Volume of cylinder + Volume of 2 hemispheres = \({ \pi r }^{ 2 }h+\frac { 4 }{ 3 } { \pi r }^{ 3 }\)

Hemisphere on Cube or Hemispherical Cavity on Cube

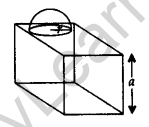
a = side of cube;
r = radius of hemisphere.
Surface area = Surface area of cube – Area of hemisphere face + Curved surface area of hemisphere
= 6a² – πr² + 2πr² = 6a² + πr²
Volume = Volume of cube + Volume of hemisphere = \({ a }^{ 3 }+\frac { 4 }{ 3 } { \pi r }^{ 3 }\)

Hemispherical Cavity in a Cylinder

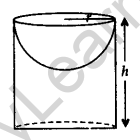
r = radius of hemisphere;
h = height of cylinder
Total surface area = Curved surface area of cylinder + Surface area of base + Curved surface area of hemisphere
= 2πrh + πr² + 2πr² = 2πrh + 3πr²
Volume = Volume of cylinder – Volume of hemisphere = \({ \pi r }^{ 2 }h-\frac { 2 }{ 3 } { \pi r }^{ 3 }\)

We hope the given CBSE Class 10 Maths Notes Chapter 13 Surface Areas and Volumes Pdf free download will help you. If you have any query regarding NCERT Class 10 Maths Notes Chapter 13 Surface Areas and Volumes, drop a comment below and we will get back to you at the earliest.