Introduction to Euclid’s Geometry Class 9 Notes Maths Chapter 3

CBSE Class 9 Maths Notes Chapter 3 Introduction to Euclid’s Geometry

1. Axioms or Postulates: Axioms or postulates are the assumptions which are obvious universal truths. They are not proved.
Mainly postulates are used for especially geometry and axioms are used for especially algebra.

2. Theorems: Theorems are statements which are proved using ‘ definitions, axioms, previously proved statements and deductive reasoning.

3. Euclid’s Definitions, Axioms or Postulates

• A point is that which has no part.
• A line is a breadthless length.
• The ends of a line are points.
• A straight line is a line which lies evenly with the points on itself.
• A surface is that which has length and breadth only.
• The edges of a surface are lines.
• A plane surface is a surface which lies evenly with the straight lines on itself.

4. Undefined Terms: There are some terms like point etc which have no definitions, these terms are called undefined terms.
In geometry, a point, a line and a plane (in Euclid’s words, a plane surface) are undefined terms.

5. Euclid’s Axioms

• Things which are equal to the same thing are equal to one another.
• If equals are added to equals, the wholes are equal.
• If equals are subtracted from equals, the remainders are equal.
• Things which coincide with one another are equal to one another.
• The whole is greater than the part.
• Things which are double of the same things are equal to one another.
• Things which are halves of the same things are equal to one another.

6. Euclid’s Five Postulates: Euclid’s five postulates are given below
Postulate 1: A straight line may be drawn from any one point to any other point.

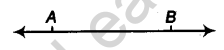
Axiom Given two distinct points, there is a unique line that passes through them.

Postulate 2: A terminated line can be produced indefinitely. Euclid’s terminated line is called a line segment. So, according to the present day terms, ‘A line segment can be extended on either side to form a line’.

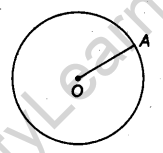
Postulate 3: A circle can be drawn with any centre and any radius.

Postulate 4: All right angles are equal to one another.

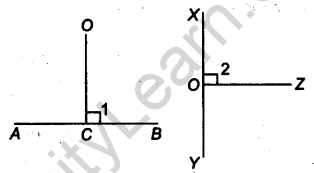
If ∠1 = 90°
and ∠2 = 90°
∠1 = ∠2

Postulate 5: If a straight line falling on two straight lines makes the interior angles on the same side of it taken together less than two right angles, then the two straight lines, if produced indefinitely, meet on that side on which the sum of angles is less than two right angles.

e.g., The line PQ falls on lines AB and CD such that the sum of the interior angles 1 and 2 is less than 180° on the left side of PQ. Therefore, the lines AB and CD will eventually intersect on the left side of PQ.

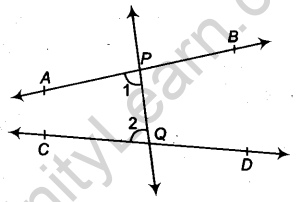
Note: Two distinct lines cannot have more than one point in common.

7. Equivalent Versions of Euclid’s Fifth Postulate: ‘For every line l and for every point P not lying on l, there exists a unique line m passing through P and parallel to ‘l’.
This result can also be stated in the following form.
Two distinct intersecting lines cannot be parallel to the same line.

We hope the given CBSE Class 9 Maths Notes Chapter 3 Introduction to Euclid’s Geometry Pdf free download will help you. If you have any query regarding NCERT Class 9 Maths Notes Chapter 3 Introduction to Euclid’s Geometry, drop a comment below and we will get back to you at the earliest.