Table of Contents
NCERT Solutions for Class 8 Maths Chapter 2 Linear Equations in One Variable Exercise 2.3
NCERT Solutions for Class 8 Maths Chapter 2 solutions are important for CBSE Exam preparation as well as preparation for competitive exams like JEE
Solve the following equations and check your results.
Ex 2.3 Class 8 Maths Question 1.
3x = 2x + 18
Solution:
We have 3x = 2x + 18
⇒ 3x – 2x = 18 (Transposing 2x to LHS)
⇒ x = 18
Hence, x = 18 is the required solution.
Check: 3x = 2x + 18
Putting x = 18, we have
LHS = 3 × 18 = 54
RHS = 2 × 18 + 18 = 36 + 18 = 54
LHS = RHS
Hence verified.
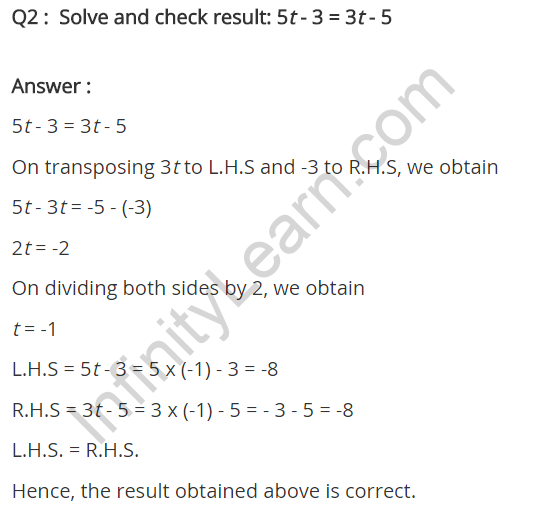
Ex 2.3 Class 8 Maths Question 2.
5t – 3 = 3t – 5
Solution:
We have 5t – 3 = 3t – 5
⇒ 5t – 3t – 3 = -5 (Transposing 3t to LHS)
⇒ 2t = -5 + 3 (Transposing -3 to RHS)
⇒ 2t = -2
⇒ t = -2 ÷ 2
⇒ t = -1
Hence t = -1 is the required solution.
Check: 5t – 3 = 3t – 5
Putting t = -1, we have
LHS = 5t – 3 = 5 × (-1)-3 = -5 – 3 = -8
RHS = 3t – 5 = 3 × (-1) – 5 = -3 – 5 = -8
LHS = RHS
Hence verified.
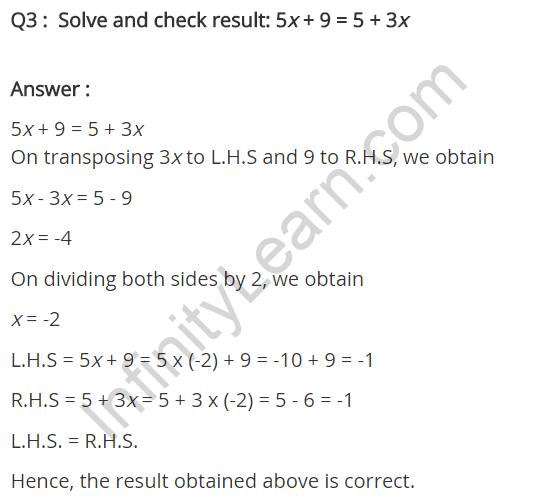
Ex 2.3 Class 8 Maths Question 3.
5x + 9 = 5 + 3x
Solution:
We have 5x + 9 = 5 + 3x
⇒ 5x – 3x + 9 = 5 (Transposing 3x to LHS) => 2x + 9 = 5
⇒ 2x = 5 – 9 (Transposing 9 to RHS)
⇒ 2x = -4
⇒ x = -4 ÷ 2 = -2
Hence x = -2 is the required solution.
Check: 5x + 9 = 5 + 3x
Putting x = -2, we have
LHS = 5 × (-2) + 9 = -10 + 9 = -1
RHS = 5 + 3 × (-2) = 5 – 6 = -1
LHS = RHS
Hence verified.
Ex 2.3 Class 8 Maths Question 4.
4z + 3 = 6 + 2z
Solution:
We have 4z + 3 = 6 + 2z
⇒ 4z – 2z + 3 = 6 (Transposing 2z to LHS)
⇒ 2z + 3 = 6
⇒ 2z = 6 – 3 (Transposing 3 to RHS)
⇒ 2z = 3
⇒ z = \(\frac { 3 }{ 2 }\)
Hence z = \(\frac { 3 }{ 2 }\) is the required solution.
Check: 4z + 3 = 6 + 2z
Putting z = \(\frac { 3 }{ 2 }\), we have
LHS = 4z + 3 = 4 × \(\frac { 3 }{ 2 }\) + 3 = 6 + 3 = 9
RHS = 6 + 2z = 6 + 2 × \(\frac { 3 }{ 2 }\) = 6 + 3 = 9
LHS = RHS
Hence verified.
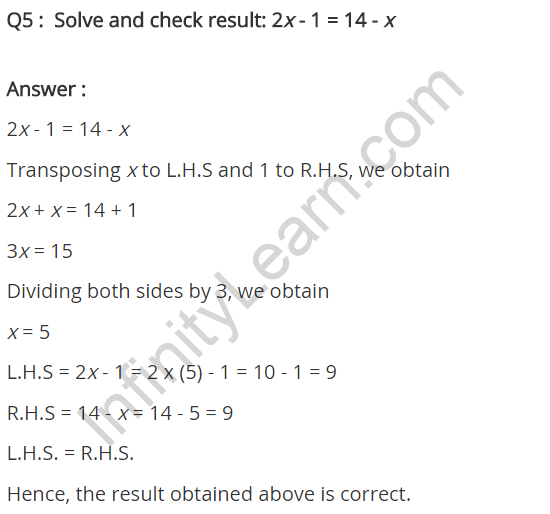
Ex 2.3 Class 8 Maths Question 5.
2x – 1 = 14 – x
Solution:
We have 2x – 1 = 14 – x
⇒ 2x + x = 14 + 1 (Transposing x to LHS and 1 to RHS)
⇒ 3x = 15
⇒ x = 15 ÷ 3 = 5
Hence x = 5 is the required solution.
Check: 2x – 1 = 14 – x
Putting x = 5
LHS we have 2x – 1 = 2 × 5 – 1 = 10 – 1 = 9
RHS = 14 – x = 14 – 5 = 9
LHS = RHS
Hence verified.
Ex 2.3 Class 8 Maths Question 6.
8x + 4 = 3(x – 1) + 7
Solution:
We have 8x + 4 = 3(x – 1) + 7
⇒ 8x + 4 = 3x – 3 + 7 (Solving the bracket)
⇒ 8x + 4 = 3x + 4
⇒ 8x – 3x = 4 – 4 [Transposing 3x to LHS and 4 to RHS]
⇒ 5x = 0
⇒ x = 0 ÷ 5 [Transposing 5 to RHS]
or x = 0
Thus x = 0 is the required solution.
Check: 8x + 4 = 3(x – 1) + 7
Putting x = 0, we have
8 × 0 + 4 = 3(0 – 1) + 7
⇒ 0 + 4 = -3 + 7
⇒ 4 = 4
LHS = RHS
Hence verified.
Ex 2.3 Class 8 Maths Question 7.
x = \(\frac { 4 }{ 5 }\) (x + 10)
Solution:
We have x = \(\frac { 4 }{ 5 }\) (x + 10)
⇒ 5 × x = 4(x + 10) (Transposing 5 to LHS)
⇒ 5x = 4x + 40 (Solving the bracket)
⇒ 5x – 4x = 40 (Transposing 4x to LHS)
⇒ x = 40
Thus x = 40 is the required solution.
Check: x = \(\frac { 4 }{ 5 }\) (x + 10)
Putting x = 40, we have
40 = \(\frac { 4 }{ 5 }\) (40 + 10)
⇒ 40 = \(\frac { 4 }{ 5 }\) × 50
⇒ 40 = 4 × 10
⇒ 40 = 40
LHS = RHS
Hence verified.
Ex 2.3 Class 8 Maths Question 8.
\(\frac { 2x }{ 3 }\) + 1 = \(\frac { 7x }{ 15 }\) + 3
Solution:
We have \(\frac { 2x }{ 3 }\) + 1 = \(\frac { 7x }{ 15 }\) + 3
15(\(\frac { 2x }{ 3 }\) + 1) = 15(\(\frac { 7x }{ 15 }\) + 3)
LCM of 3 and 15 is 15
\(\frac { 2x }{ 3 }\) × 15 + 1 × 15 = \(\frac { 7x }{ 15 }\) × 15 + 3 × 15 [Multiplying both sides by 15]
⇒ 2x × 5 + 15 = 7x + 45
⇒ 10x + 15 = 7x + 45
⇒ 10x – 7x = 45 – 15 (Transposing 7x to LHS and 15 to RHS)
⇒ 3x = 30
⇒ x = 30 ÷ 3 = 10 (Transposing 3 to RHS)
Thus the required solution is x = 10

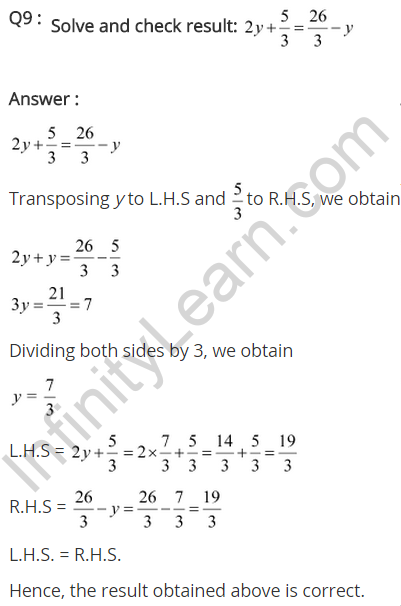
Ex 2.3 Class 8 Maths Question 9.
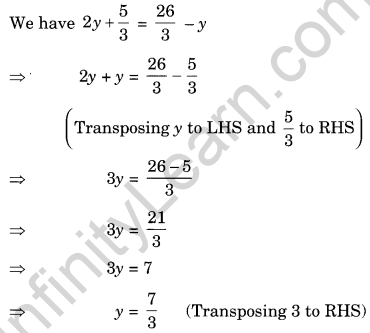
2y + \(\frac { 5 }{ 3 }\) = \(\frac { 26 }{ 3 }\) – y
Solution:

Ex 2.3 Class 8 Maths Question 10.
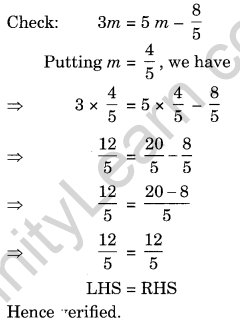
3m = 5m – \(\frac { 8 }{ 5 }\)
Solution:
We have







Infinity Learn App
Now you can find answers to all your subject queries & prepare for your Exams on our Learning App – Infinity Learn.





