Sequence
A succession of numbers arranged in a definite order according to a given certain rule is called sequence. A sequence is either finite or infinite depending upon the number of terms in a sequence.
Series
If a1, a2, a3,…… an is a sequence, then the expression a1 + a2 + a3 + a4 + … + an is called series.
Progression
A sequence whose terms follow certain patterns are more often called progression.
Arithmetic Progression (AP)
A sequence in which the difference of two consecutive terms is constant, is called Arithmetic progression (AP).
Properties of Arithmetic Progression (AP)
If a sequence is an A.P. then its nth term is a linear expression in n i.e. its nth term is given by An + B, where A and S are constant and A is common difference.
nth term of an AP : If a is the first term, d is common difference and l is the last term of an AP then
- nth term is given by an = a + (n – 1)d.
- nth term of an AP from the last term is a’n =an – (n – 1)d.
- an + a’n = constant
- Common difference of an AP i.e. d = an – an-1,∀ n > 1.
If a constant is added or subtracted from each term of an AR then the resulting sequence is an AP with same common difference.
If each term of an AP is multiplied or divided by a non-zero constant, then the resulting sequence is also an AP.
If a, b and c are three consecutive terms of an A.P then 2b = a + c.
Any three terms of an AP can be taken as (a – d), a, (a + d) and any four terms of an AP can be taken as (a – 3d), (a – d), (a + d), (a + 3d)
Sum of n Terms of an AP
Sum of n terms of an AP is given by
Sn = \(\frac { n }{ 2 }\) [2a + (n – 1)d] = \(\frac { n }{ 2 }\) (a1+ an)
A sequence is an AP If the sum of n terms is of the form An2 + Bn, where A and B are constant and A = half of common difference i.e. 2A = d.
an =Sn – Sn-1
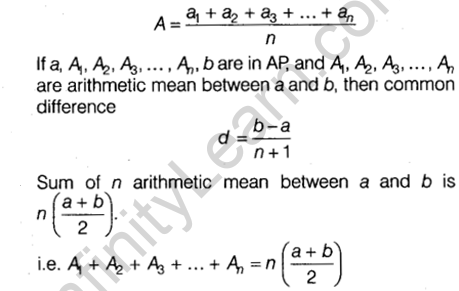
Arithmetic Mean
If a, A and b are in A.P then A = \(\frac { a+b }{ 2 }\) is called the arithmetic mean of a and b.
If a1, a2, a3,……an are n numbers, then their arithmetic mean is given by
Geometric Progression (GP)
A sequence in which the ratio of two consecutive terms is constant is called geometric progression. The constant ratio is called common ratio(r).
i.e. r = \(\frac { { a }_{ n }+1 }{ { a }_{ n } }\), ∀ n > 1
Properties of Geometric Progression
If a is the first term and r is the common ratio, then the general term or nth term of GP is an =arn-1
nth term of a GP from the end is a’n = \(\frac { 1 }{ { r }^{ n-1 } }\), l = last term
If all the terms of GP be multiplied or divided by same non-zero constant, then the resulting sequence is a GP with the same common ratio.
The reciprocal terms of a given GP form a GP.
If each term of a GP be raised to some power, the resulting sequence also forms a GP
If a, b and c are three consecutive terms of a GP then b2 = ac.
Any three terms can be taken in GP as \(\frac { a }{ r }\), a and ar and any four terms can be taken in GP as \(\frac { a }{ { r }^{ 3 } }\), \(\frac { a }{ r }\), ar and ar3.
Sum of n Terms of a G.P

Geometric Mean (GM)
If a, G and b are in GR then G is called the geometric mean of a and b and is given by G = √(ab).
If a,G1, G2, G3,….. Gn, b are in GP then G1, G2, G3,……Gn are in GM’s between a and b, then
common ratio r = \(\left( \frac { b }{ a } \right) ^{ \frac { 1 }{ n+1 } }\)
If a1, a2, a3,…, an are n numbers are non-zero and non-negative, then their GM is given by
GM = (a1 . a2 . a3 …an)1/n
Product of n GM is G1 × G2 × G3 ×… × Gn =Gn = \(\left( ab \right) ^{ \frac { n }{ 2 } }\)
Important Results on the Sum of Special Sequences
Sum of first n natural numbers is
Σn = 1 + 2 + 3 +… + n = \(\frac { n(n+1) }{ 2 }\)
Sum of squares of first n natural numbers is
Σn2 = 12 + 22 + 32 + … + n2 = \(\frac { n(n+1)(2n+1) }{ 6 }\)
Sum of cubes of first n natural numbers is
Σn3 = 13 + 23 + 33 + .. + n3 = \(\left( \frac { n\left( n+1 \right) \left( 2n+1 \right) }{ 6 } \right) ^{ 2 }\)



