Table of Contents
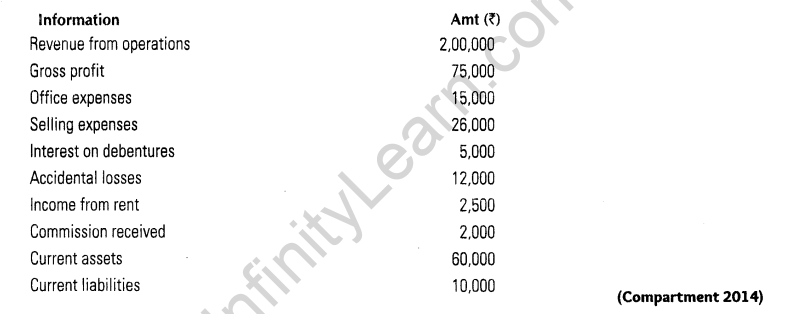
Total Surface Area of a Cylinder: Let us carry out a small activity. Take a ring. We know that ring is circular in shape. Now place another ring on the first ring and so on. You will see that when you place such rings, the structure that you get is a cylinder, which is three dimensional. Let us learn about the volume of a Cylinder and Total Surface area of a Cylinder in this chapter.
Cylinder
Any solid which has two circular ends along with a curved surface area is said to be a cylinder. It has a curved surface in the middle. The base and the top surface are identical. That means the bases are always parallel and congruent to each other. Cylinder has no vertices.
A cylinder is a three-dimensional figure with two circular bases that are congruent and parallel. The height of a cylinder is the distance between the two bases. The total surface area of a cylinder is the sum of the areas of the two circular bases and the area of the cylindrical surface.
To calculate the total surface area of a cylinder, you need to know the radius of the circular bases and the height of the cylinder. The radius is half the diameter of the circular base. The diameter is the distance across the circular base. The height is the distance between the two bases.
The following formula can be used to calculate the total surface area of a cylinder
A = 2πr² + πrh
Total Surface Area of Cylinder
The total surface area of cylinder is the area of the circles plus the area of the round section in the midle. The Surface Area (SA) is found with the formula: SA = 2πr2 + 2πrh
Find the Total Surface Area of each figure. Remember, d = 2r. Type your answers in the comment box
(i)
(ii)
(iii)
(iv)
(v)
(vi)
(vii)
(viii)
(ix)
Calculate the total surface area of a cylinder for the given problems and type your answers in the comment box.
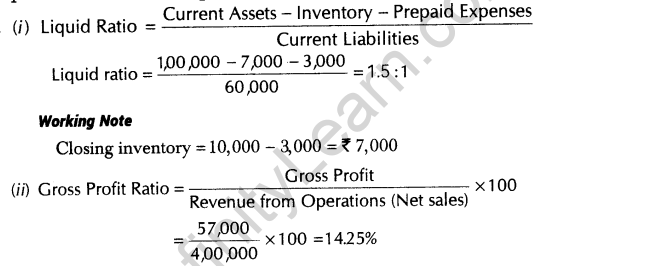
Volume of Cylinder
The Volume of Cylinder is the product of the area of the base (B) times the height. The formula for the Volume of the Cylinder is V = B x H. Volume is expressed in cubic units , or units3
Find the Volume of each figure. Remember, d = 2r. Type your answers in the comment box
(i)
(ii)
(iii)
(iv)
(v)
(vi)
(vii)
(viii)
(ix)
Calculate the volume of cylinder for the given problems and type your answers in the comment box.
Volume of a Cylinder Formulas
A cylinder is a three-dimensional figure with two circular bases. The volume of a cylinder is found by multiplying the area of the base by the height of the cylinder. The formulas for the volume of a cylinder are:
Volume = πr2h
Volume = Bh
Where r is the radius of the base and h is the height of the cylinder.
The following examples will help to illustrate how to use these formulas.
Example 1
Find the volume of the cylinder with a radius of 5 cm and a height of 10 cm.
Volume = πr2h
Volume = π(5 cm)2(10 cm)
Volume = π(25 cm2)
Volume = 250 cm3
Example 2
Find the volume of the cylinder with a radius of 10 cm and a height of 8 cm.
Volume = πr2h
Volume = π(10 cm)2(8 cm)
Volume = π(100 cm2)
Volume = 1000 cm3
Related Information:
| Surface Area Formulas | Equilateral Triangle Formula |
| Perimeter of a Parallelogram Formula | Radius Formula |
| Trapezoid formula | Diagonal of a Cube Formula |
| Parallelogram Formula | Percentage Increase Formula |
