Table of Contents
Understanding triangles is crucial for scoring well in Class 10 Maths. Below, we provide a comprehensive list of important questions for Chapter 6: Triangles. Each question is designed to help students strengthen their understanding of key triangle concepts and formulas.
Important Questions for Class 10 Maths Chapter 6 Key Concepts Covered
- Congruence of Triangles: Understanding the conditions for triangle congruence (SSS, SAS, ASA, RHS).
- Similarity of Triangles: Focus on criteria such as AA, SAS, and SSS for triangle similarity.
- Pythagoras Theorem: Solving real-life and geometric problems using the Pythagoras theorem.
Important Questions for Practice
- Prove the congruence of two triangles given specific conditions using SSS criterion.
- Find the missing sides of similar triangles using the properties of proportionality.
- Apply Pythagoras Theorem to solve practical problems.
Also Check: CBSE Syllabus for Class 10
Important Questions Class 10 Maths Chapter 6 Triangles
Question 1. If ∆ABC ~ ∆PQR, perimeter of ∆ABC = 32 cm, perimeter of ∆PQR = 48 cm and PR = 6 cm, then find the length of AC. (2012)
Solution: ∆ABC ~ ∆PQR …[Given

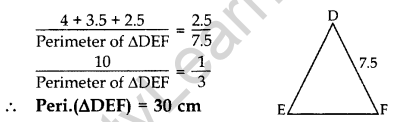
Question 2. ∆ABC ~ ∆DEF. If AB = 4 cm, BC = 3.5 cm, CA = 2.5 cm and DF = 7.5 cm, find the perimeter of ∆DEF. (2012, 2017D)
Solution: ∆ABC – ∆DEF …[Given
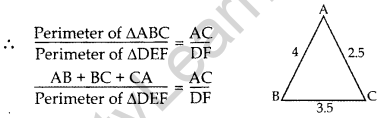
Question 3. If ∆ABC ~ ∆RPQ, AB = 3 cm, BC = 5 cm, AC = 6 cm, RP = 6 cm and PQ = 10, then find QR. (2014)
Solution: ∆ABC ~ ∆RPQ …[Given]
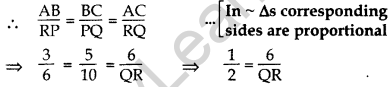
∴ QR = 12 cm
Question 4. In ∆DEW, AB || EW. If AD = 4 cm, DE = 12 cm and DW = 24 cm, then find the value of DB. (2015)
Solution: Let BD = x cm
then BW = (24 – x) cm, AE = 12 – 4 = 8 cm
In ∆DEW, AB || EW

Question 5. In ∆ABC, DE || BC, find the value of x. (2015)

Solution: In ∆ABC, DE || BC …[Given]

x(x + 5) = (x + 3)(x + 1)
x2 + 5x = x2 + 3x + x + 3
x2 + 5x – x2 – 3x – x = 3
∴ x = 3 cm
Question 6. In the given figure, if DE || BC, AE = 8 cm, EC = 2 cm and BC = 6 cm, then find DE. (2014)
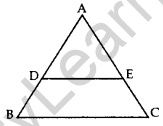
Solution: In ∆ADE and ∆ABC,
∠DAE = ∠BAC …Common
∠ADE – ∠ABC … [Corresponding angles
∆ADE – ∆ΑΒC …[AA corollary

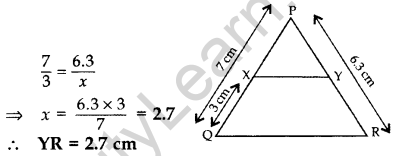
Question 7. In the given figure, XY || QR, \(\frac{P Q}{X Q}=\frac{7}{3}\) and PR = 6.3 cm, find YR. (2017OD)
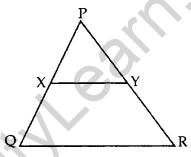
Solution: Let YR = x
\(\frac{\mathrm{PQ}}{\mathrm{XQ}}=\frac{\mathrm{PR}}{\mathrm{YR}}\) … [Thales’ theorem
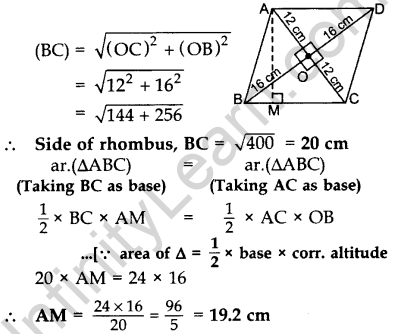
Question 8. The lengths of the diagonals of a rhombus are 24 cm and 32 cm. Calculate the length of the altitude of the rhombus. (2013)
Solution: Diagonals of a rhombus are ⊥ bisectors of each other.
∴ AC ⊥ BD,
OA = OC = \(\frac{A C}{2} \Rightarrow \frac{24}{2}\) = 12 cm
OB = OD = \(\frac{B D}{2} \Rightarrow \frac{32}{2}\) = 16 cm
In rt. ∆BOC,
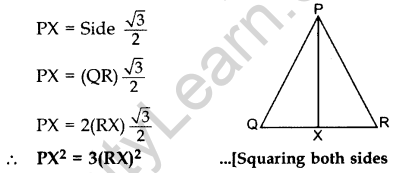
Question 9. If PQR is an equilateral triangle and PX ⊥ QR, find the value of PX2. (2013)
Solution: Altitude of an equilateral ∆,
Important Questions Class 10 Maths Chapter 6 Triangles Short Answer-I (2 Marks)
Question 10. The sides AB and AC and the perimeter P, of ∆ABC are respectively three times the corresponding sides DE and DF and the perimeter P, of ∆DEF. Are the two triangles similar? If yes, find \(\frac { ar\left( \triangle ABC \right) }{ ar\left( \triangle DEF \right) } \) (2012)
Solution:
Given: AB = 3DE and AC = 3DF
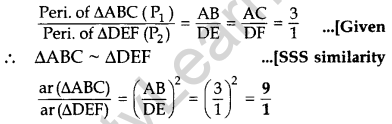
The ratio of the areas of two similar ∆s is equal to the ratio of the squares of their corresponding sides
Question 11. In the figure, EF || AC, BC = 10 cm, AB = 13 cm and EC = 2 cm, find AF. (2014)
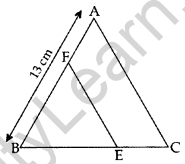
Solution: BE = BC – EC = 10 – 2 = 8 cm
Let AF = x cm, then BF = (13 – x) cm
In ∆ABC, EF || AC … [Given

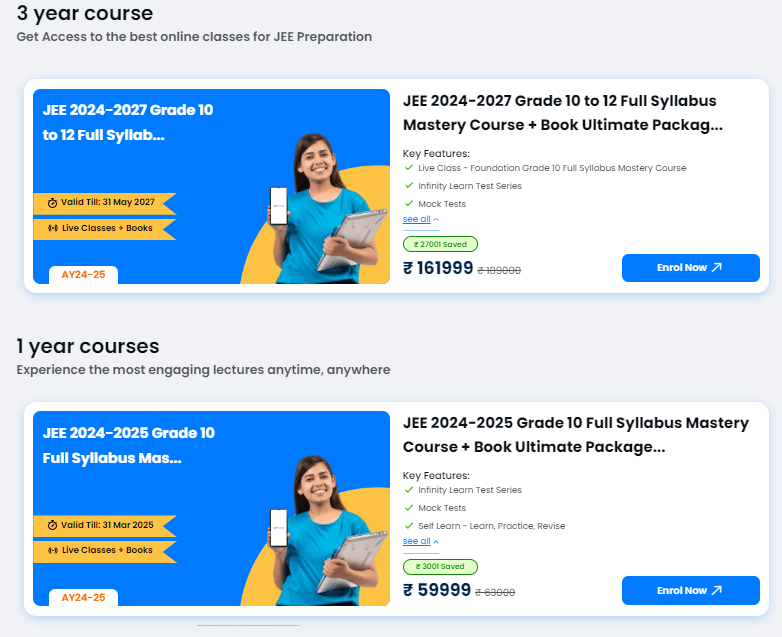
Question 12. X and Y are points on the sides AB and AC respectively of a triangle ABC such that \(\frac{\mathbf{A X}}{\mathbf{A B}}=\frac{1}{4}\), AY = 2 cm and YC = 6 cm. Find whether XY || BC or not. (2015)
Solution: Given: \(\frac{A X}{A B}=\frac{1}{4}\)
AX = 1K, AB = 4K
∴ BX = AB – AX
= 4K – 1K = 3K

∴ XY || BC … [By converse of Thales’ theorem]
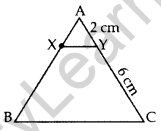
Question 13. In the given figure, ∠A = 90°, AD ⊥ BC. If BD = 2 cm and CD = 8 cm, find AD. (2012; 2017D)

Solution: ∆ADB ~ ∆CDA …[If a perpendicular is drawn from the vertex of the right angle of a rt. ∆ to the hypotenuse then As on both sides of the ⊥ are similar to the whole D and to each other
∴ \(\frac{B D}{A D}=\frac{A D}{C D}\) …[∵ Sides are proportional
AD2 = BD , DC
AD2 = (2) (8) = 16 ⇒ AD = 4 cm
Question 14. In ∆ABC, ∠BAC = 90° and AD ⊥ BC. Prove that AD\frac{B D}{A D}=\frac{A D}{C D} = BD × DC. (2013)
Solution: In 1t. ∆BDA, ∠1 + ∠5 = 90°
n rt. ∆BAC, ∠1 + ∠4 = 90° …(ii)
∠1 + ∠5 = ∠1 + ∠4 …[From (i) & (ii)
.. ∠5 = ∠4 …(iii)
In ∆BDA and ∆ADC,
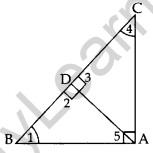
∠5 = 24 … [From (iii)
∠2 = ∠3 …[Each 90°
∴ ∆BDA ~ ∆ADC…[AA similarity
\(\frac{B D}{A D}=\frac{A D}{C D}\)
… [In ~ As corresponding BA sides are proportional
∴ AD2 = BD × DC
Question 15. A 6.5 m long ladder is placed against a wall such that its foot is at a distance of 2.5 m from the wall. Find the height of the wall where the top of the ladder touches it. (2015)
Solution: Let AC be the ladder and AB be the wall.

∴Required height, AB = 6 m
Question 16.If in the figure ABC and DBC are two right triangles. Prove that AP × PC = BP × PD. (2013)
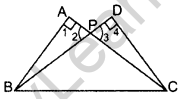
Solution:
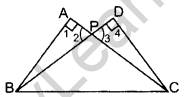
In ∆APB and ∆DPC,
∠1 = ∠4 … [Each = 90°
∠2 = ∠3 …[Vertically opp. ∠s
∴ ∆APB ~ ∆DPC …[AA corollary
⇒ \(\frac{\mathrm{BP}}{\mathrm{PC}}=\frac{\mathrm{AP}}{\mathrm{PD}}\) … [Sides are proportional
∴ AP × PC = BP × PD
Question 17. In the given figure, QA ⊥ AB and PB ⊥ AB. If AO = 20 cm, BO = 12 cm, PB = 18 cm, find AQuestion (2017OD)
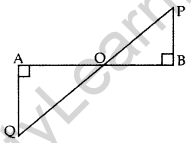
Solution:
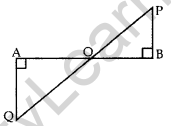
In ∆OAQ and ∆OBP,
∠OAQ = ∠OBP … [Each 90°
∠AOQ = ∠BOP … [vertically opposite angles

Triangles Class 10 Important Questions Short Answer-II (3 Marks)
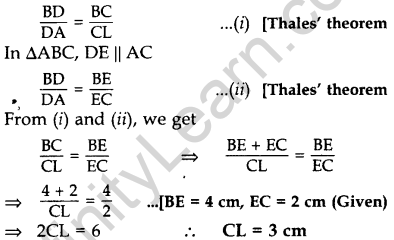
Question 18. In the given figure, CD || LA and DE || AC. Find the length of CL if BE = 4 cm and EC = 2 cm. (2012)
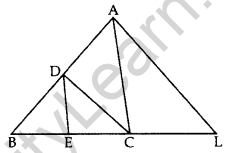
Solution:
In ∆ABL, CD || LA
Question 19. If a line segment intersects sides AB and AC of a ∆ABC at D and E respectively and is parallel to BC, prove that \(\frac{A D}{A B}=\frac{A E}{A C}\). (2013)
Solution: Given. In ∆ABC, DE || BC
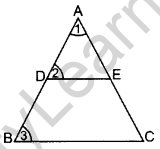
To prove. \(\frac{\mathrm{AD}}{\mathrm{AB}}=\frac{\mathrm{AE}}{\mathrm{AC}}\)
Proof.
In ∆ADE and ∆ABC
∠1 = ∠1 … Common
∠2 = ∠3 … [Corresponding angles
∆ADE ~ ∆ABC …[AA similarity
∴ \(\frac{\mathbf{A D}}{\mathbf{A B}}=\frac{\mathbf{A} \mathbf{E}}{\mathbf{A C}}\)
…[In ~∆s corresponding sides are proportional
Question 20. In a ∆ABC, DE || BC with D on AB and E on AC. If \(\frac{A D}{D B}=\frac{3}{4}\) , find \(\frac{\mathbf{B} C}{\mathbf{D} \mathbf{E}}\). (2013)
Solution:
Given: In a ∆ABC, DE || BC with D on AB and E on AC and \(\frac{A D}{D B}=\frac{3}{4}\)
To find: \(\frac{\mathrm{BC}}{\mathrm{DE}}\)
Proof. Let AD = 3k,

DB = 4k
∴ AB = 3k + 4k = 7k
In ∆ADE and ∆ABC,
∠1 = ∠1 …[Common
∠2 = ∠3 … [Corresponding angles
∴ ∆ADE ~ ∆ABC …[AA similarity

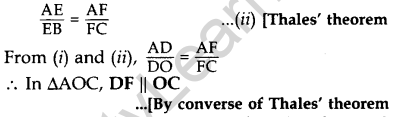
Question 21. In the figure, if DE || OB and EF || BC, then prove that DF || OC. (2014)
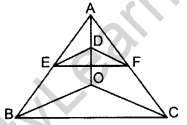
Solution:
Given. In ∆ABC, DE || OB and EF || BC
To prove. DF || OC
Proof. In ∆AOB, DE || OB … [Given
Question 22. If the perimeters of two similar triangles ABC and DEF are 50 cm and 70 cm respectively and one side of ∆ABC = 20 cm, then find the corresponding side of ∆DEF. (2014)
Solution:
Given. ∆ABC ~ ∆DEF,
Perimeter(∆ABC) = 50 cm
Perimeter(∆DEF) = 70 cm
One side of ∆ABC = 20 cm
To Find. Corresponding side of ∆DEF (i.e.,) DE. ∆ABC ~ ∆DEF …[Given

∴ The corresponding side of ADEF = 28 cm
Question 23. A vertical pole of length 8 m casts a shadow 6 cm long on the ground and at the same time a tower casts a shadow 30 m long. Find the height of tower. (2014)
Solution:
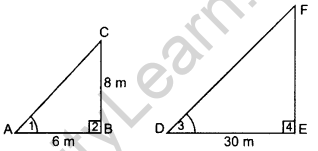
Let BC be the pole and EF be the tower Shadow AB = 6 m and DE = 30 m.
In ∆ABC and ∆DEF,
∠2 = ∠4 … [Each 90°
∠1 = ∠3 … [Sun’s angle of elevation at the same time
∆ABC ~ ∆DEF …[AA similarity
\(\frac{A B}{D E}=\frac{B C}{E F}\) … [In -As corresponding sides are proportional
⇒ \(\frac{6}{30}=\frac{8}{\mathrm{EF}}\) ∴ EF = 40 m
Question 24. In given figure, EB ⊥ AC, BG ⊥ AE and CF ⊥ AE (2015)
Prove that:
(a) ∆ABG ~ ∆DCB
(b) \(\frac{\mathbf{B C}}{\mathbf{B D}}=\frac{\mathbf{B E}}{\mathbf{B A}}\)
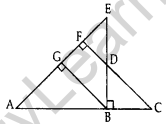
Solution:
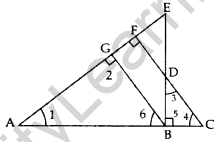
Given: EB ⊥ AC, BG ⊥ AE and CF ⊥ AE.
To prove: (a) ∆ABG – ∆DCB,
(b) \(\frac{B C}{B D}=\frac{B E}{B A}\)
Proof: (a) In ∆ABG and ∆DCB,
∠2 = ∠5 … [each 90°
∠6 = ∠4 … [corresponding angles
∴ ∆ABG ~ ∆DCB … [By AA similarity
(Hence Proved)
∴ ∠1 = ∠3 …(CPCT … [In ~∆s, corresponding angles are equal
(b) In ∆ABE and ∆DBC,
∠1 = ∠3 …(proved above
∠ABE = ∠5 … [each is 90°, EB ⊥ AC (Given)
∆ABE ~ ∆DBC … [By AA similarity
\(\frac{B C}{B D}=\frac{B E}{B A}\)
… [In ~∆s, corresponding sides are proportional
∴ \(\frac{B C}{B D}=\frac{B E}{B A}\) (Hence Proved)
Question 25. ∆ABC ~ ∆PQR. AD is the median to BC and PM is the median to QR. Prove that \(\frac{\mathbf{A B}}{\mathbf{P Q}}=\frac{\mathbf{A D}}{\mathbf{P M}}\). (2017D)
Solution:
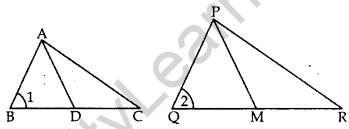
∆ABC ~ ∆PQR … [Given
∠1 = ∠2 … [In ~∆s corresponding angles are equal

Question 26. State whether the given pairs of triangles are similar or not. In case of similarity mention the criterion. (2015)

Solution:
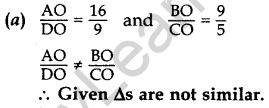
(b) In ∆PQR, ∠P + ∠Q + ∠ZR = 180° …[Angle-Sum Property of a ∆
45° + 78° + ∠R = 180°
∠R = 180° – 45° – 78° = 57°
In ∆LMN, ∠L + ∠M + ∠N = 180° …[Angle-Sum Property of a ∆
57° + 45° + ∠N = 180°
∠N = 180° – 57 – 45° = 78°
∠P = ∠M … (each = 45°
∠Q = ∠N … (each = 78°
∠R = ∠L …(each = 57°
∴ ∆PQR – ∆MNL …[By AAA similarity theorem
Question 27. In the figure of ∆ABC, D divides CA in the ratio 4 : 3. If DE || BC, then find ar (BCDE) : ar (∆ABC). (2015)
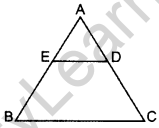
Solution:
Given:
D divides CA in 4 : 3
CD = 4K
DA = 3K
DE || BC …[Given
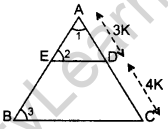
In ∆AED and ∆ABC,
∠1 = ∠1 …[common
∠2 = ∠3 … corresponding angles
∴ ∆AED – ∆ABC …(AA similarity
⇒ \(\frac { ar\left( \triangle AED \right) }{ ar\left( \triangle ABC \right) } =\left( \frac { AD }{ AC } \right) ^{ 2 }\)
… [The ratio of the areas of two similar triangles is equal to the square of the ratio of their corresponding sides
⇒ \(\\frac { \left( 3K \right) ^{ 2 } }{ \left( 7K \right) ^{ 2 } } =\frac { { 9K }^{ 2 } }{ { 49K }^{ 2 } } =\frac { ar\left( \triangle AED \right) }{ ar\left( \triangle ABC \right) } =\frac { 9 }{ 49 } \)
Let ar(∆AED) = 9p
and ar(∆ABC) = 49p
ar(BCDE) = ar (∆ABC) – ar (∆ADE)
= 49p – 9p = 40p
∴ \(\frac { ar\left( BCDE \right) }{ ar\left( \triangle ABC \right) } =\frac { 40p }{ 49p } \)
∴ ar (BCDE) : ar(AABC) = 40 : 49
Question 28. In the given figure, DE || BC and AD : DB = 7 : 5, find \frac { ar\left( \triangle DEF \right) }{ ar\left( \triangle CFB \right) } [/latex] (2017OD)
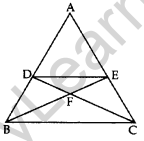
Solution: Given: In ∆ABC, DE || BC and AD : DB = 7 : 5.
To find: \(\frac { ar\left( \triangle DEF \right) }{ ar\left( \triangle CFB \right) } \) = ?
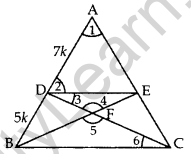
Proof: Let AD = 7k
and BD = 5k then
AB = 7k + 5k = 12k
In ∆ADE and ∆ABC,
∠1 = ∠1 …(Common
∠2 = ∠ABC … [Corresponding angles
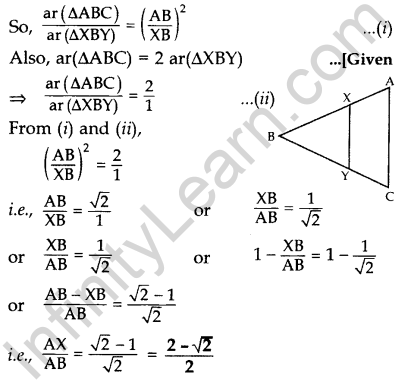
Question 29. In the given figure, the line segment XY is parallel to the side AC of ∆ABC and it divides the triangle into two parts of equal areas. Find the ratio \(\frac{\mathbf{A} \mathbf{X}}{\mathbf{A B}}\). (2017OD)
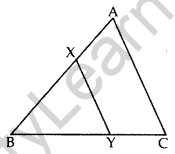
Solution: We have XY || AC … [Given
So, ∠BXY = ∠A and ∠BYX = ∠C …[Corresponding angles
∴ ∆ABC ~ ∆XBY …[AA similarity criterion
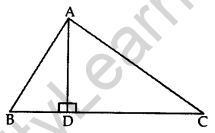
Question 30. In the given figure, AD ⊥ BC and BD = \(\frac{1}{3}\)CD. Prove that 2AC2 = 2AB2 + BC2. (2012)
Solution:
BC = BD + DC = BD + 3BD = 4BD
∴ \(\frac{\mathrm{BC}}{4}\) = BD
In rt. ∆ADB, AD2 = AB2 – BD2 ….(ii)
In rt. ∆ADC, AD2 = AC2 – CD2 …(iii)
From (ii) and (iii), we get
AC2 – CD2 = AB2 – BD2
AC2 = AB2 – BD2 + CD2

∴ 2AC2 = 2AB2 + BC2 (Hence proved)
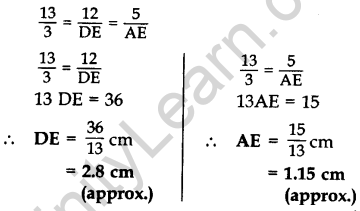
Question 31. In the given figure, ∆ABC is right-angled at C and DE ⊥ AB. Prove that ∆ABC ~ ∆ADE and hence find the lengths of AE and DE. (2012, 2017D)

Solution:
Given: ∆ABC is rt. ∠ed at C and DE ⊥ AB.
AD = 3 cm, DC = 2 cm, BC = 12 cm
To prove:
(i) ∆ABC ~ ∆ADE; (ii) AE = ? and DE = ?
Proof. (i) In ∆ABC and ∆ADE,
∠ACB = ∠AED … [Each 90°
∠BAC = ∠DAE …(Common .
∴ ∆ABC ~ ∆ADE …[AA Similarity Criterion
(ii) ∴ \(\frac{A B}{A D}=\frac{B C}{D E}=\frac{A C}{A E}\) … [side are proportional \(\frac{A B}{3}=\frac{12}{D E}=\frac{3+2}{A E}\)…..[In rt. ∆ACB, … AB2 = AC2 + BC2 (By Pythagoras’ theorem)
= (5)2 + (12)2 = 169
∴ AB = 13 cm