Section A
1.Simplify the following equation and identify whether this equation is quadratic or not:
(x – 2) (x + 2)=12.
3. Find the distance between the points A(8, – 2) and B(3, – 6).
4.Find the area of a sector of a circle when the radius of the circle is 21 cm and angle of the sector is 60°.
(Take = 22/7)
Section B
5.Find the roots of the following quadratic equation by using the method of factorization:
6X2 – 13x -5 = 0.
6. How many terms of the AP 9, 17, 25, …. must be taken to give a sum of 636?
7. The length of a tangent from a point A, at a distance 5 cm from the centre of the circle is 4 cm. Find the radius of the circle.
8. A bag contains lemon flavoured candies only. Malini takes out one candy without looking into the bag. What is the probability that she takes out
(i) an orange flavoured candy? (ii) a lemon flavoured candy?
9. A paper is in form of a rectangle ABCD in which AB = 20 cm and BC = 14 cm. A semi-circular portion with BC as diameter is cut off. Find the area of the remaining part. (Take = 22/7)
10. Two cubes each of side 10 cm are joined end to end. Find the surface area of the resulting rectangular shaped solid.
Section C
11. Solve for x: (1/x-2)+(2/x-1)=6/x (x not equal to 0,1,2)
12. The sum of the 4th and 8th terms of an AP is 24 and the sum of the 6th and 10th terms is 44. Find the first three terms of the AP.
13. Determine the value of p for which the quadratic equation px2 – 24x + 16 = 0 has equal roots.
14.Draw a circle of radius 6 cm. From a point 10 cm away from its centre, construct the pair of tangents to the circle and measure their lengths.
15. One card is drawn from a well shuffled deck of 52 cards. Find the probability of getting
(i) a king of red colour (ii) a face card (iii) the queen of diamonds
16. A lot consists of 220 ball pens out of which 22 are defective. Sunita will buy a pen if it is good but will not buy if it is defective. The shopkeeper draws one pen at random and gives it to her. What is the probability that
(i) she will buy it? (ii) she will not buy it?
17. Find the area of the triangle whose vertices are (1, – 1), (- 4, 6) and (3, – 5).
18.Find the value of x for which the distance between the points P(2, – 3) and Q(x, 5) is 10 units.
19.From a circular sheet of 10 cm radius, a disc of radius 3 cm is cut out and the portion of the sheet left behind is painted at the rate of ? 0.35 per cm2. Find the cost of painting the sheet. (Take n = -~)
20.The radii of the ends of a frustum of a right circular cone are 5 cm and 8 cm and its lateral height
(slant height) is 5 cm. Find the volume of the frustum. (Take = 22/7)
21.The perimeter of a rectangular piece of land is 130 m and its area is 1000 m2. Determine its dimensions.
22.A sum of ? 700 is to be used to give seven cash prizes to students of a school for their overall academic performance. If each prize is ? 20 less than its preceding prize, find the value of each of the prizes. What value is reflected by giving cash prizes to students?
23.Two tangents PQ and PR are drawn from an external point to a circle with centre O. Prove that QORP is a cyclic quadrilateral.
24.Two concentric circles are of radii 5 cm and 3 cm. Find the length of the chord of the larger circle which touches the smaller circle.
25.The tangent at any point of a circle is perpendicular to the radius through the point of contact. Prove it.
26.A ladder is placed against a wall of a house such that its upper end is touching the top of the wall. The foot of the ladder is 8 m away from the foot of the wall and the ladder is making an angle of 30° with the level of the ground. Determine the height of the wall. (Take = 1.732)
27.A vertical tower stands on the plane ground and is surmounted by a flagstaff of height 5 m. From a point on the ground, the angle of elevation of the bottom of the flagstaff is 45° and that of the top of the flagstaff is 60°. Find the height of the tower. (Take = 1.732)
28.Prove that the points A(- 3, 0), B(l, – 3) and C(4, 1) are the vertices of an isosceles right-angled triangle.
29.From each corner of a square of side 4 cm, a quadrant of a circle of radius 1 cm is cut and also a circle of diameter 2 cm is cut as shown in fig. Find the area of the remaining portion of the square.
(Take = 22/7)

30.A solid is in the form of a right circular cylinder mounted on a solid hemisphere of radius 14 cm. The radius of the base of the cylindrical part is 14 cm and the vertical height of the complete solid is 28 cm. Find the volume of the solid. (Take = 22/7)
31.A heap of rice is in the form of a cone of diameter 9 m and height 3.5 m. Find the volume of the rice. How much canvas cloth is required to just cover the heap? (Take = 22/7)
Answers
Section A
1.Simplify the following equation and identify whether this equation is quadratic or not:
(x – 2) (x + 2)=12.
Ans.

2.Write the AP with given first term a and common difference d for the following: a = 17,
d = – 6.
Ans.

3. Find the distance between the points A(8, – 2) and B(3, – 6).
Ans.
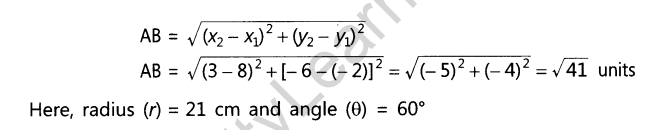
4.Find the area of a sector of a circle when the radius of the circle is 21 cm and angle of the sector is 60°.
(Take = 22/7)
Ans.
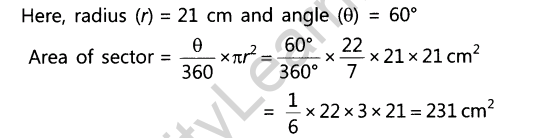
Section B
5.Find the roots of the following quadratic equation by using the method of factorization:
6X2 – 13x -5 = 0.
Ans.
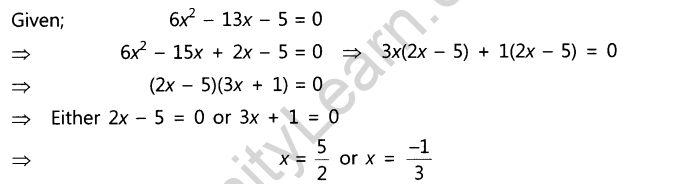
6. How many terms of the AP 9, 17, 25, …. must be taken to give a sum of 636?
Ans.

7. The length of a tangent from a point A, at a distance 5 cm from the centre of the circle is 4 cm. Find the radius of the circle.
Ans.
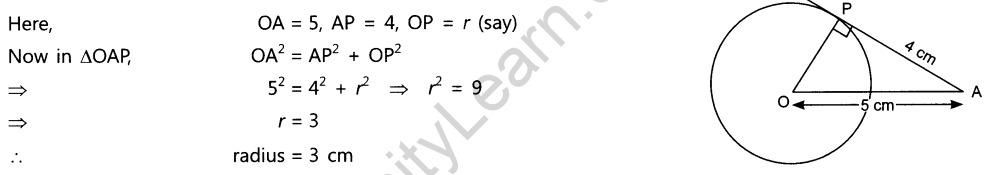
8. A bag contains lemon flavoured candies only. Malini takes out one candy without looking into the bag. What is the probability that she takes out
(i) an orange flavoured candy? (ii) a lemon flavoured candy?
Ans.
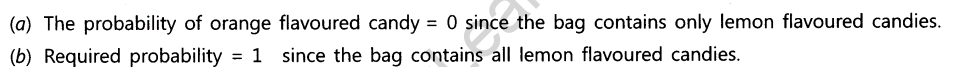
9. A paper is in form of a rectangle ABCD in which AB = 20 cm and BC = 14 cm. A semi-circular portion with BC as diameter is cut off. Find the area of the remaining part. (Take = 22/7)
Ans.

10. Two cubes each of side 10 cm are joined end to end. Find the surface area of the resulting rectangular shaped solid.
Ans.

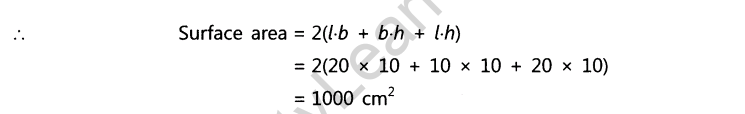
Section C
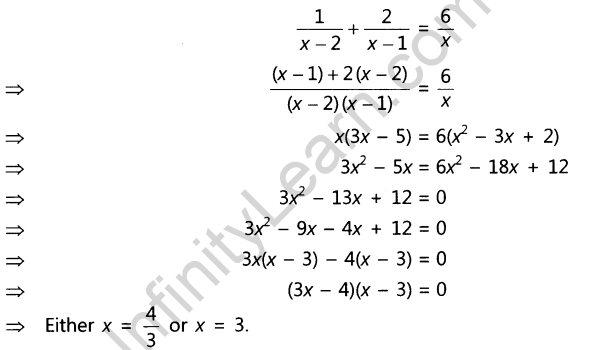
11. Solve for x: (1/x-2)+(2/x-1)=6/x (x not equal to 0,1,2)
Ans.
12. The sum of the 4th and 8th terms of an AP is 24 and the sum of the 6th and 10th terms is 44. Find the first three terms of the AP.
Ans.
13. Determine the value of p for which the quadratic equation px2 – 24x + 16 = 0 has equal roots.
Ans.
14.Draw a circle of radius 6 cm. From a point 10 cm away from its centre, construct the pair of tangents to the circle and measure their lengths.
Ans.
15. One card is drawn from a well shuffled deck of 52 cards. Find the probability of getting
(i) a king of red colour (ii) a face card (iii) the queen of diamonds
Ans.
16. A lot consists of 220 ball pens out of which 22 are defective. Sunita will buy a pen if it is good but will not buy if it is defective. The shopkeeper draws one pen at random and gives it to her. What is the probability that
(i) she will buy it? (ii) she will not buy it?
Ans.
17. Find the area of the triangle whose vertices are (1, – 1), (- 4, 6) and (3, – 5).
Ans.
18.Find the value of x for which the distance between the points P(2, – 3) and Q(x, 5) is 10 units.
Ans.
19.From a circular sheet of 10 cm radius, a disc of radius 3 cm is cut out and the portion of the sheet left behind is painted at the rate of ? 0.35 per cm2. Find the cost of painting the sheet. (Take n = 22/7)
Ans.
20.The radii of the ends of a frustum of a right circular cone are 5 cm and 8 cm and its lateral height (slant height) is 5 cm. Find the volume of the frustum. (Take = 22/7)
Ans.
21.The perimeter of a rectangular piece of land is 130 m and its area is 1000 m2. Determine its dimensions.
Ans.
22.A sum of ? 700 is to be used to give seven cash prizes to students of a school for their overall academic performance. If each prize is ? 20 less than its preceding prize, find the value of each of the prizes. What value is reflected by giving cash prizes to students?
Ans.
23.Two tangents PQ and PR are drawn from an external point to a circle with centre O. Prove that QORP is a cyclic quadrilateral.
Ans.
24.Two concentric circles are of radii 5 cm and 3 cm. Find the length of the chord of the larger circle which touches the smaller circle.
Ans.
25.The tangent at any point of a circle is perpendicular to the radius through the point of contact. Prove it.
Ans.
26.A ladder is placed against a wall of a house such that its upper end is touching the top of the wall. The foot of the ladder is 8 m away from the foot of the wall and the ladder is making an angle of 30° with the level of the ground. Determine the height of the wall. (Take = 1.732)
Ans.
27.A vertical tower stands on the plane ground and is surmounted by a flagstaff of height 5 m. From a point on the ground, the angle of elevation of the bottom of the flagstaff is 45° and that of the top of the flagstaff is 60°. Find the height of the tower. (Take = 1.732)
Ans.
28.Prove that the points A(- 3, 0), B(l, – 3) and C(4, 1) are the vertices of an isosceles right-angled triangle.
Ans.
29.From each corner of a square of side 4 cm, a quadrant of a circle of radius 1 cm is cut and also a circle of diameter 2 cm is cut as shown in fig. Find the area of the remaining portion of the square.
(Take = 22/7)

Ans.
30.A solid is in the form of a right circular cylinder mounted on a solid hemisphere of radius 14 cm. The radius of the base of the cylindrical part is 14 cm and the vertical height of the complete solid is 28 cm. Find the volume of the solid. (Take = 22/7)
Ans.
31.A heap of rice is in the form of a cone of diameter 9 m and height 3.5 m. Find the volume of the rice. How much canvas cloth is required to just cover the heap? (Take = 22/7)
Ans.





