Table of Contents
Area Formulae
Area formulas are mathematical expressions used to calculate the amount of space enclosed by a two-dimensional shape or surface. These formulas are fundamental in geometry and find application in various fields, such as engineering, architecture, and physics. Here, we will discuss some commonly used area formulas for different geometric shapes:
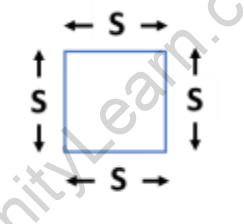
Area Formulae Of Square
The area of a square is found by multiplying the length of one side by itself: Area = side x side or A = s2, where “s” represents the length of a side.
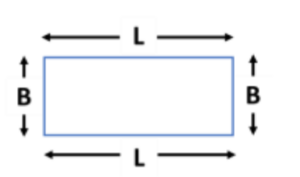
Area Formulae Of Rectangle
The area of a rectangle is given by the product of its length and width: Area = length x breadth or A = l x b.
Area Formulae Of Triangle
The area of a triangle can be calculated using different formulas based on the given information. For example:
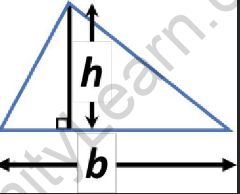
If the base and height of the triangle are known, the area is given by: Area = (1/2) x base x height or A = (1/2) x b x h.
If the lengths of all three sides are known, the area can be determined using Heron’s formula.
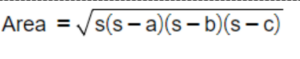
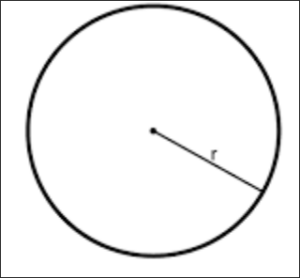
Area Formulae Of Circle
The area of a circle is calculated using the formula: Area = π x radius2 or A = π x r2, where “π” is a mathematical constant approximately equal to 3.14159 and “r” represents the radius of the circle.
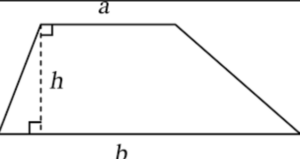
Area Formulae Of Trapezoid
The area of a trapezoid is given by: Area = (1/2) x (base1 + base2) x height or A = (1/2) x (b1 + b2) x h, where “b1” and “b2” are the lengths of the parallel bases, and “h” is the height.
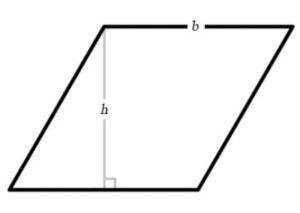
Area Formulae Of Parallelogram
The area of a parallelogram is equal to the product of the base and the corresponding height: Area = base x height or A = b x h.
Area Formulae Of Rhombus
The area of a rhombus can be found by multiplying the lengths of its diagonals and dividing by 2: Area = (diagonal1 x diagonal2) / 2 or A = (d1 x d2) / 2.

Area Formulae Of Regular Polygon
For a regular polygon with “n” sides and side length “s,” the area is given by: Area = (n x s2) / (4 x tan(π/n)).
It is essential to remember that these area formulas provide an approximate measure of the enclosed space and are applicable under specific conditions. They serve as useful tools for solving geometric problems, determining surface areas, and understanding spatial relationships in various contexts.
Solved Example on Area Formulae
Example1. Finding the area of a rectangle
Given: Length = 10 cm, Width = 5 cm
To find: Area of the rectangle
Solution:
The formula for the area of a rectangle is: Area = Length × Width
Substituting the given values, we have:
Area = 10 cm × 5 cm
Area = 50 cm²
Therefore, the area of the rectangle is 50 square centimeters.
Example 2. Finding the area of a triangle
Given: Base = 8 cm, Height = 12 cm
To find: Area of the triangle
Solution:
The formula for the area of a triangle is: Area = 0.5 × Base × Height
Substituting the given values, we have:
Area = 0.5 × 8 cm × 12 cm
Area = 48 cm²
Therefore, the area of the triangle is 48 square centimeters.
Example 3. Finding the area of a circle
Given: Radius = 5 cm
To find: Area of the circle
Solution:
The formula for the area of a circle is: Area = π × Radius²
Substituting the given values, we have:
Area = π × (5 cm)²
Area = 3.14 × 25 cm²
Area ≈ 78.5 cm² (rounded to one decimal place)
Therefore, the area of the circle is approximately 78.5 square centimeters.
Frequently Asked Questions on Area Formulae
What is surface area of a shape?
Surface area refers to the total area of all the outer faces of a three-dimensional shape. For example, the surface area of a rectangular box would include the area of all six sides: top, bottom, front, back, and two sides.
What is a formula of area of square?
The formula for the area of a square is A = side length (s) × side length (s), which can also be written as A = s^2. This means you multiply one side length by itself to find the area.
What is the formula of area?
The formula for area varies depending on the specific shape you're working with. It's essential to use the correct formula for the given shape to calculate its area.
What is another formula for area?
There are different formulas for the area of various shapes. For example: Rectangle: Area (A) = length × width Triangle: Area (A) = 1/2 × base × height Circle: Area (A) = π (pi) × radius (r) × radius (r) or A = πr^2 Each shape has its unique formula based on its properties.
What is area in physics?
In physics, area represents the extent of a two-dimensional surface or space. It plays a crucial role in various physical calculations and equations, such as calculating the flux of a vector field through a surface or determining the moment of inertia of an object. The concept of area is fundamental for understanding and solving many physical problems.









