Perimeter of Triangle Formula
What is the Perimeter of a triangle?
The perimeter of a triangle is a measure of the total length of its three sides. It is an essential concept in geometry that helps quantify the boundary or outer edge of a triangle. The perimeter is often used to determine the distance around the triangle or to compare the sizes of different triangles.
Consider a triangle with sides of lengths ‘a’, ‘b’, and ‘c’.
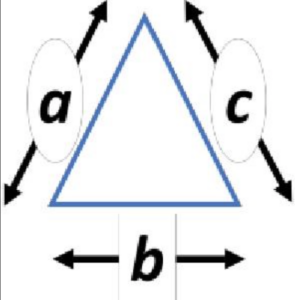
Thus, the perimeter of the triangle = a + b + c.
The formula for the perimeter of a triangle can be modified according to the type of triangle:
- Equilateral Triangle: In an equilateral triangle, all three sides are of equal length. To find the perimeter, multiply the length of one side by 3.
Perimeter = 3 x side length
- Isosceles Triangle: In an isosceles triangle, two sides are of equal length, while the third side is different. To find the perimeter, add the length of the two equal sides and the length of the different side.
Perimeter = 2 x equal side length + different side length
- Scalene Triangle: In a scalene triangle, all three sides have different lengths. To find the perimeter, simply add the lengths of all three sides.
Perimeter = side 1 length + side 2 length + side 3 length
The units of measurement used for the sides will determine the units of the perimeter. For example, if the sides are measured in centimeters, the perimeter will be in centimeters.
The perimeter of a triangle is a fundamental concept that helps in various practical applications. It is used in construction, architecture, and engineering to determine the required lengths of materials for framing and boundaries. Additionally, the perimeter is essential in solving problems related to the comparison of triangle sizes, calculating fencing requirements, or determining the distance around a triangular region on a map or in real-life scenarios.
Solved Example on Perimeter of Triangle
Example 1. A triangle has two sides of lengths 12 meters and 15 meters, and the third side is 9 meters. Calculate the perimeter of the triangle.
Solution:
To find the perimeter, we add the lengths of all three sides.
Perimeter = 12 meters + 15 meters + 9 meters
Perimeter = 36 meters
Therefore, the perimeter of the triangle is 36 meters.
Example 2. An equilateral triangle has a side length of 6 inches. Calculate its perimeter.
Solution:
Since it is an equilateral triangle, all three sides have the same length. To find the perimeter, we multiply the length of one side by 3.
Perimeter = 3 * 6 inches
Perimeter = 18 inches
Therefore, the perimeter of the equilateral triangle is 18 inches.
Example 3. In a triangle ABC, the coordinates of its vertices are A(3, 1), B(7, 4), and C(5, 8). Find the perimeter of the triangle.
Solution:
To find the perimeter of the triangle, we need to calculate the lengths of all three sides using the distance formula and then add them together.
Given:
Coordinates of A: A(3, 1)
Coordinates of B: B(7, 4)
Coordinates of C: C(5, 8)
Using the distance formula, we can find the lengths of the sides:
Side AB:
AB = √((x2 – x1)2 + (y2 – y1)2)
= √((7 – 3)2 + (4 – 1)2)
= √(42 + 32)
= √(16 + 9)
= √25
= 5
Side BC:
BC = √((x2 – x1)2 + (y2 – y1)2)
= √((5 – 7)2 + (8 – 4)2)
= √((-2)2 + 42)
= √(4 + 16)
= √20
= 2√5
Side AC:
AC = √((x2 – x1)2 + (y2 – y1)2)
= √((5 – 3)2 + (8 – 1)2)
= √(22 + 72)
= √(4 + 49)
= √53
Now, we can find the perimeter by adding the lengths of all three sides:
Perimeter = AB + BC + AC
= 5 + 2√5 + √53
Therefore, the perimeter of triangle ABC is 5 + 2√5 + √53.
Frequently Asked Questions on Perimeter of Triangle:
- What is the perimeter of a triangle?
Answer: The perimeter of a triangle is the total length of its three sides. It represents the distance around the triangle.
- How do you find the perimeter of a triangle?
Answer: To find the perimeter of a triangle, add the lengths of all three sides together. If the triangle is equilateral, you can multiply the length of one side by 3.
- Can the perimeter of a triangle be negative?
Answer: No, the perimeter of a triangle cannot be negative. It is always a positive value or zero.
- Can the perimeter of a triangle be decimal?
Answer: Yes, the perimeter of a triangle can be a decimal value. It depends on the lengths of the sides and the units of measurement used.
- Can two triangles have the same perimeter but different shapes?
Answer: Yes, two triangles can have the same perimeter but different shapes. The perimeter only depends on the lengths of the sides, not the shape or angles of the triangle.
- Can the perimeter of a triangle be used to calculate its area?
Answer: No, the perimeter of a triangle alone cannot be used to calculate its area. The area of a triangle requires the lengths of the sides as well as the height or base length.
- How is the perimeter of a triangle related to the concept of congruence?
Answer: In geometry, two triangles are congruent if they have the same shape and size. If two triangles are congruent, their perimeters will be equal.
- Is the perimeter of a triangle affected by the orientation of the triangle?
Answer: No, the perimeter of a triangle is not affected by its orientation. The lengths of the sides remain the same regardless of the orientation of the triangle.





