Table of Contents
Introduction to Right Triangle Formula
A triangle is a closed figure or shape with 3 sides, 3 angles, and 3 vertices, and for right triangle formulas, the properties have to be more specific. If any one of the angles of a triangle is a right angle (measuring 90º), the triangle is called a right-angled triangle or simply, a right triangle. Right triangle formulas would help you solve various calculations related to the perimeter, area, etc of the right triangle.
Right Triangle
A right triangle is the one in which the measure of any one of the interior angles is 90 degrees. It is to be noted here that since the sum of interior angles in a triangle is 180 degrees, only 1 of the 3 angles can be a right angle. If the other two angles are equal, that is 45 degrees each, the triangle is called an isosceles right-angled triangle. However, if the other two angles are unequal, it is a scalene right-angled triangle.
The most common application of right-angled triangles can be found in trigonometry. In fact, the relation between its angles and sides forms the basis for trigonometry.
What Are the Right Triangle Formulas?
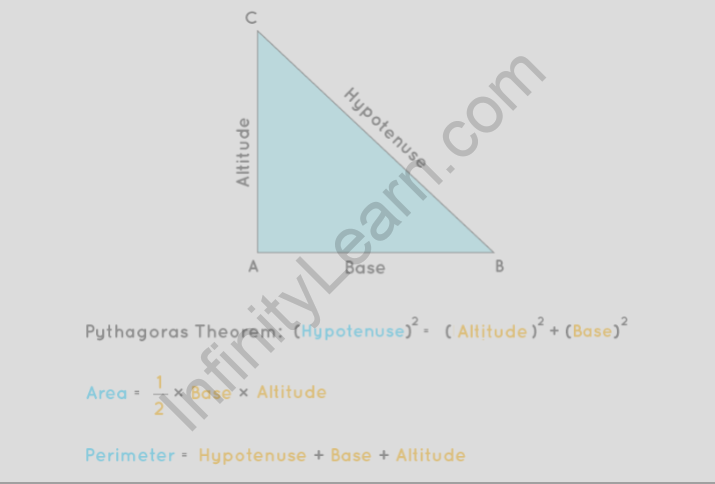
A right-angled triangle is one which has one of its interior angles measuring 90 degrees. Right-angled triangle formulas are used to calculate the perimeter, area, height, etc of a right triangle using its three sides.
Right-angled Triangle Formula
Different formulas associated with the right triangle are:
- Pythagoras Theorem – Formula
The Pythagoras theorem definition shows the relation among the three sides of a right triangle. The square of the hypotenuse is equal to the sum of the square of the other two sides.
(Hypotenuse)2 = (Perpendicular)2 + (Base)2
- Area of a right triangle formula
The formula to calculate the area of a right triangle formula is given as:
Area = ½ × Base × Height = 1/2 × b × h
where height,h is equal to the length of the perpendicular side of the triangle.
- The perimeter of a right triangle formula
The formula to calculate the area of a right triangle formula is given as:
Perimeter = a + b + c
where a, b, and c are the three sides of the triangle.
Solved Examples on Right Triangle Formula
Example 1: The length of the base and perpendicular of a right-angled triangle is 6 in and 8 in respectively. Find Length of its hypotenuse, The perimeter of the triangle, Area of the triangle.
Solution:
To find:
Given: length of base = 6 in, length of perpendicular = 8 in
- i) Using Pythogoras’ theorem,
(Hypotenuse)2 = (Base)2 + (Perpendicular)2
(Hypotenuse)2 = 62 + 82 = 100
Hypotenuse = √100 = 10 in
- ii) Using the perimeter of a right triangle formula,
Perimeter = Sum of all sides
Perimeter = 6 + 8 + 10 = 24 in
iii) Using the area of triangle formula,
Area = (1/2) × b × h
= (1/2) × 6 × 8
= 24 in2
Example 2: The height and hypotenuse of a right-angled triangle measure 12 in and 13 in respectively. Find its area.
Solution:
To find: Area of a right-angled triangle
Given: Height = 12 in, Hypotenuse = 13 in
Using Pythagoras’ theorem,
(13)2 = (Base)2 + (12)2
(Base)2 = (13)2 – (12)2 = 25
Base = √25 = 5 in
Using the Area of a triangle formula,
Area = (1/2) × b × h
Area = (1/2) × 5 × 12
Area = 30 in2
Example 3: Determine the area of a right-angled triangle whose perimeter is 30 units, height is 12 units, and the hypotenuse is 13 units
Solution:
To find: Area of a right-angled triangle
Given: perimeter = 30 units, hypotenuse = 13 units, height = 12 units
We know that perimeter = base + hypotenuse + height
30 units = 13 + 12 + base
Therefore, base = 30 – 25 = 5 units
Area = 1/2bh = 1/2(5×12) = 30 sq units.
Topics Related to Triangle
Area of Triangles with 3 Sides
Volume of a Triangular Prism Formula
Isosceles Triangle Perimeter Formula
Frequently Asked Questions on Right Triangle Formula
What is Right Triangle Formula in Geometry?
In geometry, the right triangle formulas are formulas of the right triangle that are used to calculate the perimeter, area, height, etc of the triangle using three of its sides - base, height, and hypotenuse. Pythagoras Theorem - Formula: (Hypotenuse)2 = (Perpendicular)2 + (Base)2 Area of a right triangle formula: Area = 1/2 × Base × Height Perimeter of a right triangle formula = Sum of lengths of 3 sides.
What are the Applications of Right Triangle Formula?
There are numerous applications of the right triangle in real life, the most common is its use in the branch of trigonometry as the relation between its angles and sides form the basis for trigonometry. It is further utilized in the construction and engineering field.
How to Calculate Area of Right Triangle Using Right Triangle Formula When its Perimeter, Height, and Base are Given?
In order, to calculate the area of the right triangle when its perimeter, height, and base are given, we will consider only two parameters - height and base. Step 1: Check for the given values. Step 2: Put the values of height h and base b in the area formula, (1/2)bh
How to Find the Height of a Right Triangle Formula?
How to Find the Height of a Right Triangle Formula?
What is a right-angled triangle?
If any one of the angles of a triangle is a right angle (measuring 90º), the triangle is called a right-angled triangle or simply, a right triangle.
What is the 3 4 5 rule right triangle?
The 3:4:5 triangle is the best way I know to determine with absolutely certainty that an angle is 90 degrees. This rule says that if one side of a triangle measures 3 and the adjacent side measures 4, then the diagonal between those two points must measure 5 in order for it to be a right triangle.
What is the Pythagorean theorem?
The Pythagorean theorem is a fundamental formula in right triangles. It states that in a right triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides.