Kinematics Formulas 

Kinematics formulas serve as essential mathematical tools in physics, elucidating the motion and positional attributes of objects. These equations establish connections between displacement, velocity, and acceleration, enabling comprehensive analysis of motion characteristics such as speed, time intervals, and trajectories. By leveraging these formulas, we can effectively quantify both geometric and dynamic features of moving objects, thereby enhancing our grasp of the fundamental principles governing physical phenomena.

Kinematics Formulas

Kinematics formulas are essential physics equations used to describe the motion of objects. They link key motion variables such as displacement, velocity, acceleration, and time, allowing us to analyze and predict the behavior of moving objects. These formulas are crucial in fields like physics, engineering, and robotics, providing the tools to understand and control various types of motion.

Kinematical Equations

The kinematic equations delineate object motion in terms of displacement, velocity, acceleration, and time. Rooted in foundational principles of calculus, these equations are universally applicable to objects experiencing either constant or uniformly accelerated motion.

The fundamental kinematic equations are articulated as follows:

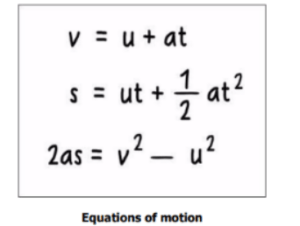
Here, ‘u’ is the initial velocity, ‘v’ is the final velocity, ‘a’ is the acceleration, ‘t’ is the time,

and ’s’ is the distance or displacement of the body.

The kinematic equations are primarily used for linear or straight-line motion. They assume constant acceleration, uniform motion, and no external forces acting on the object.

Application of Kinematics Formulas

Kinematics formulas find extensive applications across diverse fields. In physics, they play a crucial role in analyzing and predicting object motion, whether it’s tracking the path of a projectile, mapping planetary movements, or studying particle behavior in accelerators. Engineering and robotics rely heavily on these formulas to design and control mechanical systems and robots. In sports, kinematics formulas help analyze athletes’ movements, measure metrics like speed and acceleration, and refine techniques for optimal performance. Moreover, they are pivotal in computer graphics, animation, and virtual reality, enabling realistic simulations of motion and the creation of immersive virtual environments.

Solved Examples on Kinematics Formulas

Example 1: A car starts from rest and accelerates uniformly at a rate of 3 m/s². How far does it travel in 5 seconds?

Solution:

Given:

Initial velocity (u) = 0 m/s

Acceleration (a) = 3 m/s²

Time (t) = 5 s

We need to find the displacement (s).

Using Equation 2: s = ut + (1/2)at²

s = (0)(5) + (1/2)(3)(5²)

s = 0 + (1/2)(3)(25)

s = 0 + 37.5

s = 37.5 m

Hence, the car covers a distance of 37.5 meters within a span of 5 seconds.

Example 2: A ball is thrown vertically upwards with an initial velocity of 20 m/s. How high does it go before coming back down?

Solution:

Given:

Initial velocity (u) = 20 m/s

Acceleration due to gravity (g) = -9.8 m/s² (negative since it acts downwards)

We need to find the maximum height reached, which is the displacement at the highest point (where the velocity becomes zero).

Using Equation 3: v² = u² + 2as

At the highest point, the final velocity (v) is 0 m/s.

0² = (20)² + 2(-9.8)s

0 = 400 – 19.6s

19.6s = 400

s = 400 / 19.6

s≈ 20.41 m

Hence the ball reaches a maximum height of approx 20.41 meters.

Example 3: A cyclist begins from rest and accelerates uniformly at 2 m/s² for 10 seconds. Subsequently, the cyclist maintains a constant velocity for the next 20 seconds. Finally, the cyclist decelerates uniformly at 3 m/s² until coming to a stop. Calculate the total displacement of the cyclist.

Solution:

Phase 1: Acceleration

Initial velocity (u) = 0 m/s

Acceleration (a) = 2 m/s²

Time (t) = 10 s

Using Equation 2: s = ut + (1/2)at²

s1 = (0)(10) + (1/2)(2)(10²)

s1 = 0 + 100

s1 = 100 m

Phase 2: Constant Velocity

Velocity (v) = 20 m/s (since the cyclist maintains constant velocity)

Time (t) = 20 s

Using Equation 4: s = v x t

s2 = 20 m/s x 20

s2 = 400 m

Phase 3: Deceleration

Final velocity (v) = 0 m/s

Acceleration (a) = -3 m/s² (negative sign indicates deceleration)

Time (t) = ?

Using Equation 1: v = u + at

0 = 20 + (-3)t

3t = 20

t = 20/3 ≈ 6.67 s

Using Equation 2: s = ut + (1/2)at²

s3 = (20)(6.67) + (1/2)(-3)(6.67)²

s3 = 133.4 – 66.7

s3 = 66.7 m

Total displacement = s1 + s2 + s3

Total displacement = 100 + 400 + 66.7

Total displacement = 566.7 m

What are the main 3 kinematical equations?

The three main kinematic equations are fundamental tools in physics for describing the motion of objects. They relate an object's displacement, initial velocity, final velocity, acceleration, and time elapsed. These equations are derived from basic principles of calculus and are crucial for analyzing various types of motion, including uniformly accelerated motion and motion with constant velocity.

What is kinematics first equation?

First equation of kinematics is v= u + at where 𝑢 is its initial velocity and 𝑎 is its acceleration.

Who is considered the father of kinematics?

Sir Isaac Newton is often regarded as the father of kinematics for his foundational contributions to the study of motion and mechanics, particularly through his laws of motion and universal gravitation.

What role do kinematic equations play in the study of motion?

Kinematic equations provide a mathematical framework for describing and predicting the motion of objects, linking variables such as displacement, velocity, acceleration, and time.

What are the standard units used in kinematics?

Kinematics involves various quantities related to motion, each with its own specific units. The units commonly associated with kinematics include: Position: The unit of position is typically measured in meters (m), representing the distance from a reference point. Velocity: Velocity is the rate of change of position and is measured in meters per second (m/s). Acceleration: Acceleration represents the rate of change of velocity and is measured in meters per second squared (m/s²). Time: Time is a fundamental quantity in kinematics and is measured in seconds (s). These units are used to quantify and describe the motion of objects in kinematics.

What is the use of kinematics?

Kinematics is used to study and analyze the motion of objects without considering the forces involved. It helps in understanding the concepts of position, velocity, acceleration, and time in relation to the motion of objects. Kinematics is widely applied in fields such as physics, engineering, robotics, and animation to predict and describe the movement of objects, design mechanical systems, simulate motion, and optimize performance. It forms the foundation for understanding and analyzing various types of motion, providing valuable insights into the behavior of objects in motion.

What does v stand for in motion?

In the context of motion, v typically represents the symbol for velocity. It is measured in units of distance per unit time, such as meters per second (m/s) or kilometers per hour (km/h). The direction of velocity is typically indicated using a positive or negative sign, or by specifying a specific angle or direction.

What is free fall formula?

The formula for free fall describes the motion of an object falling freely under the influence of gravity, assuming no air resistance. In free fall, the only force acting on the object is the force of gravity. The formula for free fall involves the variables of time (t) and acceleration due to gravity (g). The general formula for free fall is: h = (1/2)gt² Where: h is the height or vertical displacement of the object, g is the acceleration due to gravity (approximately 9.8 m/s² on Earth), t is the time elapsed. It is derived from the second kinematical equation: s = ut + (1/2)at²